Linear Algebra Example Problems - Subspace Example #3

preview_player
Показать описание


We work with a subset of vectors from the vector space R2. We show that this subset of vectors is NOT a subspace of the vector space.

In general, given a subset of a vector space, one must show that all of the following are true:

1) Contains the zero vector, 2) Is closed under addition, and 3) Is closed under scalar multiplication.

If any of these fail, the subset is not a subspace. In this problem we show that both 1) and 3) are not true, and thus the subset of R2 is not a subspace of R2.

  1. Adam Panagos
  2. linear algebra subspace proofs
  3. subspace linear algebra
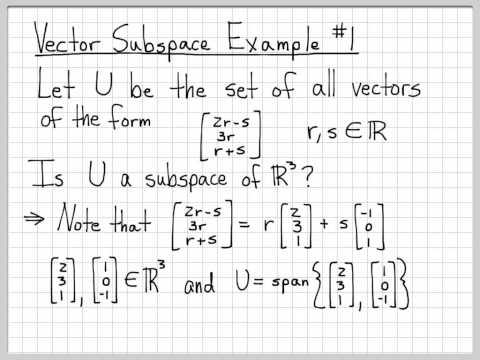
  4. subspace linear algebra examples
  5. linear subspace examples
  6. Linear Subspace
Рекомендации по теме
Linear Algebra Example 0:04:48

Linear Algebra Example Problems - Subspace Example #1

Linear Algebra Example 0:04:47

Linear Algebra Example Problems - Vector Space Basis Example #1

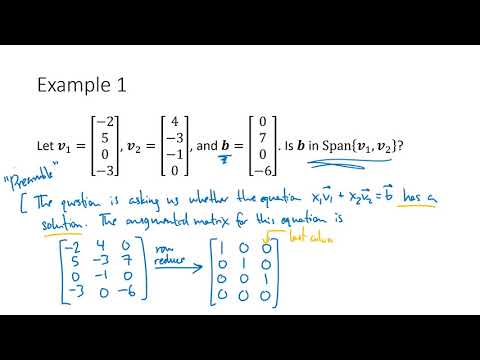
Linear Algebra Example: 0:12:47

Linear Algebra Example: Span Questions

Linear Algebra Example 0:03:53

Linear Algebra Example Problems - Linear Combination of Vectors #2

Linear Algebra Example 0:04:31

Linear Algebra Example Problems - Spanning Vectors #1

Linear Algebra Example 0:05:52

Linear Algebra Example Problems - Finding 'A' of a Linear Transformation #1

Linear Algebra 7 0:10:56

Linear Algebra 7 | Examples for Subspaces

linear algebra vector 0:30:16

linear algebra vector space (25 examples)

Normed vector space: 0:45:02

Normed vector space: definition and examples of vector and matrix norms

Linear Algebra Example 0:05:54

Linear Algebra Example Problems - Coordinate System Representation Example #1

Linear Algebra Example 0:04:42

Linear Algebra Example Problems - Linear Transformation Ax #1

Linear Algebra Example 0:05:02

Linear Algebra Example Problems - Vector Space Basis Example #2

Linear Algebra Example 0:08:41

Linear Algebra Example Problems - Change of Coordinates Matrix #1

Linear Algebra Example 0:04:41

Linear Algebra Example Problems - Diagonalizing a Matrix

Linear Algebra Example 0:07:40

Linear Algebra Example Problems - Finding 'A' of a Linear Transformation #2

Linear Algebra Example 0:05:20

Linear Algebra Example Problems - Onto Linear Transformations

Linear Algebra Example 0:07:14

Linear Algebra Example Problems - Subspace Example #2

Linear Algebra Example 0:04:13

Linear Algebra Example Problems - Eigenvalue Computation #1

Linear Algebra Example 0:03:37

Linear Algebra Example Problems - Linear Combination of Vectors #1

Linear Algebra Example 0:04:27

Linear Algebra Example Problems - Spanning Vectors #2

Linear Algebra Example 0:04:58

Linear Algebra Example Problems - Linearly Independent Vectors #1

Linear Algebra Example 0:06:14

Linear Algebra Example Problems - A Polynomial Subspace

Linear Algebra Example 0:09:10

Linear Algebra Example Problems - Subspace Example #4

Linear Algebra Example 0:05:46

Linear Algebra Example Problems - Subspace Example #3

Комментарии
Автор

simple and concise, by the way i thought that scalar C should be an element of the same set, after reading your comment it is clear, thank you.

DavidMOUSSONGA
Автор

Thank you so much!! I have a final tomorrow and this helps so much!

stephiediores
Автор

Your videos are so helpful. Thanks for taking the time to create these!

travisl
Автор

Very straight forward and easy to understand. Thank You!

adamali
Автор

Does U violate the 2nd rule as well in this problem? Can it be closed under addition?

adam.kruger
Автор

Sir thank you so much...sir can you upload the videos on INNER PRODUCT SPACE .concept...sir.

mathsgnr
Автор

but in example 2 (previous video) you didn't choose any value for the scalar C, couldn't you make C = 1 and it will satisfy the 3rd condition?

amermurshak
Автор

but [0 0] do have zero element and it is closed under addition and multiplication. Check the khan academy video they mentioned [0 0] is a subspace

PankajKumar-yggy
Автор

one question, why did you pick -1?
or is the c not defined by x > 1

schotterfresse