filmov
tv
Linear Algebra Example Problems - A Polynomial Subspace

Показать описание
The vector space P3 is the set of all at most 3rd order polynomials with the "normal" addition and scalar multiplication operators.
In this video we work with a subset of elements from P3 and formally show that it is a subspace. Since P3 is already a vector space, to show this subset is a subspace we only need to check 3 properties, not all 10 normal vector space properties. We work through each of these 3 properties to show the subset is indeed a subspace.
Linear Algebra Example Problems - Subspace Example #1
Linear Algebra Example Problems - Vector Space Basis Example #1
Linear Algebra Example: Span Questions
Linear Algebra Example Problems - Spanning Vectors #1
Linear Algebra Example Problems - Linear Combination of Vectors #2
Linear Algebra Example Problems - Finding 'A' of a Linear Transformation #1
Linear Algebra 7 | Examples for Subspaces
linear algebra vector space (25 examples)
Linear Algebra: number systems, overview of matrix math, 8-19-24
Linear Algebra Example Problems - Spanning Vectors #2
Linear Algebra Example Problems - Coordinate System Representation Example #1
Linear Algebra Example Problems - Diagonalizing a Matrix
Linear Transformations , Example 1, Part 1 of 2
Linear Algebra Example Problems - Change of Coordinates Matrix #1
Linear Algebra Example Problems - Linear Transformation Ax #1
Linear Algebra Example Problems - Linearly Independent Vectors #1
Linear Algebra Example Problems - Linear Combination of Vectors #1
Gilbert Strang: Linear Algebra vs Calculus
Linear Algebra Example Problems - Eigenvalue Computation #1
Linear Algebra Example Problems - Onto Linear Transformations
Linear Algebra Example Problems - Subspace Example #2
Linear Algebra Example Problems - Finding 'A' of a Linear Transformation #2
Linear Algebra Example Problems - Subspace Example #4
Linear Algebra Example Problems - A Polynomial Subspace
Комментарии
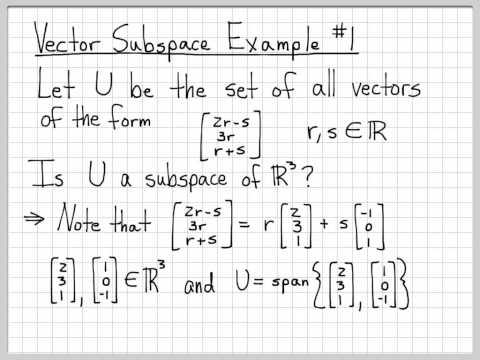 0:04:48
0:04:48
 0:04:47
0:04:47
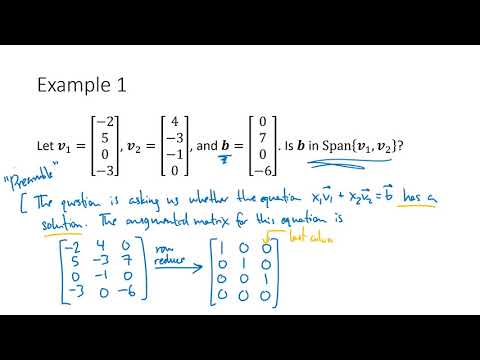 0:12:47
0:12:47
 0:04:31
0:04:31
 0:03:53
0:03:53
 0:05:52
0:05:52
 0:10:56
0:10:56
 0:30:16
0:30:16
 0:46:13
0:46:13
 0:04:27
0:04:27
 0:05:54
0:05:54
 0:04:41
0:04:41
 0:09:06
0:09:06
 0:08:41
0:08:41
 0:04:42
0:04:42
 0:04:58
0:04:58
 0:03:37
0:03:37
 0:02:14
0:02:14
 0:04:13
0:04:13
 0:05:20
0:05:20
 0:07:14
0:07:14
 0:07:40
0:07:40
 0:09:10
0:09:10
 0:06:14
0:06:14