Linear Algebra Example Problems - Onto Linear Transformations

Показать описание

An onto linear transformation can "reach" every element in its codomain. More specifically, consider the linear transformation T: Rn to Rm. The linear transformation T is onto if for each b in Rm, there exists an x in Rn such that T(x) = b.

When a linear transformation is described in term of a matrix it is easy to determine if the linear transformation is onto or not by checking the span of the columns of the matrix. If the columns span Rm, then the linear transformation is onto. If the columns do not span Rm, then the linear transformation is not onto.

This video works two different examples. One linear transformation is found to be onto while the other linear transformation is NOT onto.

Рекомендации по теме

Комментарии

You are the real OG. What a legend. Someone get this bro an Emmy award. You should become a world leader

andyhype

If columns all have pivots then LI one to one

If rows all have pivots then onto

mookitty

Simple explanation, thank you. my professor spent 45 minutes explaining onto and one to one by using theorems.

silverreyes

Thank you for your videos! You explain things so very clearly and simply.

sarahb

Just watched the other video on 'one-to-one'! Great videos!!

ryandavis

That means Linearly independent condition holds both one-one and one to though onto means it spans R3. Do we miss anything to know?
BTW You videos are extremely beautiful. Thank you for helping us

xavier.antony

Great video, it had everything I wanted to know

Owlberti

Hmm so for both Onto and 1to1 you check for Linear Independence. What is the difference between the two then?

Duxa_

Does this imply that if a linear transformation is 1-to-1, it is onto as well?

alexandroochoa

What is the difference on onto and one-to-one only spanning?

brsege

but spanning doesn't mean that it must be linearly independent

ekm

does not this make the check for one-to-one and onto the same

RizwanAhmad-ommd

But thats confusing. For one to one we check for linear independence and for onto we check for it again?? Then whats the difference between onto n one one ??

shadmankhan

I'm a bit confused. Onto and 1:1 have different meanings, but the exact same criterium to determine them?

anniamatthews

What is meant by ℝ^3, Does it show matrix has 3 rows? Isn't this ℝ symbol used for real numbers?

hammad

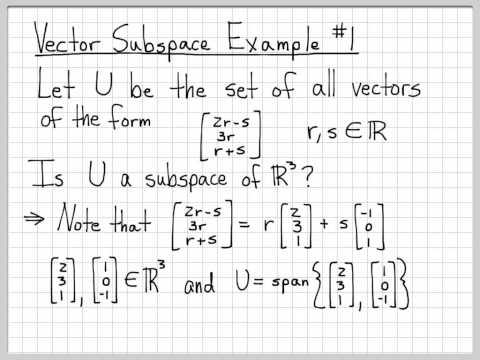
Linear Algebra Example Problems - Onto Linear Transformations

Linear Algebra Example Problems - Subspace Example #1

Linear Algebra Example Problems - Finding 'A' of a Linear Transformation #1

Linear Algebra Example Problems - Eigenvalue Computation #1

Gilbert Strang: Linear Algebra vs Calculus

Linear Algebra Example Problems - Solving Systems of Equations (1/3)

Linear Algebra Example Problems - Subspace Dimension #2 (Rank Theorem)

Linear Algebra Example Problems - Vector Space Basis Example #1

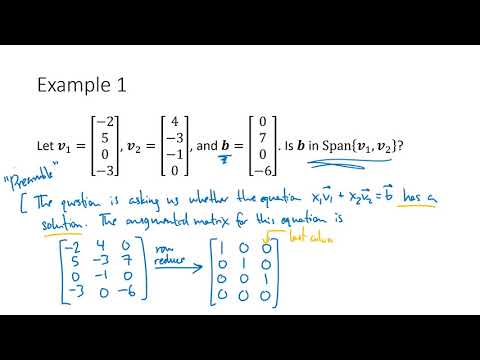
Linear Algebra Example: Span Questions

Linear Algebra: number systems, overview of matrix math, 8-19-24

Linear Algebra Example Problems - Matrix Multiplication #2

Linear Algebra Example Problems - Spanning Vectors #1

Matrix Determinant Properties Example #1 - Linear Algebra Example Problems -

Linear Algebra Example Problems - Linear Combination of Vectors #2

Linear Algebra Example Problems - Matrix Multiplication #1

Linear Algebra 7 | Examples for Subspaces

Linear Algebra Example Problems - Finding 'A' of a Linear Transformation #3

Linear Algebra Example Problems - Matrix Determinant Computation

Matrix Determinant Properties Example #2 - Linear Algebra Example Problems -

linear algebra vector space (25 examples)

Linear Algebra Example Problems - Subspace Example #4

Matrix Determinant Properties Example #3 - Linear Algebra Example Problems

Linear Algebra Example Problems - Coordinate System Representation Example #1

Linear Algebra Example Problems - Eigenvalue Computation #2

Linear Algebra Example Problems - Spanning Vectors #2