filmov
tv
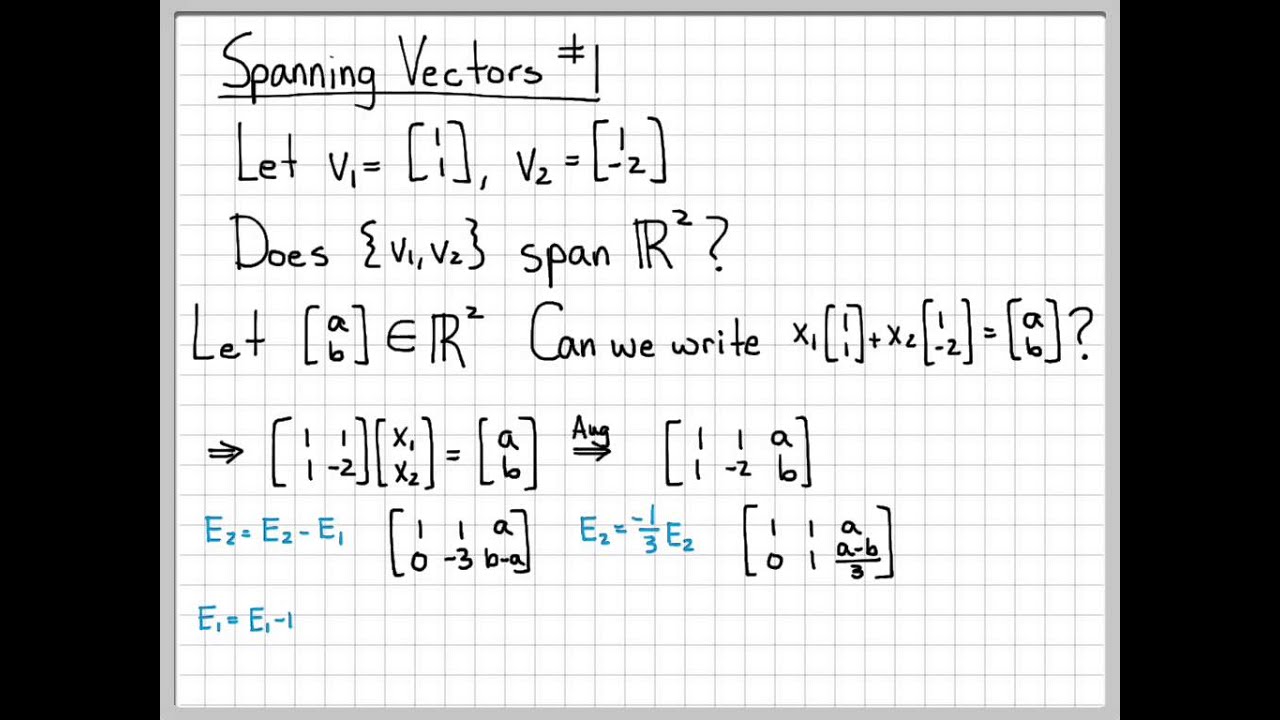
Linear Algebra Example Problems - Spanning Vectors #1
Показать описание
In this problem we work with the vectors v1 and v2 and determine if the set {v1, v2} spans R2.
If the set of vectors {v1,v2} spans R2, then ANY vector from R2 can be written as a linear combination of these vectors. To see if this is true, an arbitrary vector from R2 is selected an and an augmented matrix is constructed and solved.
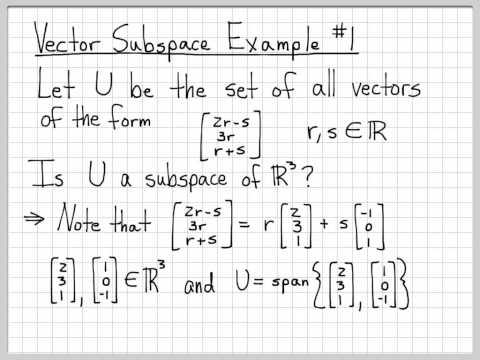
Linear Algebra Example Problems - Subspace Example #1
Linear Algebra Example Problems - Vector Space Basis Example #1
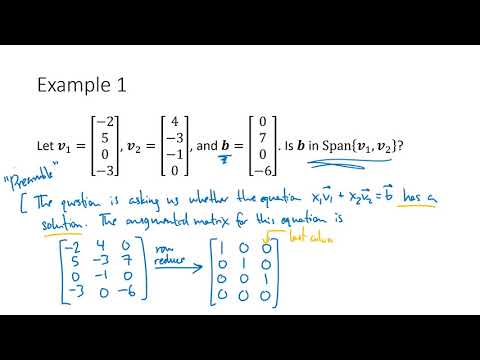
Linear Algebra Example: Span Questions
Linear Algebra Example Problems - Spanning Vectors #1
Linear Algebra Example Problems - Finding 'A' of a Linear Transformation #1
Linear Algebra Example Problems - Linear Combination of Vectors #2
Linear Algebra 7 | Examples for Subspaces
linear algebra vector space (25 examples)
Polynomials and Matrices | Linear Algebra | Today's School
Linear Algebra Example Problems - Coordinate System Representation Example #1
Linear Algebra Example Problems - Diagonalizing a Matrix
Linear Algebra Example Problems - Linear Transformation Ax #1
Linear Algebra Example Problems - Change of Coordinates Matrix #1
Linear Algebra Example Problems - Linearly Independent Vectors #1
Linear Algebra Example Problems - Subspace Example #2
Linear Transformations , Example 1, Part 1 of 2
Linear Algebra Example Problems - Eigenvalue Computation #1
Linear Algebra Example Problems - Spanning Vectors #2
Linear Algebra Example Problems - Finding 'A' of a Linear Transformation #2
Linear Algebra Example Problems - Linear Combination of Vectors #1
Linear Algebra Example Problems - Onto Linear Transformations
Linear Algebra Example Problems - Subspace Example #4
Linear Algebra Example Problems - A Polynomial Subspace
Linear Algebra Example Problems - Basis for an Eigenspace
Комментарии
 0:04:48
0:04:48
 0:04:47
0:04:47
 0:12:47
0:12:47
 0:04:31
0:04:31
 0:05:52
0:05:52
 0:03:53
0:03:53
 0:10:56
0:10:56
 0:30:16
0:30:16
 0:28:40
0:28:40
 0:05:54
0:05:54
 0:04:41
0:04:41
 0:04:42
0:04:42
 0:08:41
0:08:41
 0:04:58
0:04:58
 0:07:14
0:07:14
 0:09:06
0:09:06
 0:04:13
0:04:13
 0:04:27
0:04:27
 0:07:40
0:07:40
 0:03:37
0:03:37
 0:05:20
0:05:20
 0:09:10
0:09:10
 0:06:14
0:06:14
 0:07:48
0:07:48