Prove the Triangle Inequality (Real Number Case)

preview_player
Показать описание

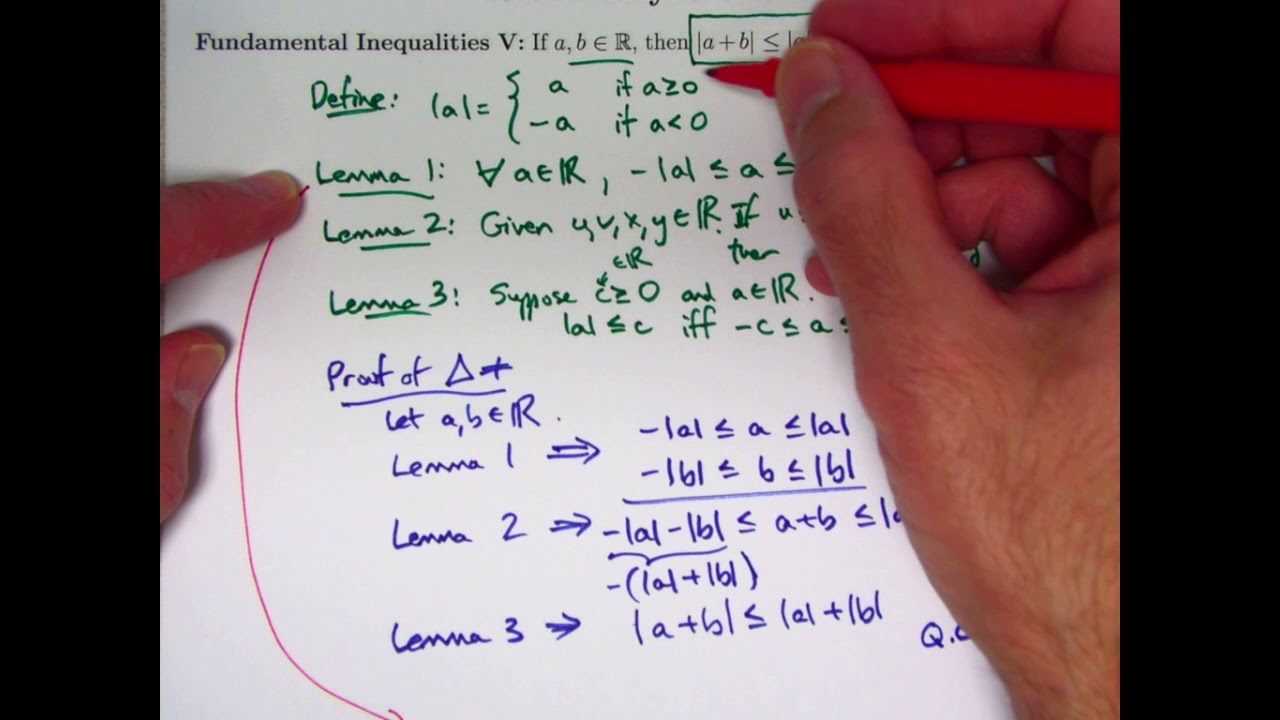
Real Analysis Proofs, Video #7 (Fundamental Inequalities V). Use the formula for the absolute value function and basic lemmas. It is best to structure the proof of the triangle inequality through the use of lemmas.

(0:00) Statement of the triangle inequality
(1:13) Define |x|, the absolute value of a real number, with a piecewise formula
(2:42) Lemma 1: -|a| is less than or equal to a is less than or equal to |a|
(3:34) Lemma 2: Inequalities are preserved by addition
(4:13) Lemma 3: |a| is less than or equal to c if and only if a is between -c and c
(5:22) Prove the triangle inequality using the lemmas
(9:16) Prove Lemma 1 using cases
(11:15) Prove Lemma 2
(12:24) Prove Lemma 3

AMAZON ASSOCIATE
As an Amazon Associate I earn from qualifying purchases.
  1. Bill Kinney
  2. real analysis
  3. triangle inequality
  4. triangle inequality theorem
  5. why is the triangle inequality theorem true
  6. why is the triangle inequality true
Рекомендации по теме
Proof: Triangle Inequality 0:05:30

Proof: Triangle Inequality Theorem | Real Analysis

Triangle Inequality for 0:03:08

Triangle Inequality for Real Numbers Proof

Proof: Reverse Triangle 0:08:53

Proof: Reverse Triangle Inequality Theorem | Real Analysis

Prove the Triangle 0:14:19

Prove the Triangle Inequality (Real Number Case)

Can You Prove 0:03:01

Can You Prove the Triangle Inequality?

Triangular inequality Proof 0:05:10

Triangular inequality Proof (easy method)

A quick proof 0:00:48

A quick proof of the triangle inequality

Triangle inequality 0:00:05

Triangle inequality

CALCULUS. Proof Triangle 0:07:27

CALCULUS. Proof Triangle Inequality (#5)

Proving the triangle 0:04:10

Proving the triangle inequality, math proofs, Learn to do mathematical proofs

Prove the Triangle 0:08:45

Prove the Triangle Inequality for Complex Numbers

triangular inequality 0:00:10

triangular inequality

Triangle Inequality Proof 0:06:02

Triangle Inequality Proof

Triangle Inequality - 0:09:46

Triangle Inequality - Simple Proof

Reverse Triangle Inequality 0:05:04

Reverse Triangle Inequality Proof

The Triangle Inequality 0:15:08

The Triangle Inequality

Idea of Proof 0:14:00

Idea of Proof of Triangle Inequality on R^n

Triangle Inequality Explained 0:00:31

Triangle Inequality Explained Simply

The Triangle Inequality 0:11:49

The Triangle Inequality Theorem and It's Proof

Triangle Inequality 0:13:08

Triangle Inequality

Triangle inequality theorem 0:05:52

Triangle inequality theorem | Perimeter, area, and volume | Geometry | Khan Academy

Triangular inequality theorem 0:06:07

Triangular inequality theorem proof || statement + Proof || urdu hindi lecture ||maths by Zahfran

Reverse Triangle Inequality 0:03:37

Reverse Triangle Inequality Absolute Value Proof

How to Prove 0:09:24

How to Prove the Triangle Inequality for Complex Numbers

Комментарии
Автор

Idk how people learned math without Youtube. Thanks!

ddiq
Автор

There is a trend among mathematics communicators to approach proofs or mathematics in general relying on the audience to fill in gaps of explanation (essentially adopting a blasé attitude about minor details). This is ok I guess for most things but when it comes to inequalities it’s best to not skip steps in explaining. For this reason I would say this video is the best.

alephnull
Автор

Thank you Professor, God bless you always!

DrTymish
Автор

Sir i didnt get the define thing in the starting
|5|=5 but |-5|=-5?? How?
|| is supposed to positify the no.s within it? Isn't it?

shiwangee
Автор

Im asking for your whatsapp line professor ... If you don't mind sir

slimthanos