filmov
tv
Solving a Nice Polynomial Equation
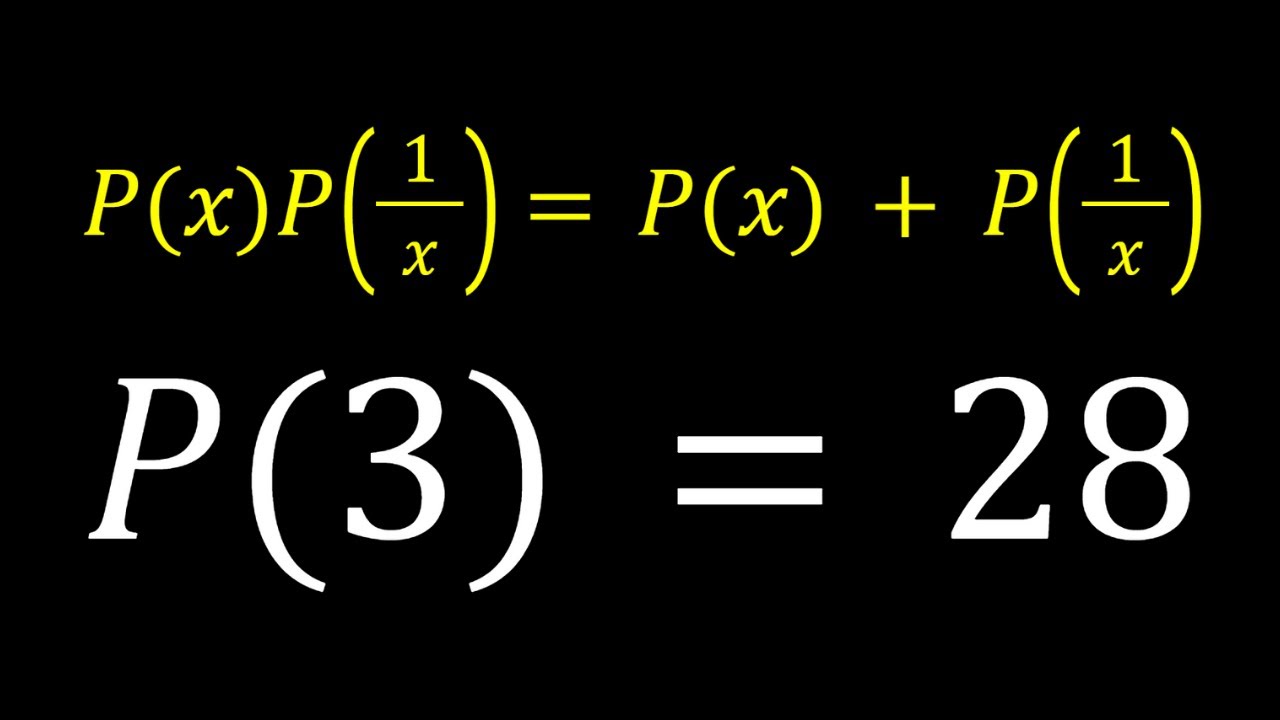
Показать описание
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #PolynomialEquations
PLAYLISTS 🎵 :
#ChallengingMathProblems #PolynomialEquations
PLAYLISTS 🎵 :
Solving a Nice Polynomial Equation
A Nice Polynomial Equation | Algebra
Solving a Nice Polynomial System in Two Ways
Solving A Nice Polynomial Equation
Solving A Nice Polynomial Equation
Solving a Nice Polynomial System in Two Ways
Solving a Nice Polynomial System in Two Ways
Solving A Nice Polynomial System | Algebra
A Nice Math Olympiad exponential equation Problem | #maths #matheolympiad | @ncertlearninglab
Solving A Nice Polynomial System | Two Ways
Solving Polynomial Equations By Factoring and Using Synthetic Division
Let's Solve A Nice Polynomial Equation | Math Olympiads
Let's Solve A Nice Polynomial System
A Nice Polynomial Equation from Croatia 🇭🇷
A Nice Polynomial System
A Nice Polynomial Equation | Math Olympiads
I Solved A Nice Polynomial System
A Nice Polynomial System
A Nice Polynomial Equation
A Nice Polynomial Equation | Cubic Formula?
A Nice Polynomial System
Solving A Nice Polynomial System by Math26039335
A Nice Polynomial Equation | Cubic Formula
A Nice Polynomial Equation from Croatia
Комментарии
 0:05:53
0:05:53
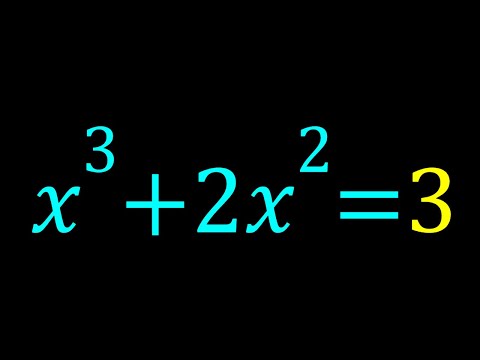 0:10:00
0:10:00
 0:09:03
0:09:03
 0:08:35
0:08:35
 0:08:20
0:08:20
 0:09:27
0:09:27
 0:08:38
0:08:38
 0:08:57
0:08:57
 0:12:29
0:12:29
 0:10:56
0:10:56
 0:14:19
0:14:19
 0:09:34
0:09:34
 0:10:27
0:10:27
 0:09:27
0:09:27
 0:08:13
0:08:13
 0:09:32
0:09:32
 0:06:23
0:06:23
 0:08:40
0:08:40
 0:00:51
0:00:51
 0:08:26
0:08:26
 0:08:19
0:08:19
 0:11:06
0:11:06
 0:10:56
0:10:56
 0:09:53
0:09:53