filmov
tv
Solving a Nice Polynomial System in Two Ways
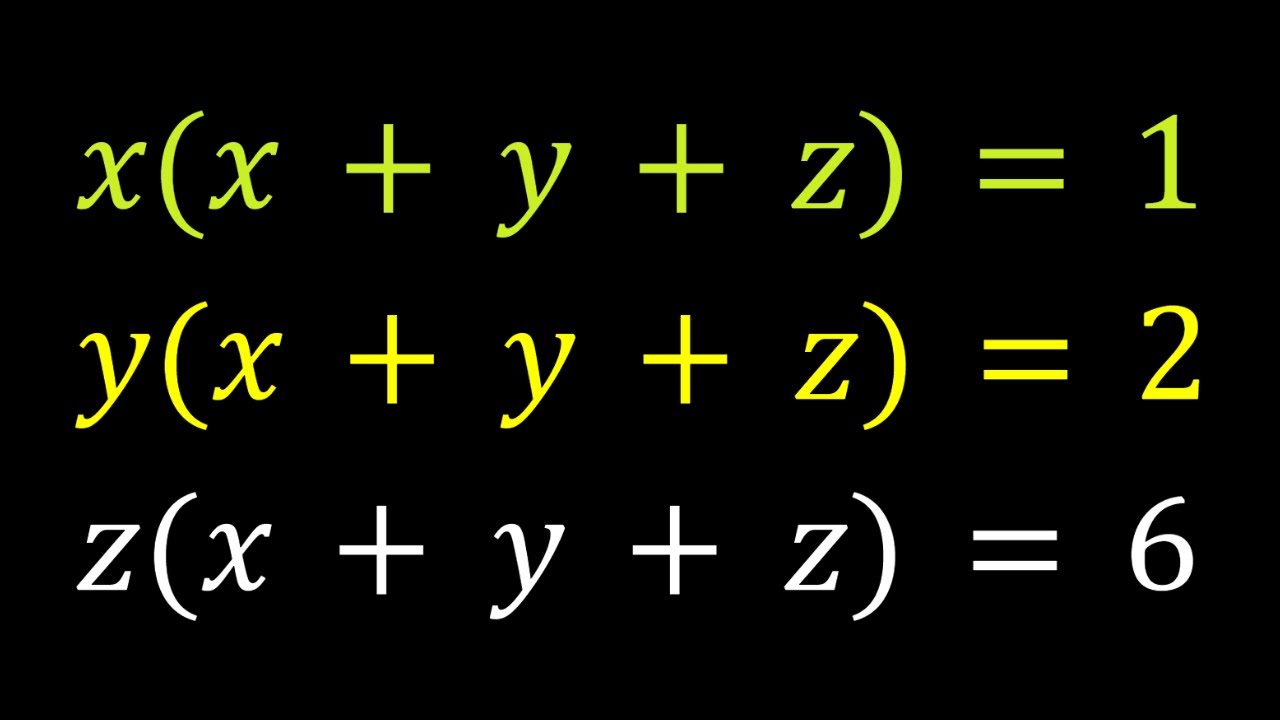
Показать описание
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #PolynomialSystems
PLAYLISTS 🎵 :
#ChallengingMathProblems #PolynomialSystems
PLAYLISTS 🎵 :
Solving a Nice Polynomial System in Two Ways
Solving a Nice Polynomial System in Two Ways
Solving a Nice Polynomial System in Two Ways
Solving A Nice Polynomial System | Algebra
A Nice Polynomial System #algebra
Solving A Nice Polynomial System | Two Ways
Let's Solve A Nice Polynomial System
A Nice Polynomial System
J.E.D.I. Training MATH for DIGITAL SAT - Day 6 of 7 (37 Digital SAT Math Problems EXPLAINED)
A Nice Polynomial System
I Solved A Nice Polynomial System
A Nice Polynomial System
Solving A Nice Polynomial System by Math26039335
Solving a Nice Polynomial Equation
A Nice Polynomial System by Stanley Rabinowitz
A Very Nice Polynomial System
A Nice Polynomial Equation | Algebra
Let's Solve A Nice Polynomial Equation | Math Olympiads
A Nice Polynomial System Solved in Three Ways
Solving A Real Polynomial System in Two Ways
Solving a Polynomial System of Equations
A Nice Polynomial System | Math Olympiads
An Interesting Polynomial System
Solving A Nice Polynomial Equation
Комментарии
 0:09:03
0:09:03
 0:08:38
0:08:38
 0:09:27
0:09:27
 0:08:57
0:08:57
 0:09:25
0:09:25
 0:10:56
0:10:56
 0:10:27
0:10:27
 0:08:40
0:08:40
 1:04:45
1:04:45
 0:08:13
0:08:13
 0:06:23
0:06:23
 0:08:19
0:08:19
 0:11:06
0:11:06
 0:05:53
0:05:53
 0:10:27
0:10:27
 0:10:31
0:10:31
 0:10:00
0:10:00
 0:10:49
0:10:49
 0:09:33
0:09:33
 0:10:59
0:10:59
 0:11:00
0:11:00
 0:07:10
0:07:10
 0:09:58
0:09:58
 0:08:35
0:08:35