filmov
tv
A Nice Functional Equation

Показать описание
🤩 Hello everyone, I'm very excited to bring you a new channel (SyberMath Shorts).
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #FunctionalEquations #Functions
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #FunctionalEquations #Functions
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
a nice functional equation
Solving A Nice Functional Equation
A Nice and Easy Functional Equation
Solving a Nice Functional Equation
Solving A Nice Functional Equation
A Nice Functional Equation
A Nice and Easy Functional Equation
a nice functional equation.
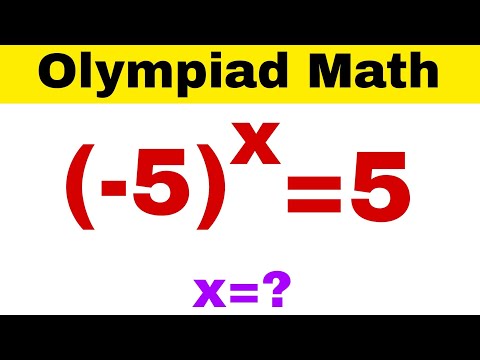
A Nice Exponential Olympiad Math Problem | Algebraic Equation
what a nice functional equation!
A nice functional equation from Romania
the greatest functional equation of all time.
Another Nice Functional Equation #algebra
A Nice And Easy Functional Equation
A Nice and Easy Functional Equation
a fun functional equation
A Nice Functional Equation #maths #matholympiad #education #functions #function
Solving f(x/y)=f(x)/f(y), A Nice Functional Equation
A Functional Equation from Samara Math Olympiads
A Very Nice Functional Equation on Reals
A Nice Homemade Functional Equation
A functional equation from my favorite book.
One of the coolest functional equations I have seen!
A fun functional equation!!
Комментарии
 0:11:25
0:11:25
 0:09:50
0:09:50
 0:05:07
0:05:07
 0:06:28
0:06:28
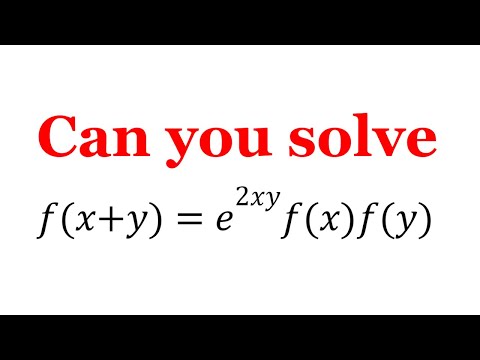 0:08:26
0:08:26
 0:08:45
0:08:45
 0:08:14
0:08:14
 0:18:42
0:18:42
 0:02:50
0:02:50
 0:10:10
0:10:10
 0:18:36
0:18:36
 0:07:48
0:07:48
 0:05:51
0:05:51
 0:10:51
0:10:51
 0:08:32
0:08:32
 0:09:20
0:09:20
 0:00:44
0:00:44
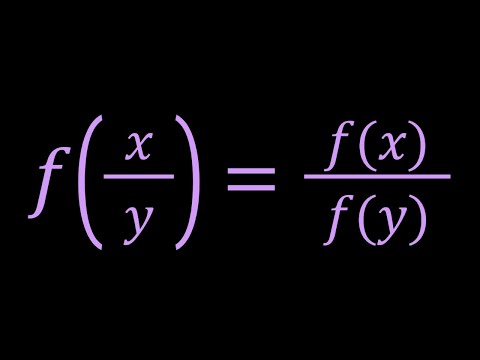 0:07:10
0:07:10
 0:08:47
0:08:47
 0:08:15
0:08:15
 0:09:22
0:09:22
 0:11:23
0:11:23
 0:15:34
0:15:34
 0:11:13
0:11:13