filmov
tv
what a nice functional equation!

Показать описание
🌟Support the channel🌟
🌟my other channels🌟
🌟My Links🌟
🌟How I make Thumbnails🌟
🌟Suggest a problem🌟
🌟my other channels🌟
🌟My Links🌟
🌟How I make Thumbnails🌟
🌟Suggest a problem🌟
a nice functional equation
Solving A Nice Functional Equation
what a nice functional equation!
A Nice and Easy Functional Equation
A Nice Functional Equation
Solving A Nice Functional Equation
a nice functional equation.
Solving a Nice Functional Equation
A Nice Algebra Exponential problem || maths olympiad questions || find value of x
the greatest functional equation of all time.
a fun functional equation
A nice functional equation from Romania
A Nice and Easy Functional Equation
Another Nice Functional Equation #algebra
A fun functional equation!!
a notorious functional equation.
A functional equation from my favorite book.
A Functional Equation from Samara Math Olympiads
A functional equation from @goodplacetostop2973
A Nice Functional Equation #maths #matholympiad #education #functions #function
One of the coolest functional equations I have seen!
A super nice Rational functional equation (Not real, or is it?😉)
A Very Nice Functional Equation on Reals
Solving f(x/y)=f(x)/f(y), A Nice Functional Equation
Комментарии
 0:11:25
0:11:25
 0:09:50
0:09:50
 0:10:10
0:10:10
 0:05:07
0:05:07
 0:08:45
0:08:45
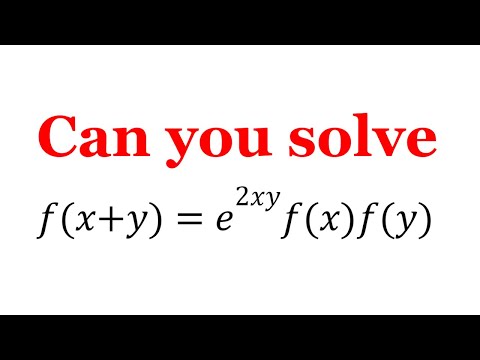 0:08:26
0:08:26
 0:18:42
0:18:42
 0:06:28
0:06:28
 0:04:12
0:04:12
 0:07:48
0:07:48
 0:09:20
0:09:20
 0:18:36
0:18:36
 0:08:14
0:08:14
 0:05:51
0:05:51
 0:11:13
0:11:13
 0:19:30
0:19:30
 0:11:23
0:11:23
 0:08:47
0:08:47
 0:08:18
0:08:18
 0:00:44
0:00:44
 0:15:34
0:15:34
 0:19:33
0:19:33
 0:08:15
0:08:15
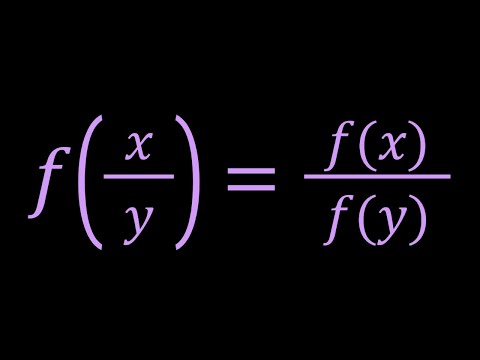 0:07:10
0:07:10