filmov
tv
Prove that (1 + 1/n)^n ﹤ 3 (ILIEKMATHPHYSICS)

Показать описание
In this video, we prove that for all positive integers n, (1 + 1/n)^n ﹤ 3. This is a useful component if you want to prove that the sequence (1 + 1/1)^1, (1 + 1/2)^2, (1 + 1/3)^3, ..., (1 + 1/n)^n, ... converges. In fact, this sequence converges to the value e = 2.718281828459.... This proof shows that this sequence is bounded above (by 3), but we did not show that the sequence is monotonically increasing. In fact, you can use a very similar argument (using Bernoulli's Inequality) to show that this sequence is monotonically increasing. By the Monotone Convergence Theorem, the sequence converges, and we may call the value it converges to by "e".
Notice that the method to prove (1 + 1/n)^n ﹤ 3 in this video can be extended to a stronger claim -- in the sense that you can also prove (1 + 1/n)^n ﹤ 2.75, or even (1 + 1/n)^n ﹤ 2.72.
Thanks and enjoy the video!
Notice that the method to prove (1 + 1/n)^n ﹤ 3 in this video can be extended to a stronger claim -- in the sense that you can also prove (1 + 1/n)^n ﹤ 2.75, or even (1 + 1/n)^n ﹤ 2.72.
Thanks and enjoy the video!
Prove that (1 + 1/n)^n ﹤ 3 (ILIEKMATHPHYSICS)
Proof: Supremum of {n/(n+1)} = 1 | Real Analysis
Prove lim (n→∞)[(1+1/n)^n]=e
Prove that Sum((-1)^n * (n choose r)) = 0
Proof that the Sequence (-1)^n Diverges using the Definition
Discrete Mathematics: Prove 1*1! + 2*2! + ... + n*n! = (n+1)! - 1
Sequences (Real Analysis) | SE#7 | Monotone convergence theorem | prove (1+1/n)^n is convergent
Induction Inequality Proof Example 1: Σ(k = 1 to n) 1/k² ≤ 2 - 1/n
Prove by Mathematical Induction. Sum = n/n+1
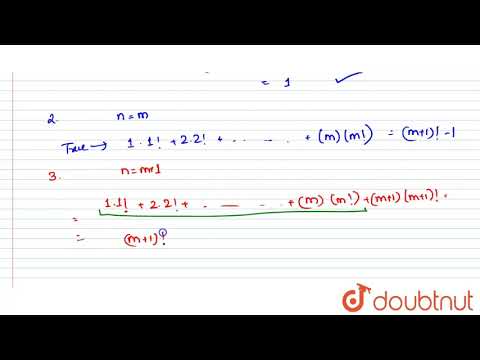
Prove by the principle of mathematical induction, that `1.1!+2.2!+3.3!+.....+(n.n!)=(n+1)!-1\'f...
Prove (1+x)^n is greater than or equal to 1+nx. Principle of Mathematical Induction
Every Student Should See This
PROVE THAT n CHOOSE r + n CHOOSE r-1=n+1 CHOOSE r {Let n and r be positive integers such n ≥ r} COMB...
If I did this in 1734 I'd be World Famous
Definition of the Limit of a Sequence | Real Analysis
Proof by Mathematical Induction - How to do a Mathematical Induction Proof ( Example 1 )
Prove that `1+1/4+1/9+1/(16)+....+1/n^2 lt2-1/n` for all `n gt2`, n`in`N
Prove Bernoulli's Inequality, (1 + x)^n ≥ 1 + nx (ILIEKMATHPHYSICS)
Proof of Binomial Coefficients to follow Pascals Triangle
The Harmonic Number Is Never An Integer When n Is BIGGER Than 1
Prove that 1/2 + 1/4 + 1/8 + ... + 1/2^(n - 1) is less than 1
ASTOUNDING: 1 + 2 + 3 + 4 + 5 + ... = -1/12
why does 1+1/2+1/3+... = infinity?
🐝 PROVE : 1(1)! +2(2)! +3(3)! +....n(n)! =(n+1) ! - 1
Комментарии
 0:12:08
0:12:08
 0:10:20
0:10:20
![Prove lim (n→∞)[(1+1/n)^n]=e](https://i.ytimg.com/vi/go0qeFDTGCo/hqdefault.jpg) 0:05:58
0:05:58
 0:02:13
0:02:13
 0:04:27
0:04:27
 0:10:36
0:10:36
 0:03:38
0:03:38
 0:13:09
0:13:09
 0:08:22
0:08:22
 0:02:26
0:02:26
 0:05:33
0:05:33
 0:00:58
0:00:58
 0:15:33
0:15:33
 0:03:57
0:03:57
 0:13:59
0:13:59
 0:07:32
0:07:32
 0:03:59
0:03:59
 0:04:42
0:04:42
 0:05:56
0:05:56
 0:21:18
0:21:18
 0:04:40
0:04:40
 0:07:50
0:07:50
 0:07:46
0:07:46
 0:01:01
0:01:01