filmov
tv
Induction Inequality Proof Example 1: Σ(k = 1 to n) 1/k² ≤ 2 - 1/n
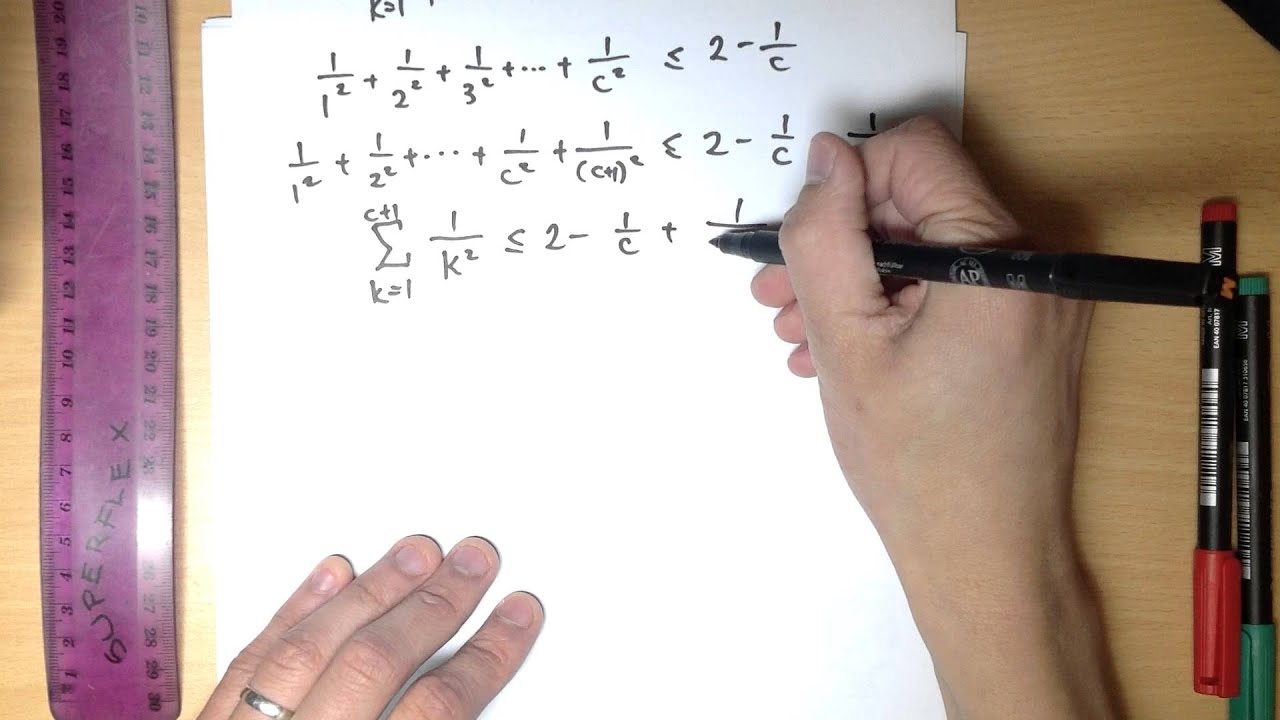
Показать описание
Induction proofs involving sigma notation look intimidating, but they are no more difficult than any of the other proofs that we've encountered!
A-Level Further Maths: A1-26 Proof by Induction: Inequality Example 1
Induction Inequality Proof Example 1: Σ(k = 1 to n) 1/k² ≤ 2 - 1/n
Induction with inequalities
Induction: Inequality Proofs
00b - Mathematical Induction Inequality
Inequality Mathematical Induction Proof: 2^n greater than n^2
Principle of Mathematical Induction Inequality Proof Video
Induction Proofs Involving Inequalities.
LC HL proof by induction - inequality proofs
💯 How to Find the Initial Value of an Inequality Proof by Math Induction Explained
Unlock the Secrets of Inequality Proof with Mathematical Induction 🧐🔍
Discrete Math - 5.1.2 Proof Using Mathematical Induction - Inequalities
Induction Inequality Proof
Example of Proof by Induction 4 - Hard Inequality
Proof by induction Inequality example 2 Leaving Cert Higher Level Maths
💯 The Inequality Proof with an Assumption by Math Induction Explained an Example
Proof by induction Inequality example 1 Leaving Cert Higher Level Maths
Mathematical induction with inequality
Induction Inequality Proof: 3^n is greater than or equal to 2n + 1
Proof by Mathematical Induction - How to do a Mathematical Induction Proof ( Example 1 )
Mathematical Induction Practice Problems
Induction Inequality Proof Example 7: 4^n ≥ 1+3n
proof by induction inequality example
Proof by Induction: Example with Inequality
Комментарии
 0:08:34
0:08:34
 0:13:09
0:13:09
 0:04:07
0:04:07
 0:14:30
0:14:30
 0:18:47
0:18:47
 0:09:20
0:09:20
 0:05:54
0:05:54
 0:06:34
0:06:34
 0:14:11
0:14:11
 0:09:28
0:09:28
 0:07:04
0:07:04
 0:09:53
0:09:53
 0:17:54
0:17:54
 0:06:03
0:06:03
 0:05:54
0:05:54
 0:06:43
0:06:43
 0:09:24
0:09:24
 0:12:53
0:12:53
 0:08:49
0:08:49
 0:07:32
0:07:32
 0:18:08
0:18:08
 0:09:39
0:09:39
 0:04:06
0:04:06
 0:08:43
0:08:43