filmov
tv
A Nice Factorial Equation | n!=2^n
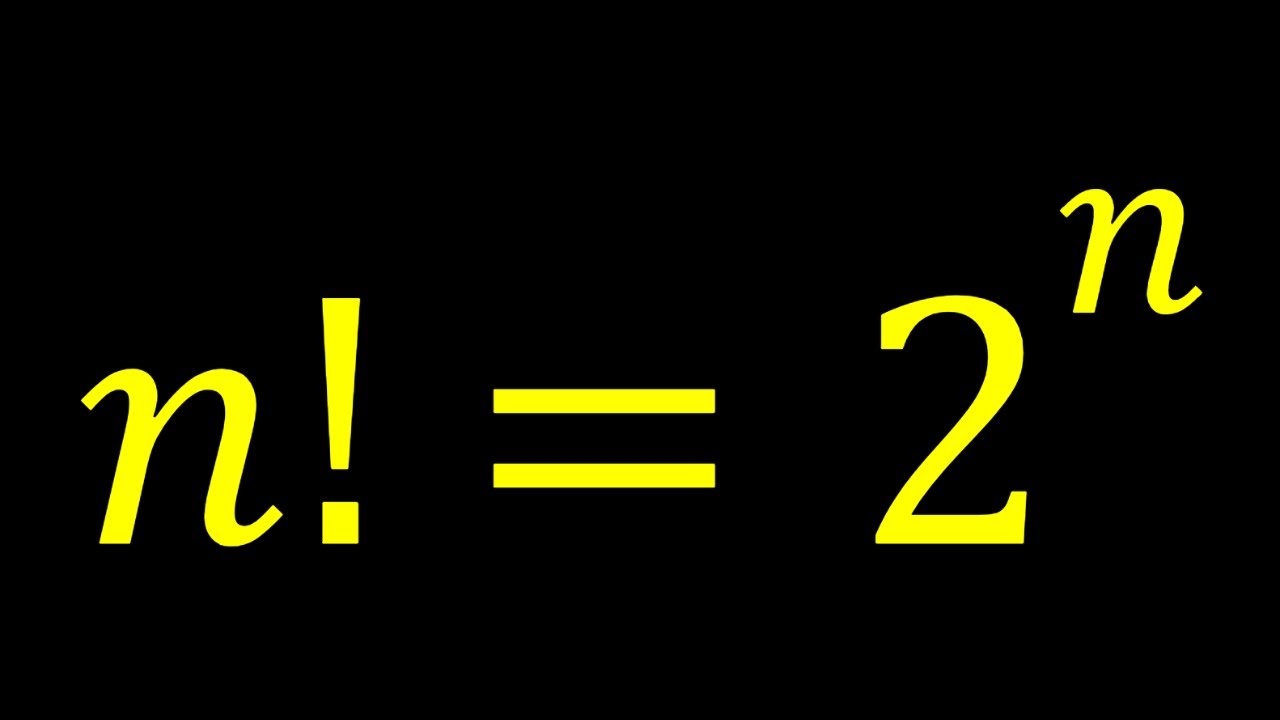
Показать описание
🤩 Hello everyone, I'm very excited to bring you a new channel (SyberMath Shorts)
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#NumberTheoryProblems #FactorialEquations #Factorials
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#NumberTheoryProblems #FactorialEquations #Factorials
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Solving A Nice Factorial Equation
A Nice Factorial Equation
A nice factorial equation: The math behind solving equations.
a nice factorial equation.
Can We Solve A Nice Factorial Equation
A Nice Math Factorial Problem • Evaluate #shorts #olympiad #mathematics #maths #matholympiad #tips
a nice factorial problem
Math Olympiad Preparation | A Nice Factorial Problem | Calculate Without Calculators...
DAY 03 | D & AOA | V SEM | BCA | ASYMPTOTIC NOTATIONS & BASIC EFFICIENCY CLASSES | L1
Let's Solve A Nice Factorial Equation
A Nice Factorial Equation | n!=2^n
A Nice Factorial Equation 🔥• X= ?
A Nice Factorial Equation #maths #matholympiad #education
Brazil | A nice factorial equation | math Olympiad
Norway - Math Olympiad Question | A Nice Factorial Problem
USA | A nice factorial equation | math Olympiad problem
A Nice Factorial Equation #shorts #mathstricks
A Nice And Perfect Factorial Equation
what is the value of X ? | A nice factorial equation to solve
A Beautiful Exponential Problem Olympiad Math || A Nice Factorial Equation
A Nice Factorial Equation | x!=6!7!
Factorials Explained!
Factorial Equation
How to Take the Factorial of Any Number
Комментарии
 0:03:19
0:03:19
 0:08:38
0:08:38
 0:10:54
0:10:54
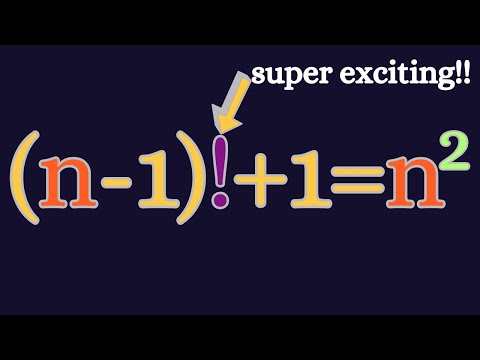 0:10:16
0:10:16
 0:09:31
0:09:31
 0:00:40
0:00:40
 0:00:37
0:00:37
 0:06:19
0:06:19
 0:26:54
0:26:54
 0:10:28
0:10:28
 0:09:32
0:09:32
 0:02:09
0:02:09
 0:00:37
0:00:37
 0:08:56
0:08:56
 0:02:35
0:02:35
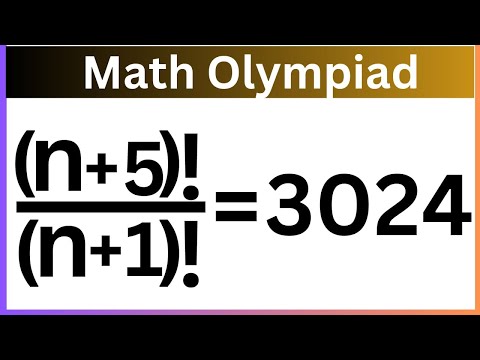 0:11:10
0:11:10
 0:01:00
0:01:00
 0:05:26
0:05:26
 0:03:37
0:03:37
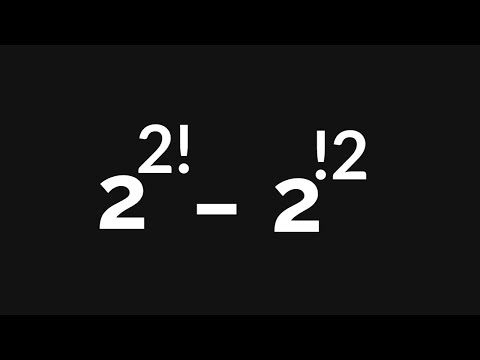 0:02:57
0:02:57
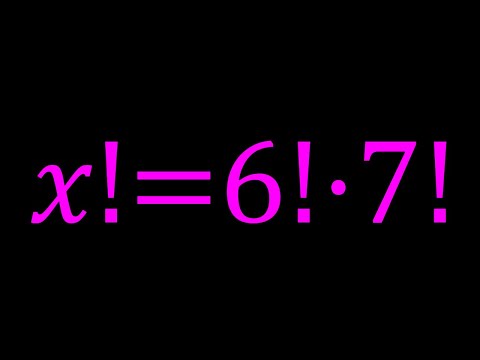 0:08:33
0:08:33
 0:11:17
0:11:17
 0:00:49
0:00:49
 0:26:31
0:26:31