filmov
tv
Solving the Schrodinger Equation in 3D | Angular Equation (Spherical Harmonics)

Показать описание
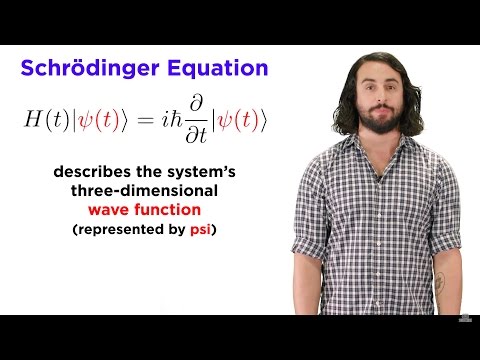
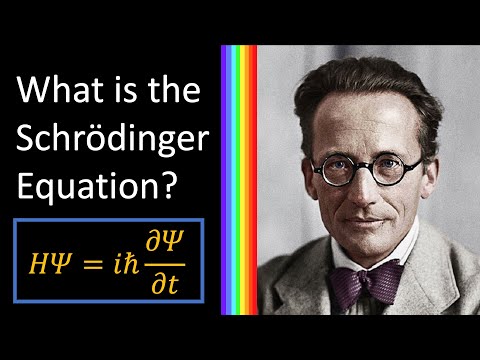
In this video I have talked about how we can solve the angular equation part of the Schrödinger equation. The solutions are spherical harmonics and based on the l (total angular momentum or azimuthal quantum number) and m (relating to z component of angular momentum called magnetic quantum number). This is the first step, actually the angular step towards finding the solutions of the Schrodinger equation for the Hydrogen atom.
The azimuthal quantum number, denoted by the symbol 'l,' describes the shape of an electron's orbital. It is also known as the angular momentum quantum number or the orbital quantum number. The value of 'l' can range from 0 to (n-1), where 'n' is the principal quantum number. The azimuthal quantum number determines the type of orbital an electron occupies:
l = 0 corresponds to an s-orbital
l = 1 corresponds to a p-orbital
l = 2 corresponds to a d-orbital
l = 3 corresponds to an f-orbital
The magnetic quantum number, represented by the symbol 'm_l,' describes the orientation of an electron's orbital in space. The values of 'm_l' range from -l to +l, including 0. For a given value of 'l,' there are (2l + 1) possible values of 'm_l.'
--------------------------------------------------------------------------------------------------------
In this series of videos I'm going to share some information about quantum physics based on the amazing book "Quantum Mechanics" by David J. Griffiths.
Quantum Physics playlist:
The azimuthal quantum number, denoted by the symbol 'l,' describes the shape of an electron's orbital. It is also known as the angular momentum quantum number or the orbital quantum number. The value of 'l' can range from 0 to (n-1), where 'n' is the principal quantum number. The azimuthal quantum number determines the type of orbital an electron occupies:
l = 0 corresponds to an s-orbital
l = 1 corresponds to a p-orbital
l = 2 corresponds to a d-orbital
l = 3 corresponds to an f-orbital
The magnetic quantum number, represented by the symbol 'm_l,' describes the orientation of an electron's orbital in space. The values of 'm_l' range from -l to +l, including 0. For a given value of 'l,' there are (2l + 1) possible values of 'm_l.'
--------------------------------------------------------------------------------------------------------
In this series of videos I'm going to share some information about quantum physics based on the amazing book "Quantum Mechanics" by David J. Griffiths.
Quantum Physics playlist:
Комментарии
 0:13:04
0:13:04
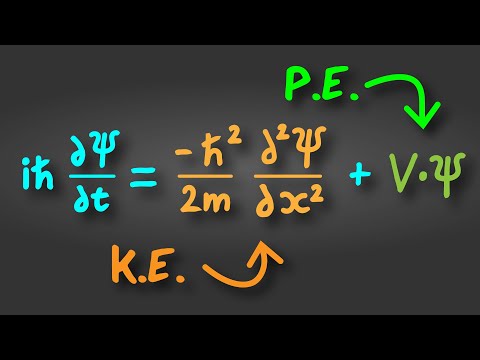 0:01:00
0:01:00
 0:16:35
0:16:35
 0:04:30
0:04:30
 0:10:19
0:10:19
 0:26:32
0:26:32
 0:49:30
0:49:30
 0:06:28
0:06:28
 0:08:45
0:08:45
 0:05:35
0:05:35
 0:12:19
0:12:19
 0:03:45
0:03:45
 0:02:38
0:02:38
 0:12:59
0:12:59
 1:27:34
1:27:34
 0:29:55
0:29:55
 0:33:23
0:33:23
 0:02:13
0:02:13
 0:13:02
0:13:02
 0:04:45
0:04:45
 0:20:59
0:20:59
 0:46:00
0:46:00
 0:14:08
0:14:08
 0:20:27
0:20:27