filmov
tv
A Nice Polynomial System #algebra
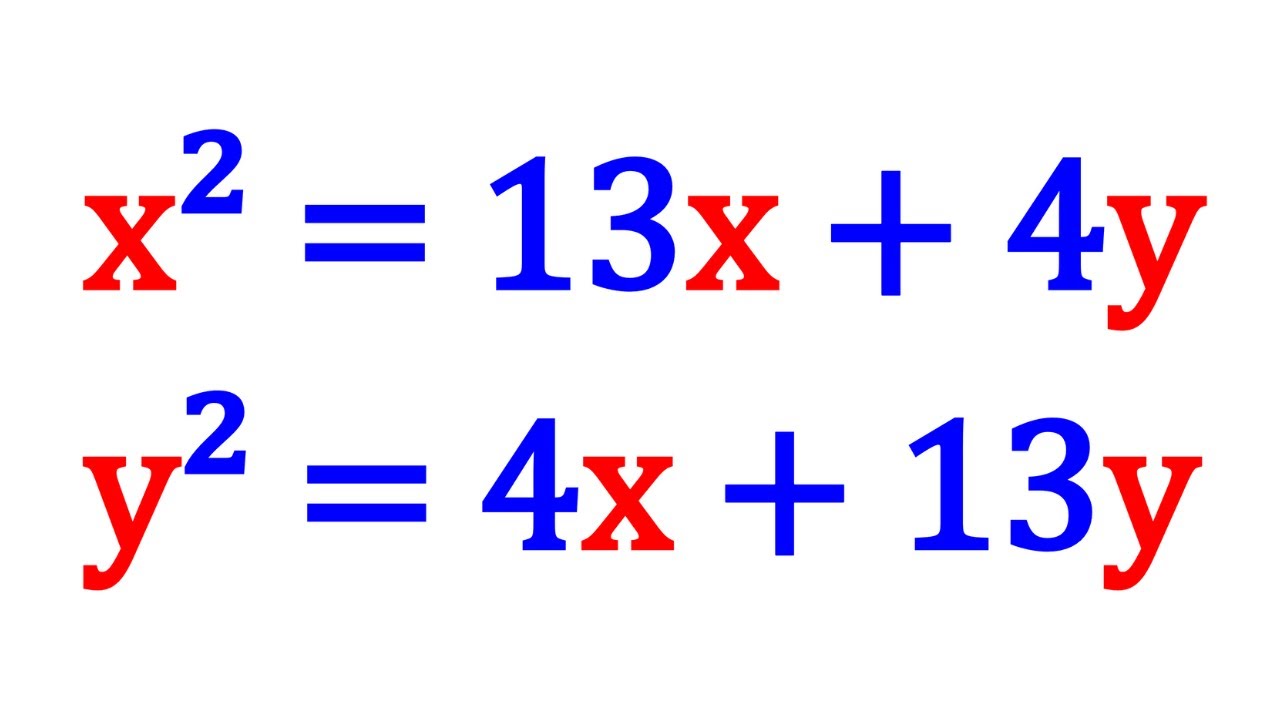
Показать описание
🤩 Hello everyone, I'm very excited to bring you a new channel (SyberMath Shorts).
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#algebra #polynomials #function
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#algebra #polynomials #function
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Solving A Nice Polynomial System | Algebra
A Nice Polynomial System
A Nice Polynomial System
A Nice Polynomial System #algebra
A Nice Polynomial System
Solving a Nice Polynomial System in Two Ways
Solving a Nice Polynomial System in Two Ways
Solving a Nice Polynomial System in Two Ways
Exponential equation. #maths #Mathematics #mathstutor #mathsisfun #exponential
A Nice Polynomial Equation | Algebra
A Nice Polynomial System | #algebra #polynomials
Let's Solve A Nice Polynomial System
I Solved A Nice Polynomial System
A Very Nice Polynomial System
A Nice Polynomial System by Stanley Rabinowitz
Solving A Nice Polynomial System | Two Ways
A Nice, Quick, and Easy Polynomial System
Solving a Nice Polynomial Equation
Solving A Polynomial System
A Nice Polynomial Equation
A Nice Polynomial Equation from Croatia 🇭🇷
Solving A Nice Polynomial System by Math26039335
A Nice Polynomial System | Math Olympiads
Relationship between zeros and coefficient of a polynomial #ncert #math #class10
Комментарии
 0:08:57
0:08:57
 0:08:40
0:08:40
 0:08:13
0:08:13
 0:09:25
0:09:25
 0:08:19
0:08:19
 0:09:03
0:09:03
 0:09:27
0:09:27
 0:08:38
0:08:38
 0:01:01
0:01:01
 0:10:00
0:10:00
 0:08:16
0:08:16
 0:10:27
0:10:27
 0:06:23
0:06:23
 0:10:31
0:10:31
 0:10:27
0:10:27
 0:10:56
0:10:56
 0:08:27
0:08:27
 0:05:53
0:05:53
 0:00:53
0:00:53
 0:00:51
0:00:51
 0:09:27
0:09:27
 0:11:06
0:11:06
 0:00:37
0:00:37
 0:00:18
0:00:18