filmov
tv
Proof: e^x is Continuous using Epsilon Delta Definition | Real Analysis Exercises
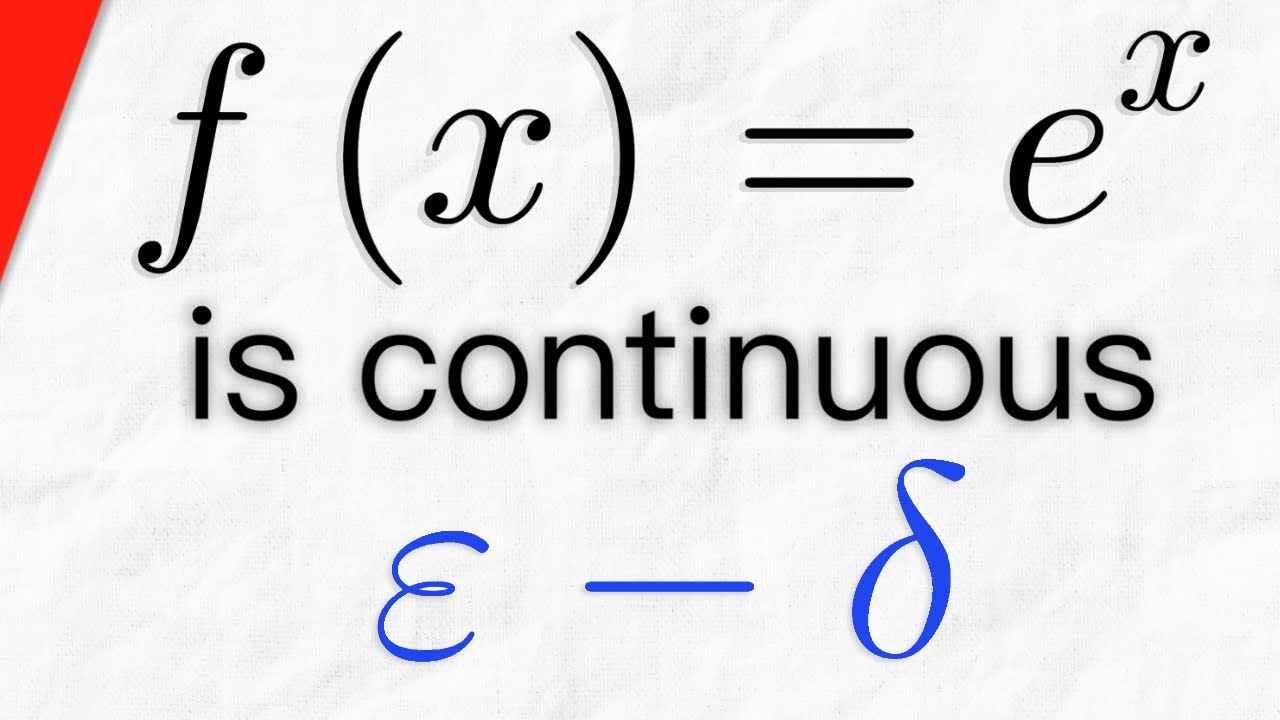
Показать описание
We prove that f(x)=e^x, the natural exponential function, is continuous on its entire domain - the real numbers. We complete this proof using the epsilon delta definition of continuity of a function at a point. To do this, we simply take an epsilon greater than 0 and an arbitrary point c from our domain, then go through the motions of finding a delta greater than 0 so that any x in D that is within delta of c has an image within epsilon of c's image. However, we'll quickly find that our problem gets reduced to proving e^x is continuous at 0. Also, we will be using the power series definition of e^x for this proof. Let me know if there are more epsilon delta continuity proofs you want to see!
#RealAnalysis #Math
★DONATE★
Thanks to Robert Rennie, Barbara Sharrock, and Rolf Waefler for their generous support on Patreon!
Follow Wrath of Math on...
#RealAnalysis #Math
★DONATE★
Thanks to Robert Rennie, Barbara Sharrock, and Rolf Waefler for their generous support on Patreon!
Follow Wrath of Math on...
Proof: e^x is Continuous using Epsilon Delta Definition | Real Analysis Exercises
Proof: f(x) = |x| is Continuous using Epsilon Delta Definition | Real Analysis Exercises
Proof: f(x) = x is Continuous using Epsilon Delta Definition | Real Analysis Exercises
proving x^2 is continuous using the epsilon delta definition
Proof: ax+b is Continuous using Epsilon Delta Definition
Proof f(x)=sin(x) is Continuous using Epsilon Delta Definition | Real Analysis Exercises
Prove that exponential function is a continuous function | In English
Proof: sqrt(x) is Continuous using Epsilon Delta Definition | Real Analysis Exercises
Lecture 4(PDEs): Fourier Theorem
Proof that f(x) = 1/x is Continuous on (0, infinity) using Delta-Epsilon
Real Analysis Proofs - Exponential Function is Continuous
3 Step Continuity Test, Discontinuity, Piecewise Functions & Limits | Calculus
Differentiable then Continuous Proof
Show that f(x) is continuous at x=4
Proving x^2 is continuous (with epsilon delta definition)
How to prove a function is not continuous using limit definition of continuity | Math with Janine
Determine if the Piecewise Function is Continuous by using the Definition of Continuity
e to the power x is a Continuous function
Proof of a Limit Value Using Epsilon and Delta
Polynomials and Rational Functions are Continuous | Real Analysis
How to determine if a function is continuous and differentiable
What is the number 'e' and where does it come from?
What's so special about Euler's number e? | Chapter 5, Essence of calculus
Prove the Piecewise Function is Continuous with the Delta-Epsilon Definition of Continuity
Комментарии
 0:14:36
0:14:36
 0:04:19
0:04:19
 0:04:46
0:04:46
 0:09:35
0:09:35
 0:03:40
0:03:40
 0:05:19
0:05:19
 0:06:54
0:06:54
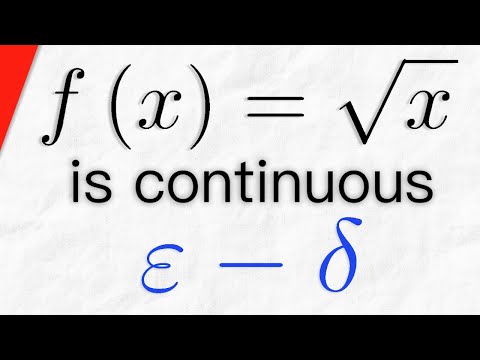 0:09:22
0:09:22
 0:43:55
0:43:55
 0:09:56
0:09:56
 0:05:04
0:05:04
 0:10:10
0:10:10
 0:03:51
0:03:51
 0:01:51
0:01:51
 0:01:37
0:01:37
 0:04:18
0:04:18
 0:04:01
0:04:01
 0:03:18
0:03:18
 0:09:17
0:09:17
 0:04:44
0:04:44
 0:02:21
0:02:21
 0:07:58
0:07:58
 0:13:50
0:13:50
 0:07:47
0:07:47