filmov
tv
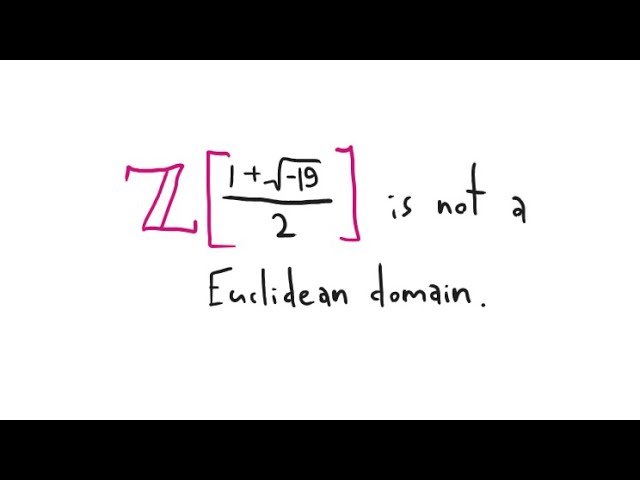
Proof that Z[(1+sqrt(-19))/2] is not a Euclidean domain
Показать описание
This is an example of a ring which is a PID but is not a Euclidean domain.
Proof that Z[(1+sqrt(-19))/2] is not a Euclidean domain
Abstract Algebra | A PID that is not a Euclidean Domain
5 simple unsolvable equations
Do you remember the square roots of these perfect squares? 🤯 #Shorts #math #maths #mathematics
NEWYES Calculator VS Casio calculator
Multiples of 8 😎 #Shorts #math #maths #mathematics
Among Us in HD (Part 89) SHRINK LOOP #Shorts
Why is pi here? And why is it squared? A geometric answer to the Basel problem
Calculus 1 Exam Review Part8
IIT Bombay CSE 😍 #shorts #iit #iitbombay
HOW CHINESE STUDENTS SO FAST IN SOLVING MATH OVER AMERICAN STUDENTS
4 in 1 about smoking and drinking #rifanaartandcraft #ytshorts #animationvideo #stopsmoking #ytshort
1+omega+omega square=|value of omega in complex no|1 w w2 are cube roots of unity|1+w+w2=?
Basic Algebra Tips
19. Problems on 1 by sqrt of ax^2+bx+c
JEE Aspirants ka Sach 💔 #JEE #JEEMain #Shorts
Square Shortcut Trick🔥| Square 21-30 Tricks #math #shorts #shortvideo #short #trending #vedicmaths...
Quadratic Equations IIT Questions No 11 ( X Class)
That's Why Mohit Sir Called 'God Of Mathematics'| Puzzle Brain teaser | #competishun ...
😁 Playing 🐍Snake🐍 game on calculator 😜 [official video] #shorts #viral #casio
Maths Ki Problem 😜 PIHOOZZ
An Algebra Problem | x^2+y^2+z^2=xy+xz+yz
MATHS PROBLEM | Try to solve #Shorts PIHOOZZ
Quadratic Equations IIT Questions No 05 ( X Class)
Комментарии
![Proof that Z[(1+sqrt(-19))/2]](https://i.ytimg.com/vi/PDMVgT4Tka8/hqdefault.jpg) 0:13:49
0:13:49
 0:36:49
0:36:49
 0:00:50
0:00:50
 0:00:11
0:00:11
 0:00:14
0:00:14
 0:00:20
0:00:20
 0:00:08
0:00:08
 0:17:08
0:17:08
 3:10:17
3:10:17
 0:00:11
0:00:11
 0:00:23
0:00:23
 0:00:16
0:00:16
 0:00:09
0:00:09
 0:00:48
0:00:48
 0:17:01
0:17:01
 0:00:12
0:00:12
 0:00:19
0:00:19
 0:00:50
0:00:50
 0:00:19
0:00:19
 0:00:47
0:00:47
 0:00:23
0:00:23
 0:00:53
0:00:53
 0:00:31
0:00:31
 0:00:57
0:00:57