filmov
tv
Euler's Formula Using Taylor Series Expansions

Показать описание
Today, we prove Euler's formula using Taylor series.
Using Taylor Series to Derive Euler's Identity (Bonus Included)
Euler's Formula & Euler's Identity - Proof via Taylor Series
Euler's Formula Using Taylor Series Expansions
Taylor Series of the Exponential Function and Euler's Formula!
Using Taylor Series to Find Euler’s Identity! #calculus #calculushelp #mathematics #taylorseries
Euler's Formula Proven using the Taylor Series
The Euler's formula explained!
Deriving Euler's Formula from Taylor Series
Euler's Equation from Taylor series
Derivation of Euler's Relation using Taylor Series Expansion | Euler's Identity
Taylor Swift explains the Taylor series in 90 seconds
Taylor series | Chapter 11, Essence of calculus
Proof of Euler's Formula Without Taylor Series
Euler's Formula Proof
Derive Euler's Formula by Maclaurin Series Expansion
The Euler Formula
Euler's Formula Proof with Taylor Expansion
A nice approach to Euler's formula
Eulers formula
Taylor Series - 7 - Exponential Function and Euler's Formula
Deriving Euler’s Equations from Taylor Series expansions
e^(i*pi)+1=0: No Taylor Series or Euler's Formula
e^(iπ) in 3.14 minutes, using dynamics | DE5
Euler's Formula - Proof WITHOUT Taylor Series
Комментарии
 0:08:01
0:08:01
 0:06:56
0:06:56
 0:05:05
0:05:05
 0:10:14
0:10:14
 0:00:52
0:00:52
 0:18:24
0:18:24
 0:00:33
0:00:33
 0:12:37
0:12:37
 0:17:28
0:17:28
 0:11:54
0:11:54
 0:01:29
0:01:29
 0:22:20
0:22:20
 0:03:57
0:03:57
 0:00:56
0:00:56
 0:10:17
0:10:17
 0:00:20
0:00:20
 0:05:57
0:05:57
 0:00:39
0:00:39
 0:08:42
0:08:42
 0:17:34
0:17:34
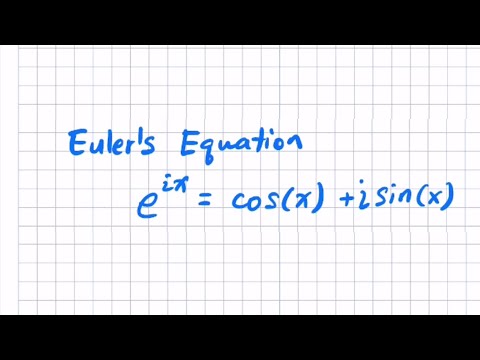 0:02:34
0:02:34
 0:08:58
0:08:58
 0:04:08
0:04:08
 0:08:51
0:08:51