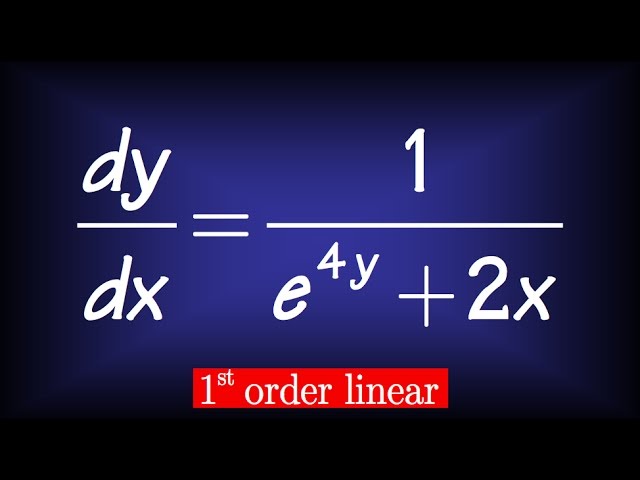
First Order Linear Differential Equation, 2.3#29

Solve dy/dx=1/(e^2y+2x),

Part1 of Differential Equation Course: How to solve first order differential equations? The topics/technique include:

separable differential equations,
first order linear differential equations,
integrating factor,
exact differential equations,
special integrating factor,
solve differential equations by substitution,
homogeneous differential equations,
bernoulli differential equation,

(this playlist provides the ideas behind each kind of diff eq, the strategies to solve each kind of diff eq, and plenty of examples/homework examples)

YOU CAN'T SOLVE DIFFERENTIAL EQUATION WITHOUT KNOWING YOUR INTEGRALS!

blackpenredpen

[aaronhooper]

That was an awesome solution! Your videos just keep getting better.

[andresvasquez]

But aren't you assuming that there exists an inverse function? How would you solve for y(x)? Because that assumption already restricts the solutions that we get by saying that all solutions have an inverse, so all solutions that we get will be of that form

[hosseinazizi]

Very beautifully explained, thank you

[infinitymfg]

I liked that problem and the technique that you used.

[bird]

Thank u so much bro ! You helped me with integrals and now you are helping me with differentials Equations !!

[AlgyCuber]

y = 1/2 ln(C+sqrt(C^2+2x)) or 1/2 ln(C-sqrt(C^2+2x))
good solution

[pco]

The initial question says dy/dx, doesn't that imply that the independent variable is x? In that case, shouldn't you give the final answer as a function in terms of x?

[fountainovaphilosopher]

At the end, you can factor the e^4y out and have a part 0.5 + C. As much as I know, we can add the constants to get another constant, and end up with a simpler solution, Ce^4y.

[MrCorto]

Sorry, but you can't invert dy/dx because it's not a fraction! the real notation is d/dx (of y) = y' (and by the way, you can solve it for y).