filmov
tv
Find area of a triangle inside of a parallelogram.

Показать описание
How to Find the Area of a Triangle | Calculate the Area of a Triangle
Area of a Triangle | MathHelp.com
Finding the Area of a Triangle | A Step-By-Step Guide | Math with Mr. J
Area of a Triangle 🔺 Math for Kids 🏠
Math Antics - Area
Area of a Triangle, Given 3 Sides, Heron's Formula
Area of a Triangle Formula Explained!
Example finding area of triangle
Right TRIANGLE 📐| How to find Area of Right Triangle, Perimeter of a Triangle| Perpendicular height...
Area of Triangle
Finding the area of any triangle
Find the area of an oblique triangle using a formula
Formula for Area of a Triangle - Why?
How To Find The Area of an Isosceles Triangle - Math
AWESOME Formula – AREA of a TRIANGLE (Herons Formula)
Find the Area of the Blue Triangle Inside of a Rectangle
How to find the area of a triangle using heron's formula
Area of Triangle = ½ x b x h Why? | Fun Math | Don't Memorise
How to find height and understand the formula for area of an oblique triangle
How to Find the Area and Perimeter of a Triangle | Math with Mr. J
Area of a Triangle: ½absinC
Area of a Triangle - 3 different samples Including Heron's Formula
Area of a Triangle (Base and Height)
Area of a Triangle With Vertices - Geometry
Комментарии
 0:06:37
0:06:37
 0:01:36
0:01:36
 0:07:35
0:07:35
 0:03:11
0:03:11
 0:10:26
0:10:26
 0:13:39
0:13:39
 0:09:06
0:09:06
 0:01:30
0:01:30
 0:04:32
0:04:32
 0:06:15
0:06:15
 0:11:15
0:11:15
 0:04:07
0:04:07
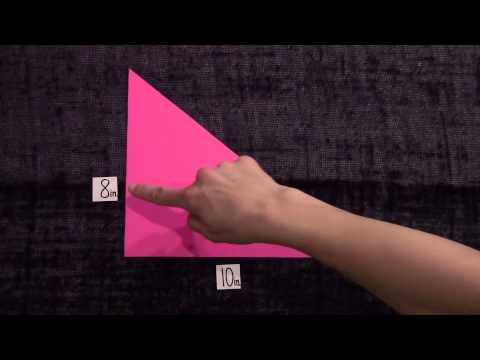 0:02:34
0:02:34
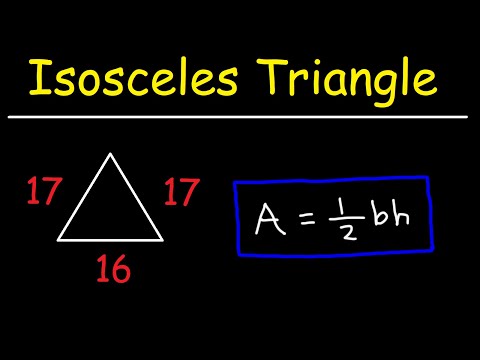 0:12:35
0:12:35
 0:14:19
0:14:19
 0:09:45
0:09:45
 0:03:55
0:03:55
 0:01:01
0:01:01
 0:07:38
0:07:38
 0:05:48
0:05:48
 0:11:14
0:11:14
 0:08:12
0:08:12
 0:01:57
0:01:57
 0:05:06
0:05:06