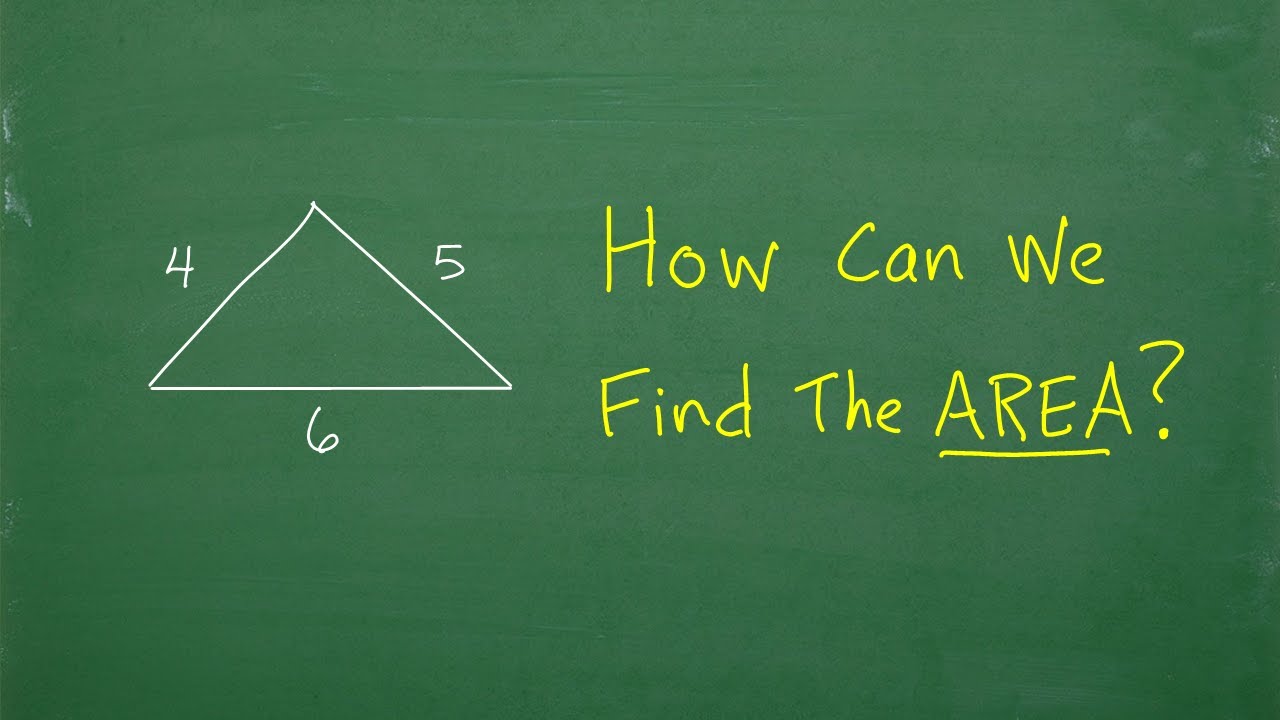
AWESOME Formula – AREA of a TRIANGLE (Herons Formula)

TabletClass Math:


Math Notes:

[ShanDysigns]

The 'quick' introduction lasted 3 minutes and 48 seconds, that's 26.54% of the whole video. I don't understand these long, meandering introductions on your videos. I love watching math techniques, I just find these marathon intros to be pointless.

[davidbrisbane]

Name the vertices of the triangle as follows. Botom left is A, top is B and bottom right is C

The area of the triangle is
(1/2)*c*b*sin(A) =(1/2)*4*6*sin(A).

By the cosine rule
5² = 4² + 6² - 2*4*6*cos(A)
⇒ cos(A) = (4² + 6² -5²)/(2"4*6) = 27/48
⇒ A = 55.7711° (4 decimal placed)
⇒ sin(A) = sin(55.7711°) = 0.8268 (4 decimal placed)

So, Area of ΔABC
= (1/2)*4*6*sin(A)
= (1/2)*4*6*0.8268
= 9.9216.

Interestingly, this approach can be used to prove Heron's formula. If instead of computing the area with numbers we use the side length of a, b, c, then we could have found sin(A) from cos(A) using the fact that sin²(A) + cos²(A) = 1,
so sin(A) = √(1 - cos²(A)).

We let s = (a + b + c)/2 and then we apply some algebra on s, a, b & c in the equation, noting how to factorise the difference between squares (twice) to find sin(A).

From there it is an easy step to find the area of the triangle using area = (1/2)*c*b*sin(A) and we end up with Heron's formula.

[johndonne]

My wife and have 4 advanced degrees between us including PhD’s. I have a professional license to teach 7-12. My mentor was teacher of the year in America. I’ve taught mathematics mastics at virtually every level up to entry level graduate studies. This man represents everything that’s wrong with math education. He droned on for more than half the video without saying a single relevant word. He then distracts the student with useless references to law of sines/cosines which at this level would be utterly opaque to the students. Then “magically “ a formula appears. Absolutely no intuition motivating it, and absolutely no mathematical reasoning as to how this formula is derived. He then instructs students to blindly apply this formula, and does a poor job of that as well. Moreover, he speaks for 15 minutes of which around 3 are actually relevant to the problem. To believe that this drivel is within the attention span of a teen student is delusional. This isn’t remotely on the spectrum of modern mathematics education. This could be used as an example of what is wrong in math education in this country. Somebody stop him before he disenfranchises another child from learning to reason mathematically.

[danmyers]

Not knowing Heron’s Formula, I went the long way around the barn. I drew in the altitude as you did. Now I have two triangles with a common height and bases of X and 6-X. I used the Pythagorean Theorem to write the equations for h^2 of each triangle, set them equal to each other and solved for X. Using that information, I calculated the areas of the two triangles, added them together and got the same 9.92 answer, it just took a little longer (maybe). FYI, h= 3.31 and X=3.75

[knotwilg]

Heron's formula actually gives the simplified solution 15/4 * sqrt 7 = 9.92...
All the short cuts mentioned in the comment section start from the illusion that the upper angle is 90 degrees. The drawing is deceptive and the angle is close, about 83 degrees.
So if you double the triangle it won't give a new triangle because the left leg won't continue in a straight line (180 degrees).

[shujaathusain]

Find one of the angles by using the cosine rule. Then get the area using the sine rule: Area = 0.5 a x b x sinc

[Dave-zusm]

Just use cosine rule to get the an angle, then use ½abSin(C)

[christopherbedford]

In any Math exam I ever took, writing down a formula for the desired result and then calculating it would get you one point for knowing how to apply it to the specific case, and maybe a second point for doing the arithmetic correctly, out of a possible max of probably 4 or 5 points -- *IF* the formula is taught in the curriculum.

If it _isn't, _ (and I never learned this one in 12 years of school and three of university) then writing down this formula and calculating the answer would probably get you no marks at all because the whole point of Math tests is not to come up with an answer like a History exam but to _work it out._

[Brettly]

4 5 6 means 4 and 5 are at 90degrees
Draw a line opposite 4 and 5 at the same measurements.
Now you have a 4x5 rectangle, 4X5=20
half of 20 is 10 (cos you want to know the area of the triangle which is half the rectangle)
The answer is 10

[joekelley]

I flipped the top down and made a rectangle but also had to flip sideways so that it was a perfect rectangle 4 by 5. Makes 20 square and had to half it making the correct answer 10 square.

[LordWilsonVILLA]

I got the answer to 10 in a lot simpler way = 4x5 = 20 which is the area of a square of 4 by 5 and halve it to get the area of the triangle = 10 - simple!!!! To find the area as described in this video is why so many students get so bored with maths YES MATHS!!! The abbreviation of mathematicS is MATHS!!! ;-)

[julietmoraa-tenu]

Area of shapes involving rectangle, square and triangles

[terryjohinke]

I used to teach Heron's Formula, along with pythagos' Teorem and trigonometry. We'd use markers of three types as mentioned and students assignment was to calculate an area, on the football oval, to use all these formulas. Yhat was year 11, here that"s pre last year of high school. Good fun. Lots of number crunching if the problem is a fraction or decimal. So we set up problems that s added to an equal number, dicided by 2 to get s. Easier to teach if it's not as cumbersome as this one.

[virgiliobartolo]

Nice formula but you didn't show how this formula was derived like what is "s" referring to. You just used the formula and plugged the numbers and I don't see mathematical justifications as to where the formula came from. But thank you and I will appreciate it if you can prove the validity of the formula.

[tonywright]

Like Dan Myers, I started out with the Pythagorean Theorem and tried to use what I call substitution of equations to find the height. I was too lazy to work it all out but my gut feeling is that by using that method, you might wind up deriving Heron's Formula. I'm not sure of that. It's just intuition. But at the end of the day, I thank Heron and I thank you for telling me about that formula.

[timspooner]

My maths teacher 12 to 18 was fantastic. Home work every night was a published maths test of 90 minutes.99% of my cohort passed O and A levels (UK).

[olivierhabineza]

First you find the half of 4+5+6=15 that is 7.5. Then you find the square root of the product 7.5(7.5-6)(7.5-5)(7.5-4)=7.5*1.5*2.5*3.5=98.4375, that is 9.9215.... square units. Thank you!

[lolitotorlao]

You can use also cosine law by finding unknown height, then apply the formula of finding the ares of triangle..tnx

[brianwalters]

Showing students how to use a formula is not teaching mathematics. Problem solving and formula derivation is always the best approach. It will develop a true understanding.

[Cladman]

Easy, make two right Triangle and calculate the area as 1/2 x base and the height. Then multiply by 2