A (correct proof) of 0.99=1 | Sequences and Limits | Math Basics | Math2Go

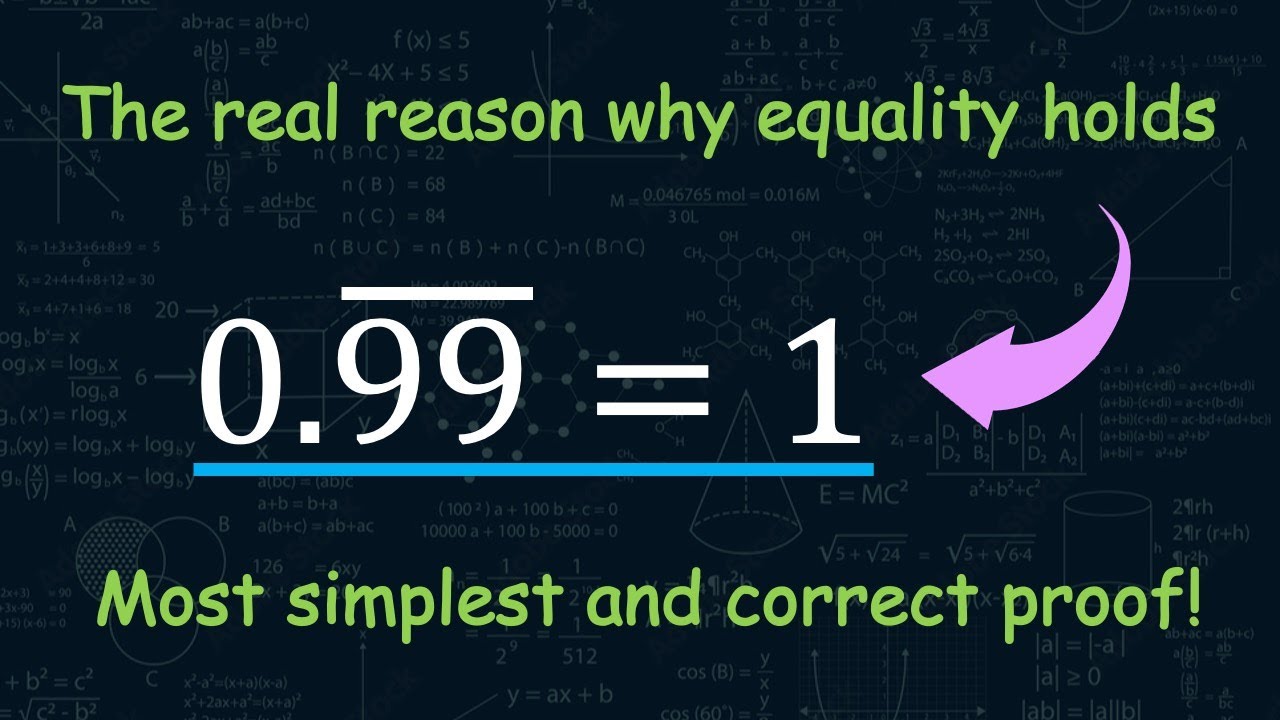
preview_player
Показать описание
In this video we will look into the well known fact that 0.99 period ("repeating") equals 1! As alot of proofs out there are mathematically wrong, we will take a different approach and prove the statement using sequences and limits.

Playlists:

If you have any questions please feel free to ask. You can also ask for specific other mathematical problems in the comment section below!

Please comment and subscribe if this video was helpful to you and you wan to stay tuned for more helpful math videos!
  1. Math2Go
  2. mathematics
  3. maths
  4. proof
  5. math
  6. numbers
Рекомендации по теме
A (correct proof) 0:04:37

A (correct proof) of 0.99=1 | Sequences and Limits | Math Basics | Math2Go

Does 0.999... = 0:02:27

Does 0.999... = 1?

0.9 Repeating Equal 0:01:01

0.9 Repeating Equal to 1 Algebraic Proof | Fun Math JusticeTheTutor #shorts #maths #math

Every PROOF you've 0:09:25

Every PROOF you've seen that .999... = 1 is WRONG

Pizza proof 0.999... 0:00:57

Pizza proof 0.999... = 1

Simple Proof That 0:01:00

Simple Proof That 0.99 Repeating Is Equal To 1:

Proving 0.9999....=1 0:00:29

Proving 0.9999....=1 A marvellous proof #shorts

Infinite Math Test 0:06:02

Infinite Math Test (Proof that 0.999... = 1)

Simplest proof that 0:01:59

Simplest proof that 0 999 is not equal to 1

1/0 = Undefined 0:04:14

1/0 = Undefined or Infinity: Easy proof to understand with a real world example

1 + 1 0:08:25

1 + 1 = 3 Proof | Breaking the rules of mathematics

MATH: Explaining Formal 1:02:41

MATH: Explaining Formal Proof that .999... = 1

proof you’re not 0:00:12

proof you’re not ugly😳 #confidence #shorts

0.9 Repeating Equal 0:00:18

0.9 Repeating Equal to 1 Algebraic Proof | Fun Math #shorts #maths #mathstricks #priyanka_prajapati

Pee Safe XXL 0:00:25

Pee Safe XXL Ultra Thin Sanitary Pads | Leak proof Sanitary Napkins | Extra Long Ultra Thin Pads

I Learned How 0:07:36

I Learned How to Divide by Zero (Don't Tell Your Teacher)

'99 Percent' Miss 0:03:49

'99 Percent' Miss This. What Is The Length?

The Simple Question 0:07:06

The Simple Question that Stumped Everyone Except Marilyn vos Savant

Fortnite makes no 0:00:15

Fortnite makes no sense...

Spanish Mathematical Olympiad 0:07:54

Spanish Mathematical Olympiad | Can You Proof 1/a^1999+1/b^1999 +1/c^1999=1/(a^1999 +b^1999+c^1999)

NASA ROVER SPOTS 0:00:41

NASA ROVER SPOTS ALIENS | The Proof Is Out There | #Shorts

The Beautiful Peacock 0:00:08

The Beautiful Peacock 1 Oz Gold Proof Coin #peacock #gold #emkcoins

Proof Air Purifier 0:00:09

Proof Air Purifier WORKS 🌬 #watchthis 👀

258. Add Digits 0:09:32

258. Add Digits | O(1) with Mathematical Proof | LeetCode Daily Challenge | LeetCode POTD

Комментарии
Автор

Noo! In the sequence x_n we are not taking the limit of x facing infinity, but the limit of *n* going towards infinity!

pseudoexpertise
Автор

I see further problems with the definition of limits and how math makes infinity finite.

I am using middleschool basics to show that ".99..." does not exactly equal 1.

Real numbers are unique and precise.

Decimal place notation has been used to represent numbers for over 20 centuries.

In decimal place notation, integers do not have nonzero digits to the right of the decimal point. ".99..." should not represent a integer.

Real numbers are a continuum that consists of zero, natural, integers, rational and Irrational numbers.

A rational number is the ratio of 2 integers. There are no two integers that result in ".99...".

The limit, while by definition, has to be a real number, the limit does not have to exactly equal the entity, function or sequence it refers to. ".99..." can't be a limit. (Nobody has said it was.)

The main problem is how math treats infinity. Infinity by simple definition:
Incomplete, (never ending)
Inconsistent, (different "sizes")
and imprecise (has no boundries)

It is not possible to represent every real number in decimal (or any number base) placed number notation. What is the number adjacent to 1. The nature of the real numbers being unique and precise and they are a continuum, there is no way to conceive of a number next to 1 in base placed number notation.

If you raise ".99..." to increasing n powers you get
.99...980...01" not simply ".99..." or 1

johnlabonte-chul