filmov
tv
Every PROOF you've seen that .999... = 1 is WRONG
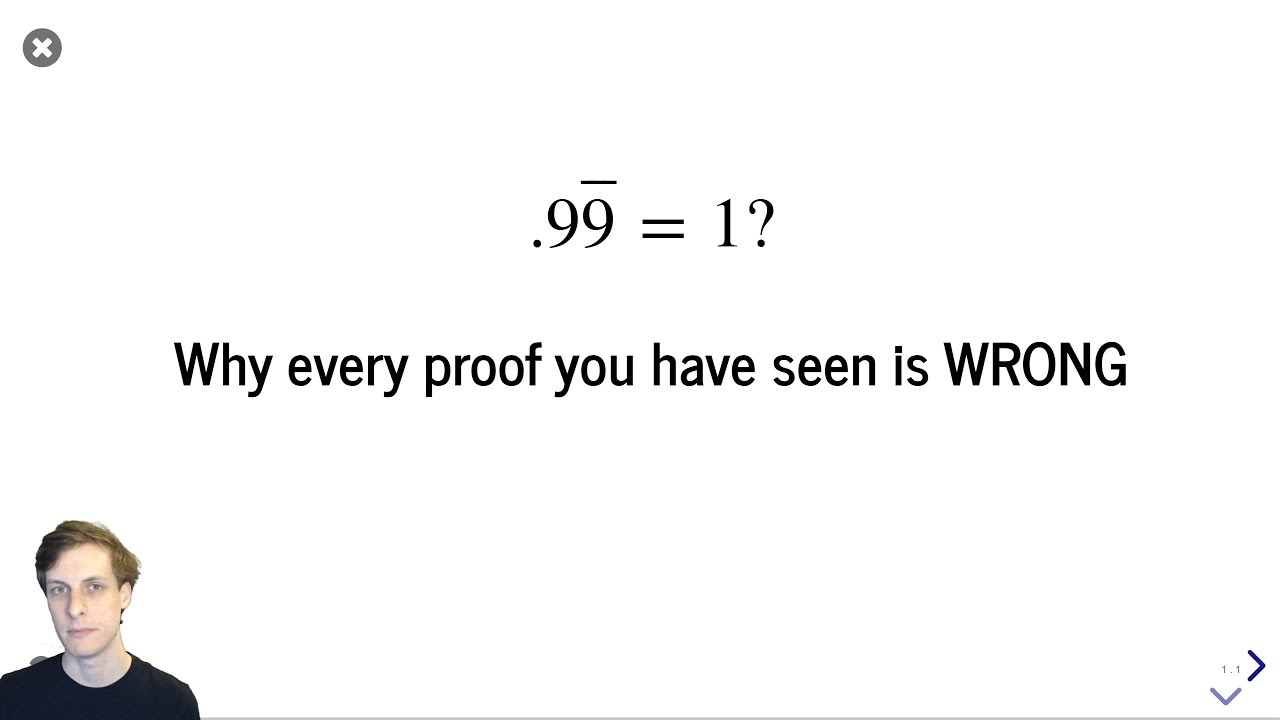
Показать описание
It's true, so why so many wrong proofs?
There are many incorrect proofs that .999... = 1 on YouTube and elsewhere. In this video we see why all those proofs are wrong, and then demystify what is actually meant when a mathematician says .999... = 1 and I explain why it is actually true. Did your favorite math creator make one of these mistakes?
*Title only applies before the first viewing of this video and only to people who have not studied real analysis.
References on the standard definition of decimals and repeating decimals (please read if you are confused or unsure about the standard notation):
SUPPORT ME ⭐
---------------------------------------------------
BE ACTIVE IN MY COMMUNITY 😄
---------------------------------------------------
There are many incorrect proofs that .999... = 1 on YouTube and elsewhere. In this video we see why all those proofs are wrong, and then demystify what is actually meant when a mathematician says .999... = 1 and I explain why it is actually true. Did your favorite math creator make one of these mistakes?
*Title only applies before the first viewing of this video and only to people who have not studied real analysis.
References on the standard definition of decimals and repeating decimals (please read if you are confused or unsure about the standard notation):
SUPPORT ME ⭐
---------------------------------------------------
BE ACTIVE IN MY COMMUNITY 😄
---------------------------------------------------
Every PROOF you've seen that .999... = 1 is WRONG
Visual proof every student should see
Zero Knowledge Proof (with Avi Wigderson) - Numberphile
Proof That Chinese is the Easiest Language
Proof that no every Hänno-Foto can be an album cover
Methods of Proof | A-level Mathematics
How To Tell If You’re A Psychopath With This Simple Test
Scientists Submit Actual Proof Aliens Are Watching You Right Now
Scientists Found Proof of GOD in DNA Code - Evidence of God - The God Code - God DNA
The Proof Is Out There: Strange Creatures in Silver Suits Discovered by Russian Divers (Season 4)
Beca Shakes Things Up (Bullet Proof) | Pitch Perfect | TUNE
No Human Has Ever Left Earth’s Atmosphere, Here's Why
Proof a Mysterious Lost Ancient GLOBAL Civilization Spanned Virtually the Entire Planet…
here’s proof that you can be fit & NEVER count calories
Beautiful, intelligent, and talented, Ysabel Ortega is proof that you really can have it all!
Heron's Formula Proof (the area of a triangle when you know all three sides)
Secret Reasons You Don't Know About Everyday Things
What Do You Swear You Saw But Have No Proof? (r/AskReddit)
Fool-Proof Test for Primes - Numberphile
PROOF - God Doesn't Leave You! - Job Testimony #encouragement
AUDC: JoJo BREAKS DOWN Over Technique Criticism (Season 2 Flashback) | Lifetime
Ways to show Proof of income when you are Self employed
Are you looking for a transfer proof lipstick that lasts all day?
10 Tips for SWEAT PROOF Makeup That Lasts All Day!
Комментарии
 0:09:25
0:09:25
 0:00:57
0:00:57
 0:33:38
0:33:38
 0:00:33
0:00:33
 0:00:23
0:00:23
 0:13:35
0:13:35
 0:01:34
0:01:34
 0:10:08
0:10:08
 0:07:14
0:07:14
 0:06:25
0:06:25
 0:01:55
0:01:55
 0:05:10
0:05:10
 0:31:07
0:31:07
 0:00:35
0:00:35
 0:00:29
0:00:29
 0:10:37
0:10:37
 0:25:14
0:25:14
 0:17:25
0:17:25
 0:03:43
0:03:43
 0:30:34
0:30:34
 0:05:09
0:05:09
 0:02:41
0:02:41
 0:00:06
0:00:06
 0:06:13
0:06:13