filmov
tv
Complex Exponentials to Solve the Laplace Transform of cos(at)

Показать описание
Typically, solving for the Laplace Transform of the sin(at) and the cos(at) involves two rounds of integration by parts. That isn't fun. The way to make it fun and much simpler is to rephrase the cosine function as a function of complex exponents with Euler's Formula. Then, several properties of the Laplace Transform make the solution short and sweet.
A Complex Exponential Equation with A Surprising Answer
e^(iπ) in 3.14 minutes, using dynamics | DE5
Solving Exponential Equation
Exponential form to find complex roots | Imaginary and complex numbers | Precalculus | Khan Academy
Response to Complex Exponential
What is a Complex Exponential?
Solving Exponential Equation
Solving Exponential Equations
Mastering Logarithmic Differentiation: Solving Complex Exponential Derivatives | d/dx[𝑎^(𝑎^𝑥 )]...
Eulers formula
Exponential Equations - Algebra and Precalculus
Complex Exponentials to Solve the Laplace Transform of cos(at)
Complex Analysis Episode 12: The Complex Exponential Function
Exponential Form to Logarithmic Form #Shorts #algebra #math #maths #mathematics #lesson #howto
The Euler's formula explained!
Rant: The Glory of Complex Exponentials
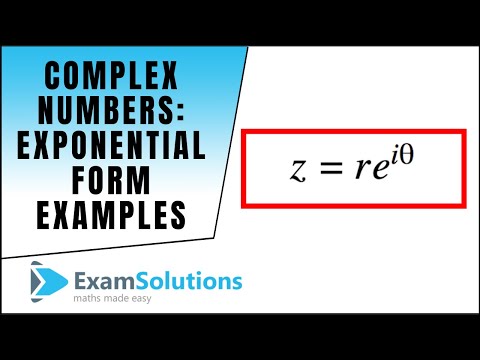
Complex Numbers - Exponential Form Examples : ExamSolutions Maths Tutorials
Exponential Form of a Complex Number
Transforming a Complex Number in to Exponential form
Can you solve this? | Exponential Equation | Algebra Problem.
Identity with the Complex Exponential - Complex Analysis Proof
Solving Complex Exponential Equations
Cosine and Sine Functions in terms of Complex Exponentials || Derivation from Euler's Formula
Absolute Value of Complex Number
Комментарии
 0:10:22
0:10:22
 0:04:08
0:04:08
 0:00:56
0:00:56
 0:11:53
0:11:53
 0:12:51
0:12:51
 0:00:53
0:00:53
 0:00:54
0:00:54
 0:16:36
0:16:36
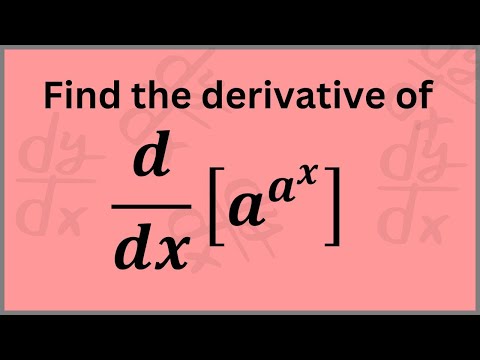 0:03:36
0:03:36
 0:08:42
0:08:42
 0:05:58
0:05:58
 0:11:42
0:11:42
 0:04:30
0:04:30
 0:00:22
0:00:22
 0:00:33
0:00:33
 0:07:46
0:07:46
 0:09:42
0:09:42
 0:03:15
0:03:15
 0:05:39
0:05:39
 0:03:33
0:03:33
 0:07:19
0:07:19
 0:07:55
0:07:55
 0:11:21
0:11:21
 0:00:45
0:00:45