filmov
tv
Absolute Value of Complex Number

Показать описание
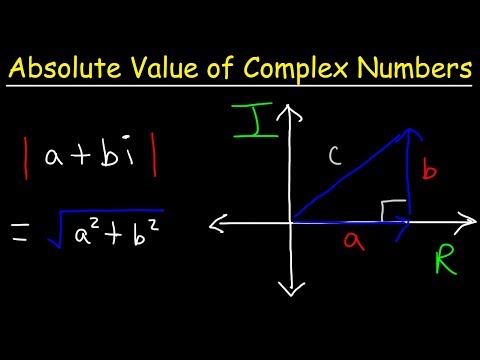
Absolute Value of Complex Numbers
Absolute value of a complex number | Imaginary and complex numbers | Precalculus | Khan Academy
Absolute Value of a Complex Number
Tutorial - Finding the absolute value of a complex number ex 1, (4 + 3i)
Absolute Value of a Complex Number
Tutorial - Finding the absolute value of a complex number ex 2, (10 - 7i)
The Absolute Value of a Complex Number
Finding the Absolute Value (Modulus) of a Complex Number (Example)
Absolute Value of Complex Number
Finding the Absolute Value (Modulus) of a Complex Number (Example)
Modulus of Complex Numbers
Tutorial - Finding the absolute value of a complex number ex 5, (4 + i)
Finding the absolute value of complex numbers
Tutorial - Finding the absolute value of a complex number ex 4, (-8i)
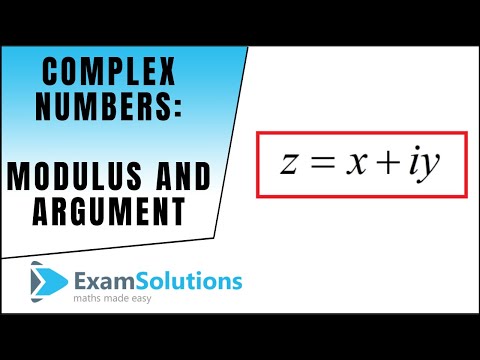
Complex Numbers : Modulus and Argument | ExamSolutions
How to Write Absolute Value of Complex Number
Complex Numbers from Absolute Value and Angle: Practice
Modulus (Absolute Value) of Complex Numbers: Formula
find absolute value of imaginary numbers
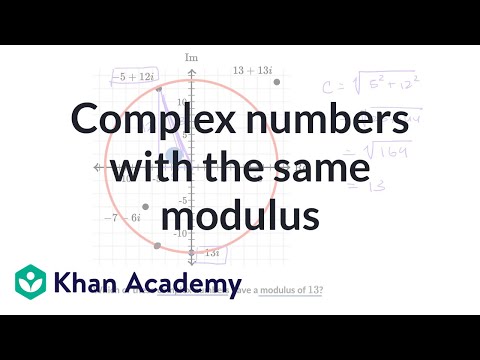
Complex numbers with the same modulus (absolute value)
Modulus of Complex Number - Absolute Value of Complex Number
Finding the Absolute Value of Complex Numbers
Complex Numbers: Graphing and Absolute Value
How To Solve Absolute Value Equations, Basic Introduction, Algebra
Комментарии
 0:02:46
0:02:46
 0:03:33
0:03:33
 0:01:58
0:01:58
 0:01:14
0:01:14
 0:02:20
0:02:20
 0:01:26
0:01:26
 0:09:22
0:09:22
 0:01:56
0:01:56
 0:00:45
0:00:45
 0:01:29
0:01:29
 0:06:33
0:06:33
 0:01:09
0:01:09
 0:02:11
0:02:11
 0:01:09
0:01:09
 0:16:50
0:16:50
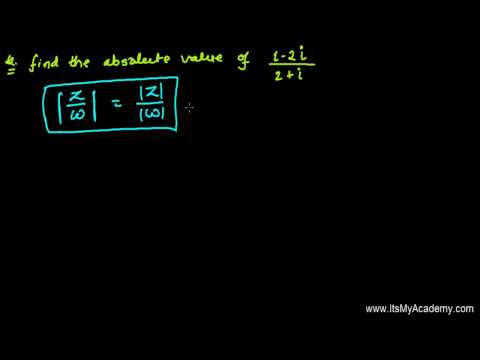 0:01:35
0:01:35
 0:06:22
0:06:22
 0:06:47
0:06:47
 0:00:37
0:00:37
 0:02:52
0:02:52
 0:03:14
0:03:14
 0:02:58
0:02:58
 0:03:11
0:03:11
 0:04:21
0:04:21