filmov
tv
A quartic equation solved in two ways! No quartic formula!!! x^4+4x-1=0

Показать описание
This video is about a quartic equation that can be solved in two ways. No formula!!!
Quartic equations, factoring, Algebra, x^4+4x-1=0
Quartic equations, factoring, Algebra, x^4+4x-1=0
Solving a Quartic Equation with Quadratic Techniques
Solving a Quartic Equation
How To Solve The Quartic Equation?
Solution of the Quartic Equation
A quartic equation, how to solve it? A special way makes it easy | Olympiad Math Practice
The most fun way of solving this quartic equation
How to Solve Quartic Equation in Quadratic Form
How to solve quartic equations. Easy process - Mathsomniac
IOQM Problem Practice | DPP for IOQM | Maths Olympiad for Class 8 9 10 | Online IOQM Coaching
Solving a quartic equation using an unusual idea. An algebraic challenge.
Classwiz How-To: Solving a Quartic Equation
Solving a Quartic Equation
Solving a Quartic Equation with Parameters
How to factor a hard 4th degree polynomial (no rational zero, can't do it by grouping)
A quartic equation solved in two ways! No quartic formula!!! x^4+4x-1=0
How to Solve Quartic Equations
HOW TO SOLVE QUARTIC EQUATION || HOW TO SOLVE FOURTH DEGREE EQUATION || QUARTIC EQUATION PROBLEM
A Non-palindromic Quartic Equation
A quartic equation that can be solved easily. No quartic formula needed.
Quartic Equation Solved in 2 Ways | Daily Math Algebra
How To Solve Quadratic Equations Using The Quadratic Formula
Solve Quartic Equation in Quadratic Form
Quadratic Equation Shortcut Trick | Solve Equation in 5 Seconds
solving equations but they get increasingly awesome
Комментарии
 0:06:57
0:06:57
 0:17:08
0:17:08
 0:10:05
0:10:05
 0:01:22
0:01:22
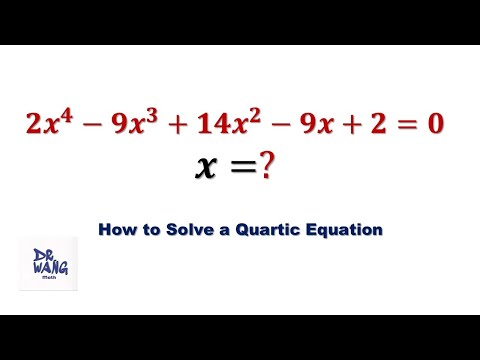 0:06:21
0:06:21
 0:06:39
0:06:39
 0:02:33
0:02:33
 0:09:30
0:09:30
 0:07:24
0:07:24
 0:17:05
0:17:05
 0:01:42
0:01:42
 0:10:13
0:10:13
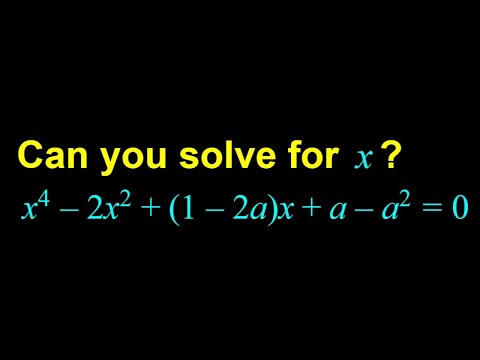 0:11:26
0:11:26
 0:04:55
0:04:55
 0:14:36
0:14:36
 0:00:56
0:00:56
 0:04:42
0:04:42
 0:10:43
0:10:43
 0:05:52
0:05:52
 0:03:58
0:03:58
 0:05:56
0:05:56
 0:04:54
0:04:54
 0:00:30
0:00:30
 0:10:44
0:10:44