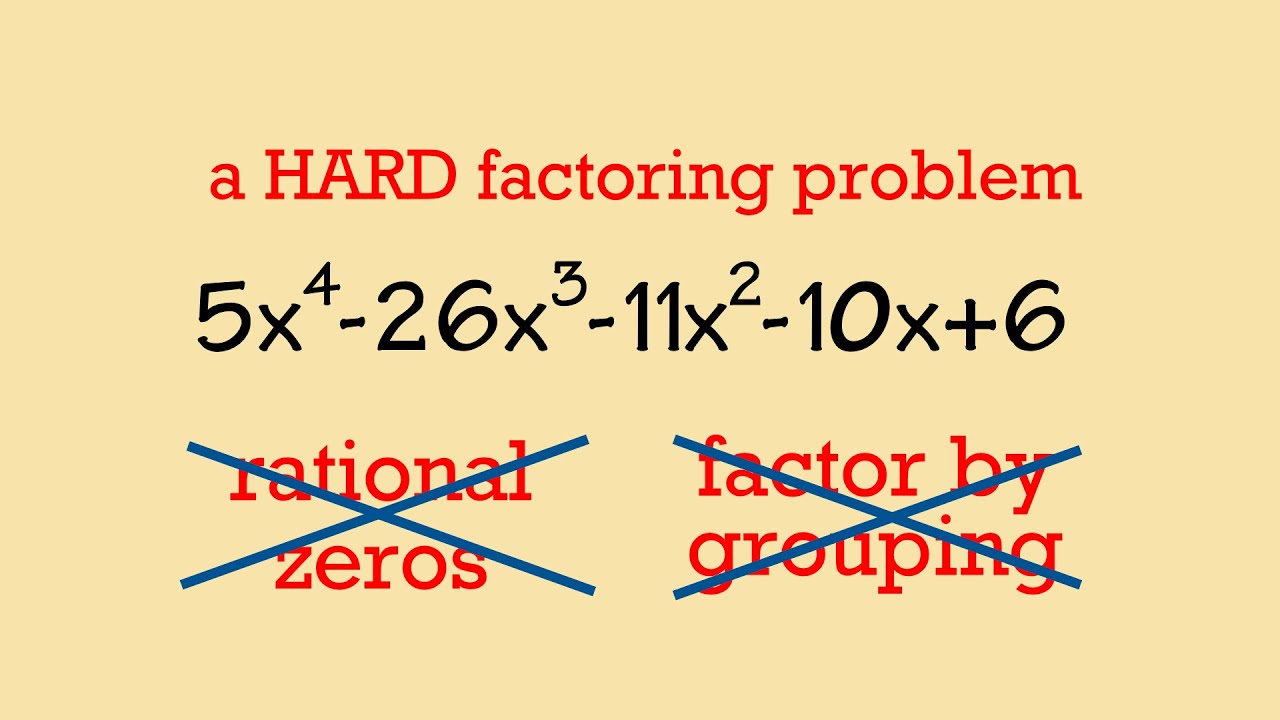
How to factor a hard 4th degree polynomial (no rational zero, can't do it by grouping)

Use "WELCOME10" for 10% off
-----------------------------
-----------------------------
#math #algebra #mathbasics

[bprpmathbasics]

Wanna learn a different method? Factor x^4-4x^3+2x^2-11x+12

[afuyeas]

A quartic can be factored into the product of two quadratics with rational coefficients if and only if a linear combination of the roots of the form a+b-c-d is rational which corresponds to the cubic polynomial having the squares of a+b-c-d, a+c-b-d and a+d-b-c as roots has a rational root that is the square of a rational. For this one the cubic has 1156/25 as a root which is the square of 34/5 so the quartic can be factorized into two quadratics with integer coefficient! If the 3 roots satisfy this property then there are actually three factorizations into two quadratics with integer coefficient which corresponds to the fact that the quartic only has rational roots.

[pauloravazzano]

I'm a big fan of you! I'm 15 years old and you inpires me! Thank you for your channel! Hello from Brazil!

[teelo]

Way too complicated. Heres how to factor 5x^4 + -26x^3 -11x^2 -10x +6: ANSWER: 1(5x^4 + -26x^3 -11x^2 -10x +6)

[kimobrien.]

They are formulas for third and fourth power linear polynomials .Rational root theorem will tell tell you if any degree polynomial has rational roots. In other words if polynomial of degree n Ax^n+Bx^(n-1)+Cx^(n-2) with integer coefficients has at least one rational root it must be a factor (ax+b) with integer coefficients where a is a factor of A and b is a factor of Z. If you can find one real factor you can divide and reduce the degree by one. The binomial theorem and pascal's triangle can help you choose coefficients and substitutions for sums and differences of squares and cubes and make alternating terms disappear. You can also try some complex analysis where you substitute a complex variable a+bi for x and split it into at least two simultaneous equations of real and imaginary parts. Then there is linear algabra wher you can solve sytems of equations using matrices, determinants and inverses.

[NadiehFan]

Here is an alternative method to do this, actually Ferrari's method for solving quartic equations adapted to factoring a quartic polynomial. The quartic polynomial to factor is

5x⁴ − 26x³ − 11x² − 10x + 6

and we are given that this polynomial has no rational zeros, but it does factor into two quadratics with integer coefficients. The task is to find this factorization.

To avoid having to deal with either irrational or fractional coefficients later on we first multiply this polynomial by _four times the coefficient of the leading term_ which is 4·5 = 20. Of course, when we are done we will have to divide the result again by 20 to obtain the factorization of the original quartic. Multiplying by 20 we have

100x⁴ − 520x³ − 220x² − 200x + 120

Now we have 100x⁴ = (10x²)² and 520x³ = 2·(10x²)·26x which is twice the product of 10x² and 26x. Therefore, we can complete the square with respect to the quartic and cubic terms by adding and subtracting (26x)² = 676x² since, applying the identity a² − 2·a·b + b² = (a − b)², we have 100x⁴ − 520x³ + 676x² = (10x²)² − 2·(10x²)·26x + (26x)² = (10x² − 26x)² so we get

(10x² − 26x)² − 676x² − 220x² − 200x + 120
(10x² − 26x)² − 896x² − 200x + 120

(10x² − 26x)² − (896x² + 200x − 120)

We have now written our quartic polynomial as a _difference of two terms_ where the first term is a perfect square but the second term is not. The difference of two terms will remain the same if we add the same quantity to each of the two terms. The idea is to add something to both terms in such a way that the first term will _remain_ a perfect square and the second term will _become_ a perfect square as well. We want to create a difference of two squares because we can then use the difference of two squares identity a² − b² = (a + b)(a − b) to factor our polynomial into two quadratics.

Now, if we take _any_ number λ and add 2λ(10x² − 26x) + λ² = 20λx² − 52λx + λ² to both terms, then the first term will _remain_ a perfect square because, applying the identity a² + 2·a·b + b² = (a + b)², we then have (10x² − 26x)² + 2·(10x² − 26x)·λ + λ² = (10x² − 26x + λ)².

Adding 2λ(10x² − 26x) + λ² = 20λx² − 52λx + λ² to each of the two terms of (10x² − 26x)² − (896x² + 200x − 120) we therefore get

(10x² − 26x + λ)² − ((20λ + 896)x² + (200 − 52λ)x + (λ² − 120))

Since the first term (10x² − 26x + λ)² is a perfect square regardless of the value of λ we are free to choose λ in such a way that the second term (20λ + 896)x² + (200 − 52λ)x + (λ² − 120), which is a quadratic polynomial in x, will also become a perfect square.

A quadratic polynomial ax² + bx + c (a ≠ 0) is a perfect square, that is, the square of a linear polynomial in x, if and only if this quadratic has two coinciding and therefore identical zeros. This is the case if and only if the _discriminant_ b² − 4ac of the quadratic is equal to zero. For our quadratic polynomial (20λ + 896)x² + (200 − 52λ)x + (λ² − 120) we have a = 20λ + 896, b = 200 − 52λ, c = λ² − 120 so this will be a perfect square if and only if λ satisfies

(200 − 52λ)² − 4(20λ + 896)(λ² − 120) = 0

This is a cubic equation in λ but we _do not need to solve this equation_ considering that our quartic polynomial has a factorization into two quadratics with integer coefficients.

If there exists a value of λ that makes (20λ + 896)x² + (200 − 52λ)x + (λ² − 120) the square (mx + n)² = m²x² + 2mnx + n² of a linear polynomial mx + n with _integer_ coefficients m and n, then both the coefficient 20λ + 896 of x² and the constant term λ² − 120 must be squares of integers.

Clearly, if λ² − 120 is to be the square of an integer then λ² must be an integer. But λ itself cannot be irrational because 20λ + 896 also needs to be the square of an integer, and therefore an integer. So, if there exists a value of λ which makes both 20λ + 896 and λ² − 120 the square of an integer, then this value of λ must be an integer.

But how do we go about finding integer values of λ which will make both 20λ + 896 and λ² − 120 the square of an integer? Well, if λ² − 120 is the square of some integer μ for some integer λ, that is, λ² − 120 = μ², then 120 = λ² − μ² must be a difference of two squares of integers. Now, any product of two quantities p and q can be rewritten as a difference of squares since

pq = ((p + q)/2 + (p − q)/2)((p + q)/2 − (p − q)/2) = ((p + q)/2)² − ((p − q)/2)²

Clearly, if (p + q)/2 and (p − q)/2 are integers then their sum p and their difference q must be integers and, moreover, p and q must be integers of like parity if (p + q)/2 and (p − q)/2 are to be integers. Since 120 = 2³·3·5 is even this number evidently cannot be written as the product of two odd integers but we can write 120 as the product of two even integers in several ways, and using the identity above we have

120 = 60·2 = (31 + 29)(31 − 29) = 31² − 29²
120 = 30·4 = (17 + 13)(17 − 13) = 17² − 13²
120 = 20·6 = (13 + 7)(13 − 7) = 13² − 7²
120 = 12·10 = (11 + 1)(11 − 1) = 11² − 1²

which exhausts all possibilities of writing 120 as a difference of two squares of integers.

Evidently if λ² − 120 is the square of some integer μ for some integer λ and therefore 120 = λ² − μ² then λ must be one of the numbers ±11, ±13, ±17, ±31 so we need to test at most eight integer values of λ to see if this will make 20λ + 896 a square as well.

Starting with λ = 11 an λ = −11 we have 20λ + 896 = 20·11 + 896 = 1116 and 20·(−11) + 896 = 776 which are not squares of integers since 33² = 1089 < 1116 < 34² = 1156 and 27² = 729 < 776 < 28² = 784. But with λ = 13 we have 20λ + 896 = 20·13 + 896 = 1156 = 34², as required.

With λ = 13 our polynomial

(10x² − 26x + λ)² − ((20λ + 896)x² + (200 − 52λ)x + (λ² − 120))

becomes

(10x² − 26x + 13)² − (1156x² − 476x + 49)

which is

(10x² − 26x + 13)² − (34x − 7)²

and we have converted our quartic polynomial into a difference of two squares. Using the difference of two squares identity a² − b² = (a + b)(a − b) this can be written as

(10x² − 26x + 13 + 34x − 7)(10x² − 26x + 13 − 34x + 7)

which is

(10x² + 8x + 6)(10x² − 60x + 20)

and we have factored our quartic into two quadratics. Now note that the first quadratic has a factor 2 and that the second quadratic has a factor 10 we can take out to get

20(5x² + 4x + 3)(x² − 6x + 2)

and since we started out by multiplying the polynomial to factor by 20 this means that the original quartic polynomial

5x⁴ − 26x³ − 11x² − 10x + 6

factors as

(5x² + 4x + 3)(x² − 6x + 2)

[knutthompson]

There is absolutely a quartic discriminant. Every polynomial has a discriminant. But It is complicated and alone won’t tell you if the quartic in factorable. You CAN tell using the resolvent if you turn the quartic into a depressed quartic (without a cubic term). This is not super simple to do, but can be done.

[giovannipaoloboyd-trevisan]

Thank you for making maths so enjoyable!!!

[Sneha...patter]

Best method out of all I have seen before❤❤

[wes]

If I set this expression to zero and note that there are two sign changes, there should be at least two real roots. I can use fixed-point iteration or Newton-Raphson iteration to obtain numerical values for these two roots. Then synthetically divide to obtain a quadratic, from which the remaining two roots may be obtained. Using these four roots I can then obtain the desired factors of the original expression if I really needed them.

[KippoKupo]

THANK YOU so many people are cowards like Brian Mclogan and just use a leading coefficient of 1

[SimpdePaint]

I had a class about factor polinomials like this (others too) and taught to my a class we have another way which is like factoring another polynomial.

You reach a polynomial that its quadratic term is close to that polynomial then factor. (????)

[MsAbdullah]

Is there another method to reach the answer

[jashwanthkumar]

bro how did you write last step of the ans as product of factors

[fahadnothing]

Can u factorize it plssss
x³+x+1
It will help me so much( I don't even sure if this is possible)

[m.rafay]

Why didn't you use synthetic division?

[georgecaplin]

I’m genuinely not trying to insult anyone, it’s only a bit of roasting, but I love how these mathematicians can do integrals and trigonometry without looking, then ask them to do a (fairly) simple multiplication, they freeze.

[MrBeeastFan]

What is the -11x^2 for if the answer would be in a form that does not use it in any way?

[MrBeeastFan]

Doesnt he have to factor the x^2 terms too by factoring or quadratic formula at least for a complete factor?

[antoniusnies-komponistpian]

I love when he says "Ladies and Gentlemen"