solving equations but they get increasingly awesome

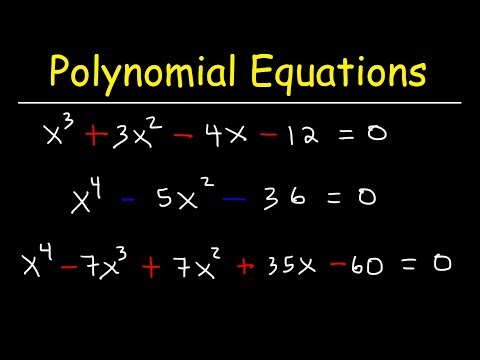
Solving polynomial equations but they get increasingly more awesome. We will also be solving them with different methods such as using the quadratic formula, factoring by grouping, a special way to complete the square, and also utilizing the 6th root of unity.

0:00 5 levels of a polynomial equation, from good to AWESOME!
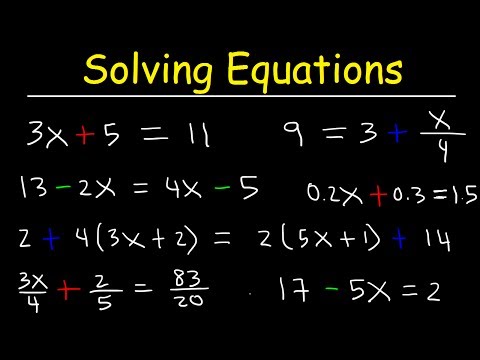
0:3 linear equation x+1=0
0:16 quadratic equation x^2+x+1=0
1:36 cubic equation x^3+x^2+x+1=0
2:41 quadratic equation x^4+x^3+x^2+x+1=0
7:01 quintic equation x^5+x^4+x^3+x^2+x+1=0

10% off with the code "WELCOME10"
----------------------------------------
***Thanks to ALL my lovely patrons for supporting my channel and believing in what I do***
AP-IP Ben Delo Marcelo Silva Ehud Ezra 3blue1brown Joseph DeStefano
Mark Mann Philippe Zivan Sussholz AlkanKondo89 Adam Quentin Colley
Gary Tugan Stephen Stofka Alex Dodge Gary Huntress Alison Hansel
Delton Ding Klemens Christopher Ursich buda Vincent Poirier Toma Kolev
Tibees Bob Maxell A.B.C Cristian Navarro Jan Bormans Galios Theorist
Robert Sundling Stuart Wurtman Nick S William O'Corrigan Ron Jensen
Patapom Daniel Kahn Lea Denise James Steven Ridgway Jason Bucata
Mirko Schultz xeioex Jean-Manuel Izaret Jason Clement robert huff
Julian Moik Hiu Fung Lam Ronald Bryant Jan Řehák Robert Toltowicz
Angel Marchev, Jr. Antonio Luiz Brandao SquadriWilliam Laderer Natasha Caron Yevonnael Andrew Angel Marchev Sam Padilla ScienceBro Ryan Bingham
Papa Fassi Hoang Nguyen Arun Iyengar Michael Miller Sandun Panthangi
Skorj Olafsen Riley Faison Rolf Waefler Andrew Jack Ingham P Dwag Jason Kevin Davis Franco Tejero Klasseh Khornate Richard Payne Witek Mozga Brandon Smith Jan Lukas Kiermeyer Ralph Sato Kischel Nair Carsten Milkau Keith Kevelson Christoph Hipp Witness Forest Roberts Abd-alijaleel Laraki Anthony Bruent-Bessette Samuel Gronwold Tyler Bennett christopher careta Troy R Katy Lap C Niltiac, Stealer of Souls Jon Daivd R meh Tom Noa Overloop Jude Khine R3factor. Jasmine Soni L
----------------------------------------

[redsurfer_]

We're no strangers to love
You know the rules and so do I
A full commitment's what I'm thinking of
You wouldn't get this from any other guy

I just wanna tell you how I'm feeling
Gotta make you understand

Never gonna give you up
Never gonna let you down
Never gonna run around and desert you
Never gonna make you cry
Never gonna say goodbye
Never gonna tell a lie and hurt you

We've known each other for so long
Your heart's been aching, but
You're too shy to say it
Inside, we both know what's been going on
We know the game and we're gonna play it

And if you ask me how I'm feeling
Don't tell me you're too blind to see

Never gonna give you up
Never gonna let you down
Never gonna run around and desert you
Never gonna make you cry
Never gonna say goodbye
Never gonna tell a lie and hurt you

Never gonna give you up
Never gonna let you down
Never gonna run around and desert you
Never gonna make you cry
Never gonna say goodbye
Never gonna tell a lie and hurt you

(Ooh, give you up)
(Ooh, give you up)
Never gonna give, never gonna give
(Give you up)
Never gonna give, never gonna give
(Give you up)

We've known each other for so long
Your heart's been aching, but
You're too shy to say it
Inside, we both know what's been going on
We know the game and we're gonna play it

I just wanna tell you how I'm feeling
Gotta make you understand

Never gonna give you up
Never gonna let you down
Never gonna run around and desert you
Never gonna make you cry
Never gonna say goodbye
Never gonna tell a lie and hurt you

Never gonna give you up
Never gonna let you down
Never gonna run around and desert you
Never gonna make you cry
Never gonna say goodbye
Never gonna tell a lie and hurt you

Never gonna give you up
Never gonna let you down
Never gonna run around and desert you
Never gonna make you cry
Never gonna say goodbye
Never gonna tell a lie and hurt you

[hellbowe.]

omg how he cleaned the board with his will at 5:24 that's so nice

[mattchu]

i can never get over how he switches between markers so effortlessly

[mith_jain_here]

In the quintic equation, the polynomial can also be factorised as (x³ + 1)(x² + x + 1) = 0 and these two are very simple to solve

[davidblauyoutube]

This would be a great way to introduce the cyclotomic polynomials.

[novidsonmychanneljustcomme]

Actually you can generalize the method of the quintic equation for all degrees of that kind of equation:
x^n+x^(n-1)+...+x+1=0 | *(x-1)/=0
=> x^(n+1)-1=0
And now calculate all roots of unity for k=1, ..., n (and without k=0 of course).

[anatolykatyshev]

Why it is difficult? n-th equation is : (X^(n+1)-1)/(x-1)=0
So we need to find all roots of X^(n+1)=1, except x=1., or to find all roots of power n of 1. It could be done easily in exponential form on complex plane. cos(2*pi*k/n)+i*sin(2*pi*k/n) where 0<k<n

[pseudo_goose]

Beautiful! The solutions to each equation were all the (n+1)'th roots of unity (except 1). A little hard to see the pattern, but the last one revealed it spectacularly.

[胡書瑋-os]

In Taiwan, Asia, we have to complete the above knowledge in three years of high school. Although mathematics is very interesting, the pressure of the exam often makes me breathless

[velmurugan-hemr]

I really wonder to see how you handle different colour pens in a single hand

[siavashghazisaidi]

One of the last roots of the last equation is -1, since it is an inverse relationship with an odd degree. The resulting quartic equation can be solved by using the method of solving inverse relations.

[josevidal]

Also, x⁴+x³+x²+x+1 can be factored as (x²+φx+1)(x²+Φx+1); where φ and Φ are the Golden ratios, (1±√5)/2, the solutions to x²-x-1=0

[Hazza]

Each polynomial is actually just a geometric series, the reason he multiplied by (x-1) for the Quintic is because of the geometric sum formula. Sum x^r from r=0 to r=n equals (x^(n+1)-1)/(x-1). So infact each and every one of this polynomials could have been multiplied by (x-1) and then solved very easily by getting the nth root of unity. In general a polynomial sum x^r, r=0 to n, i.e. x^n + x^n-1 + ….. + x +1 = 0 can be multiplied by (x-1) to obtain x^n+1 -1 =0 to then get the nth roots of unity

[Данила-Шашков]

The last equation can be solved by another way:
x⁴(x+1)+x²(x+1)+x+1=0
(x+1) (x⁴+x²+1)=0
x1=-1
let t=x²
t²+t+1=0
this is the second equation, that we solved.
But solving by using Euler's formula is beautiful

[gab_]

2:48 Although it's obvious, you should briefly mention that x cannot be equals to 0

[racemaniak]

Now for Level 0:
1=0
Try to solve that one

[marcocartaerainnocente]

0:17 wouldn’t it be better to use the formula b square - 4 (a)(c)?

[Pearking_]

I barely understand this but it's still fun to learn something. I love your videos and they are so well done.

[IamExeller]

The first precious knowledge I earned... From your educative channel...

Is...

2:40 - 7:00 Quartic = Quadratic

[weasel]

this is so cool omg i noticed how we got some interesting roots of unity in the x^3 + x^2 + x + 1 case thats such a beautiful connection!!!