filmov
tv
An Intriguing Rational Equation Challenge You Can't Miss!
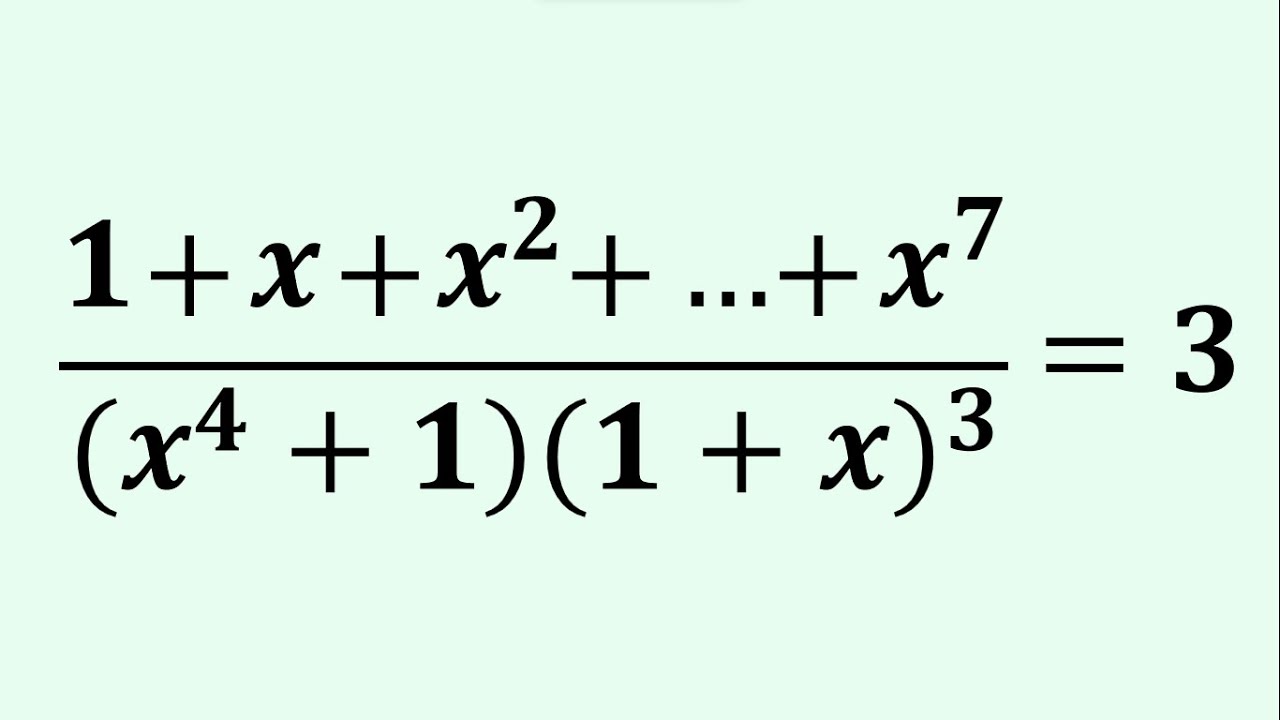
Показать описание
An Intriguing Rational Equation Challenge You Can't Miss!
Dive into the world of mathematical intrigue with our latest video – 'An Intriguing Rational Equation Challenge You Can't Miss!' 🌐🧮 Join us on this journey as we unravel the complexities of a fascinating rational equation. Are you up for the challenge? Sharpen your problem-solving skills and explore the beauty of mathematical puzzles. Don't miss out on this opportunity to stretch your mathematical muscles! 🤔💡 #MathChallenge #RationalEquation #ProblemSolving #MathEnigma #EquationChallenge #Mathematics #PuzzleSolving #CriticalThinking #ChallengeAccepted #MathAdventure #algebra #maths #math
Topics covered:
Algebra Challenge
Rational Equation
How to solve Rational Equations?
Math Olympiad
Geometric progression
Sum in geometric series
Algebra
Math Tricks
Algebraic identities
Algebraic manipulations
Real Solutions
Quadratic formula
Quadratic equations
Domain
Math Tutorial
Algebraic Challenging Equations
Math Olympiad Preparation
📚 Timestamps:
0:00 - Introduction
0:36 - Domain
2:14 - Geometric progression
4:10 - Algebraic manipulations
6:18 - Algebraic identities
9:33 - Quadratic equation
9:52 - Quadratic formula
10:24 - Real solutions
Don't forget to like, share, and subscribe to stay updated on more thrilling math challenges and solutions! Challenge your friends to see who can solve it faster.
We'd love to hear from you! Did you manage to solve the equation? What other math problems would you like us to cover? Let us know in the comments below!
🎓 Happy learning, and see you in the next video! 🎉
Thanks for Watching !!
@infyGyan
Dive into the world of mathematical intrigue with our latest video – 'An Intriguing Rational Equation Challenge You Can't Miss!' 🌐🧮 Join us on this journey as we unravel the complexities of a fascinating rational equation. Are you up for the challenge? Sharpen your problem-solving skills and explore the beauty of mathematical puzzles. Don't miss out on this opportunity to stretch your mathematical muscles! 🤔💡 #MathChallenge #RationalEquation #ProblemSolving #MathEnigma #EquationChallenge #Mathematics #PuzzleSolving #CriticalThinking #ChallengeAccepted #MathAdventure #algebra #maths #math
Topics covered:
Algebra Challenge
Rational Equation
How to solve Rational Equations?
Math Olympiad
Geometric progression
Sum in geometric series
Algebra
Math Tricks
Algebraic identities
Algebraic manipulations
Real Solutions
Quadratic formula
Quadratic equations
Domain
Math Tutorial
Algebraic Challenging Equations
Math Olympiad Preparation
📚 Timestamps:
0:00 - Introduction
0:36 - Domain
2:14 - Geometric progression
4:10 - Algebraic manipulations
6:18 - Algebraic identities
9:33 - Quadratic equation
9:52 - Quadratic formula
10:24 - Real solutions
Don't forget to like, share, and subscribe to stay updated on more thrilling math challenges and solutions! Challenge your friends to see who can solve it faster.
We'd love to hear from you! Did you manage to solve the equation? What other math problems would you like us to cover? Let us know in the comments below!
🎓 Happy learning, and see you in the next video! 🎉
Thanks for Watching !!
@infyGyan
Комментарии
 0:10:36
0:10:36
 0:14:55
0:14:55
 0:18:01
0:18:01
 0:15:54
0:15:54
 0:10:24
0:10:24
 0:13:14
0:13:14
 0:13:14
0:13:14
 0:11:34
0:11:34
 0:09:32
0:09:32
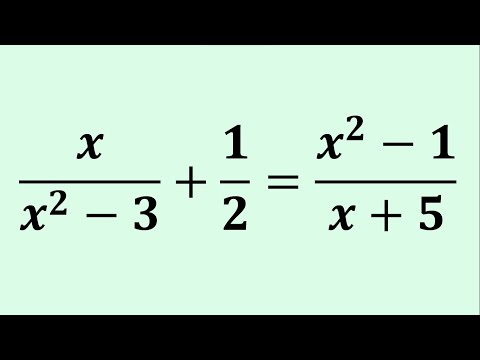 0:13:19
0:13:19
 0:09:18
0:09:18
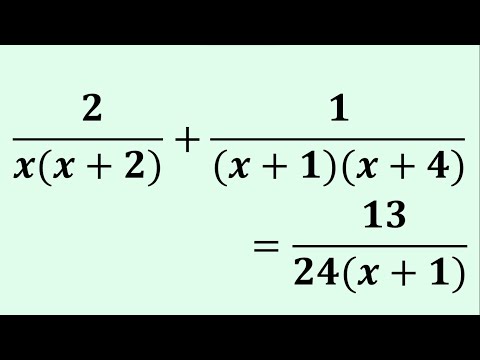 0:12:53
0:12:53
 0:10:10
0:10:10
 0:10:44
0:10:44
 0:14:12
0:14:12
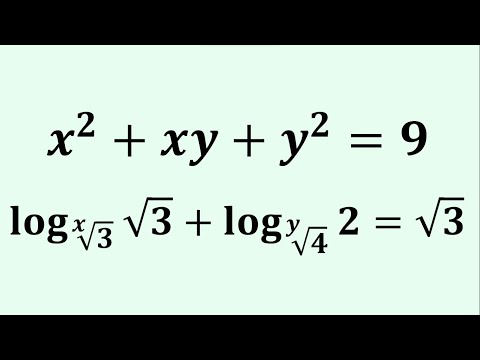 0:08:57
0:08:57
 0:10:42
0:10:42
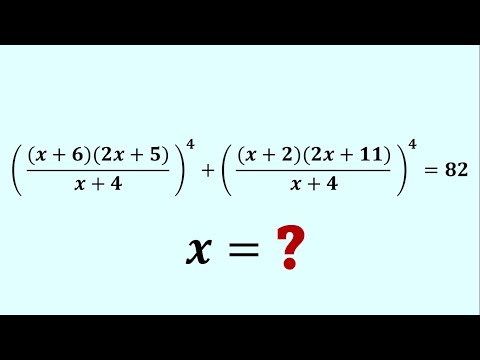 0:13:35
0:13:35
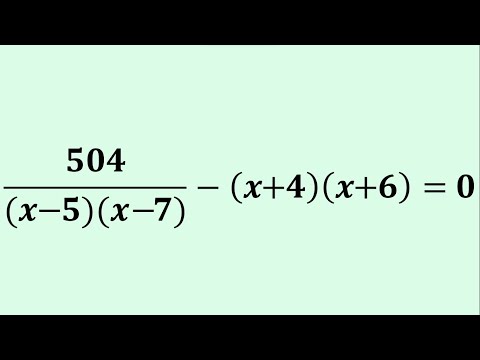 0:12:05
0:12:05
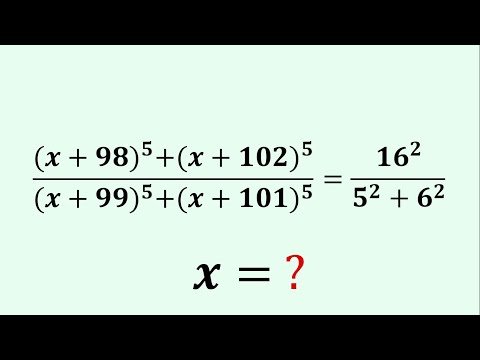 0:13:54
0:13:54
 0:09:12
0:09:12
 0:00:12
0:00:12
 0:00:57
0:00:57
 0:11:03
0:11:03