filmov
tv
Proof of the Derivative of e^x ( Definition of Derivative)

Показать описание
Using the definition of derivative to prove the derivative of e^x.
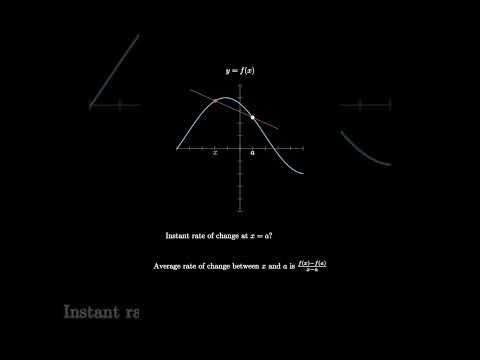
Visual Derivative Definition!
Proof of the derivative of sin(x) | Derivatives introduction | AP Calculus AB | Khan Academy
Visual derivative of x squared
Visualizing the derivative of sin(x)
Proof of the derivative of e^x: A Step-by-Step Proof and Explanation
Proof of the derivative of cos(x) | Derivative rules | AP Calculus AB | Khan Academy
Derivative as a concept | Derivatives introduction | AP Calculus AB | Khan Academy
Proof of the Formula for the Derivative of a^x
STATE And Proof Fundamental Theorem Of Integral Calculus By Pk SIR
Proofs of derivatives of ln(x) and e^x | Taking derivatives | Differential Calculus | Khan Academy
Proof of the Derivative of the Exponential Functions
Proof of the derivative of tanx: A Step-by-Step Proof and Explanation
Proof: The derivative of __ is __ | Advanced derivatives | AP Calculus AB | Khan Academy
Definition of the Derivative
What is a Derivative? Deriving the Power Rule
Exponential derivative visual
Shortcut For Derivative of x^x
Proof of the derivative of cosx: A Step-by-Step Proof and Explanation
Proof: Derivative of Constant
Proof: the derivative of ln(x) is 1/x | Advanced derivatives | AP Calculus AB | Khan Academy
Proof: Derivative of sin(x) = cos(x) by First Principles
Chain rule proof | Derivative rules | AP Calculus AB | Khan Academy
Proof of the Derivative of Sinx
AP Calculus - Proof Definition of the Definition of a Derivative
Комментарии
 0:01:00
0:01:00
 0:05:52
0:05:52
 0:00:58
0:00:58
 0:00:59
0:00:59
 0:03:51
0:03:51
 0:03:18
0:03:18
 0:07:16
0:07:16
 0:03:01
0:03:01
 1:04:19
1:04:19
 0:12:27
0:12:27
 0:02:35
0:02:35
 0:06:09
0:06:09
 0:08:39
0:08:39
 0:23:31
0:23:31
 0:10:05
0:10:05
 0:00:57
0:00:57
 0:00:52
0:00:52
 0:04:53
0:04:53
 0:02:39
0:02:39
 0:08:08
0:08:08
 0:14:40
0:14:40
 0:05:39
0:05:39
 0:04:57
0:04:57
 0:07:26
0:07:26