filmov
tv
Exponential derivative visual

Показать описание
A visual of the derivative of f(x)=e^x. We show how to think about the derivative of a function visually.
#manim #calculus #derivatives #derivative #tangentline #slope #parabola #mathvideo #mathshorts #math #visualmath #graph #exponential #linearapproximation
#manim #calculus #derivatives #derivative #tangentline #slope #parabola #mathvideo #mathshorts #math #visualmath #graph #exponential #linearapproximation
Exponential derivative visual
Differentiating Exponential Functions (1 of 3: A Visual Argument for the Derivative)
Visual derivative of x squared
Calculus of Exponential Functions (1 of 4: Considering derivatives visually)
What's so special about Euler's number e? | Chapter 5, Essence of calculus
How to Graph Exponential Functions
Introducing Calculus of Exponential Functions (1 of 6: Considering other functions)
How REAL Men Integrate Functions
What is e and ln(x)? (Euler's Number and The Natural Logarithm)
Understanding Exponential Functions and their Gradients: Intuitive Approach
Exponential Derivative ?!
Ableitung e-Funktion mit Kettenregel | Visual X
When mathematicians get bored (ep1)
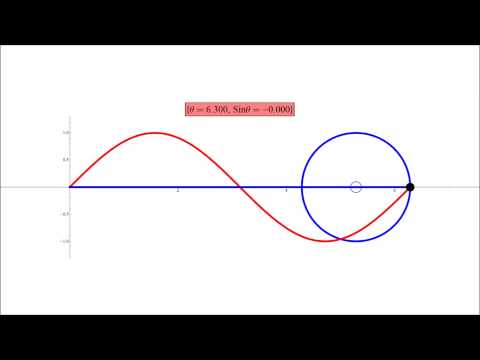
Sine Curve and the Unit Circle
5 simple unsolvable equations
Exponential functions differentiation intro | Advanced derivatives | AP Calculus AB | Khan Academy
Derivatives of #exponential #functions | #derivative
e^(iπ) in 3.14 minutes, using dynamics | DE5
Difference Between Partial and Total Derivative
Higher order derivatives | Chapter 10, Essence of calculus
exponential function | infinite series #calculus #math #animation #learn #study
Derivative of exponential functions #shorts #maths
Connecting f, f', and f'' graphically | AP Calculus AB | Khan Academy
Differentiating Exponential Functions (1 of 2: Reviewing simple derivatives)
Комментарии
 0:00:57
0:00:57
 0:10:08
0:10:08
 0:00:58
0:00:58
 0:09:14
0:09:14
 0:13:50
0:13:50
 0:00:40
0:00:40
 0:05:46
0:05:46
 0:00:35
0:00:35
 0:12:02
0:12:02
 0:08:10
0:08:10
 0:25:54
0:25:54
 0:06:38
0:06:38
 0:00:37
0:00:37
 0:00:27
0:00:27
 0:00:50
0:00:50
 0:05:24
0:05:24
 0:00:12
0:00:12
 0:04:08
0:04:08
 0:01:44
0:01:44
 0:05:39
0:05:39
 0:00:12
0:00:12
 0:00:25
0:00:25
 0:05:36
0:05:36
 0:11:43
0:11:43