filmov
tv
Proof: Derivative of sin(x) = cos(x) by First Principles
Показать описание
In this video, we prove that the derivative of sin(x) equals cos(x) by the very definition of the derivative, which is:
df/dx = f'(x) = lim_(h approaches 0) f(x + h) - f(x) / h
Thus:
d/dx [sin(x)] = lim_(h approaches 0) sin(x + h) - sin(x) / h
Thanks for watching. Please give me a "thumbs up" if you have found this video helpful.
Please ask me a maths question by commenting below and I will try to help you in future videos.
df/dx = f'(x) = lim_(h approaches 0) f(x + h) - f(x) / h
Thus:
d/dx [sin(x)] = lim_(h approaches 0) sin(x + h) - sin(x) / h
Thanks for watching. Please give me a "thumbs up" if you have found this video helpful.
Please ask me a maths question by commenting below and I will try to help you in future videos.
0:05:52
Proof of the derivative of sin(x) | Derivatives introduction | AP Calculus AB | Khan Academy
0:09:18
Derivative of sin(x) and cos(x), PROOF
0:04:57
Proof of the Derivative of Sinx
0:14:40
Proof: Derivative of sin(x) = cos(x) by First Principles
0:06:06
Proof of the derivative of sinx: A Step-by-Step Proof and Explanation
0:08:11
Proof - Derivative of Sin(x) | JK Math
0:09:39
Derivative of sin(x) from First Principles
0:03:08
Visual Calculus: Derivative of sin(θ) is cos(θ)
0:21:25
BSc 1st sem Math || Ch- Successive Differentiation || By G K Mahaur || Differential Calculus L -8 ✍️...
0:03:50
Derivative of sinx Proof | Differentiation of sinx is cosx Proof
0:09:39
Proof: Derivative of Sin is Cos (Version 2)
0:03:41
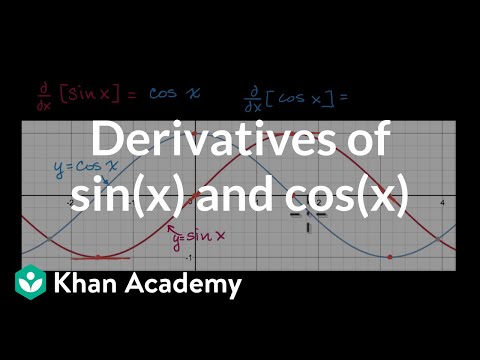
Derivatives of sin(x) and cos(x) | Derivative rules | AP Calculus AB | Khan Academy
0:19:53
Derivatives of ALL trig functions (proofs!)
0:07:49
Derivative of Sine X = Cos X || Differentiate Sin X || Proof in Calculus Math
0:10:46
The derivative proof for sin x
0:01:37
Derivative of sin(x) | Animation | mathocube |
0:07:32
derivative of sin(x) by using the definition of derivative
0:05:20
Derivative of Sin(x) Proof (Using the Limit Definition)
0:17:08
Establishing the Derivatives of sin x, cos x & tan x
0:11:54
The most important limit in Calculus // Geometric Proof & Applications
0:09:16
Limit of sin(x)/x as x approaches 0 | Derivative rules | AP Calculus AB | Khan Academy
0:03:47
The PROOF that the Derivative of sin(x) is cos(x)!!! | Limit Definition of Derivatives
0:05:40
Proof of the derivative of inverse sin x: A Step-by-Step Proof and Explanation
0:05:34
Graphical Proof of the Derivative of sin(x)
Комментарии