filmov
tv
A nice square root problem | Learn Math Olympiad question solution
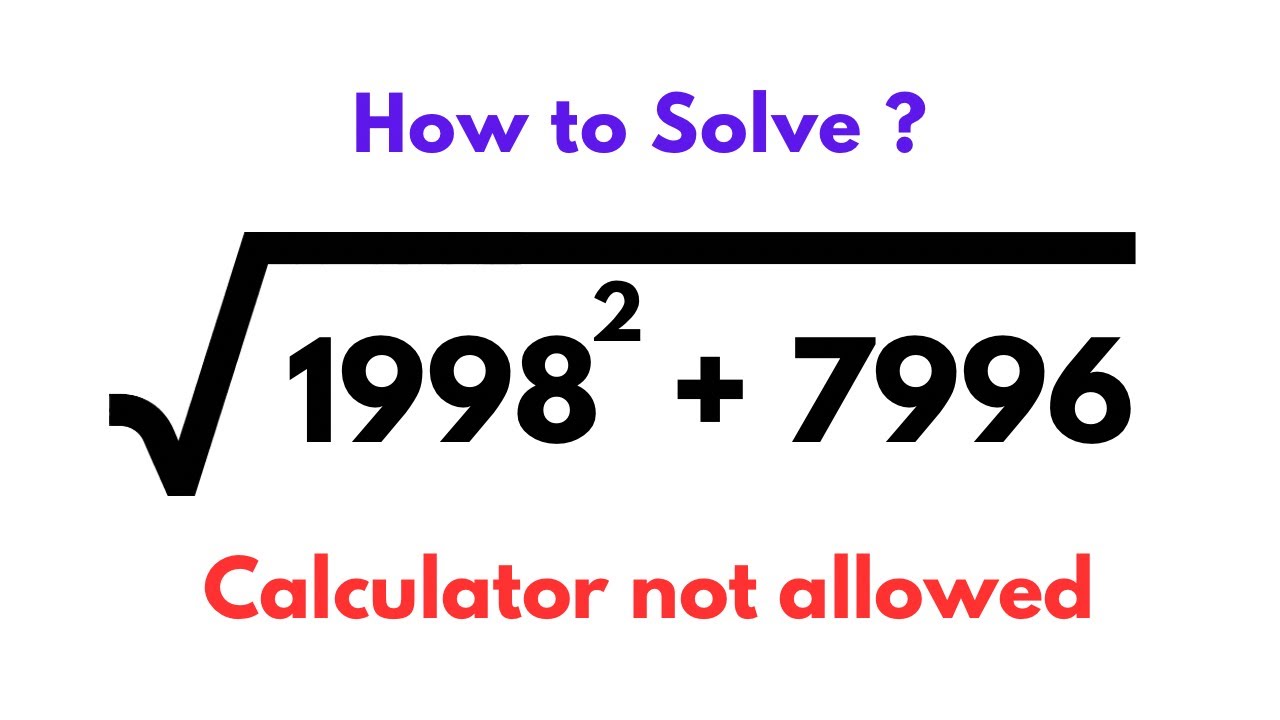
Показать описание
Learn the easy method to solve square root related problems. This video tells step wise how to solve a tricky square root question. If you like the video, please like & subscribe the channel.
#math #mathematics #algebra #matholympiadquestion #mathstricks #mathsolympiad #mathlover #calculus #learnmaths #mathstrick #mathematicsanalysis #mathsclass #equation #quadraticequation #squareroot #squareroots
#math #mathematics #algebra #matholympiadquestion #mathstricks #mathsolympiad #mathlover #calculus #learnmaths #mathstrick #mathematicsanalysis #mathsclass #equation #quadraticequation #squareroot #squareroots
Nice Square Root Math Simplification |Find the Value of X
why sqrt(36) is just positive 6
A Nice Square Root Algebra Problem
Solving a tricky SAT square root problem
What are Square Roots? | Math with Mr. J
A Proof That The Square Root of Two Is Irrational
Nice Square Root Math Simplification | How many solutions?
No more confusion on square root
How to solve square root problem 🙂#maths
Math Antics - Exponents and Square Roots
A Wonderful Math Problem. Square root i + Square root -i =?
A Nice Square Root Problem | Math Olympiad Preparation
Nice square root math problem
A DIFFICULT SQUARE ROOT Problem Simplified
How to Approximate Square Root of a Number
How To Calculate Square Roots - Numerals That Changed Math Forever
Square root problem
Nice square root problem
Simplifying Square Roots | Math with Mr. J
Square root in 3 seconds - math trick
Germany | A Tricky Maths Olympiad Question | Square Roots |
How To Simplify Square Roots
China |A Nice Square root Problem | How to Simplify A Radical Problem | Calculator Not Allowed |
Approximating a Square Root Without a Calculator
Комментарии
 0:09:55
0:09:55
 0:01:42
0:01:42
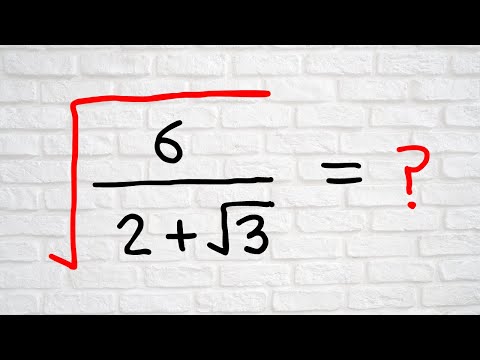 0:05:16
0:05:16
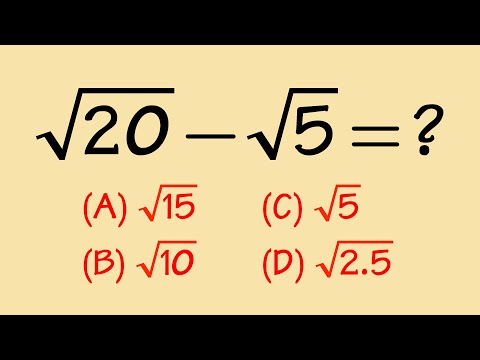 0:04:33
0:04:33
 0:04:00
0:04:00
 0:17:22
0:17:22
 0:11:28
0:11:28
 0:01:02
0:01:02
 0:00:18
0:00:18
 0:11:09
0:11:09
 0:03:55
0:03:55
 0:04:42
0:04:42
 0:00:37
0:00:37
 0:10:34
0:10:34
 0:00:52
0:00:52
 0:10:16
0:10:16
 0:00:32
0:00:32
 0:00:34
0:00:34
 0:07:44
0:07:44
 0:09:46
0:09:46
 0:08:35
0:08:35
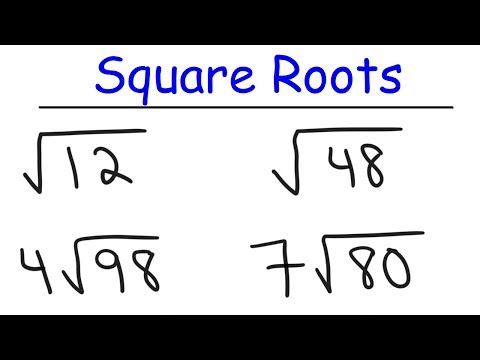 0:10:46
0:10:46
 0:11:42
0:11:42
 0:03:40
0:03:40