filmov
tv
No more confusion on square root

Показать описание
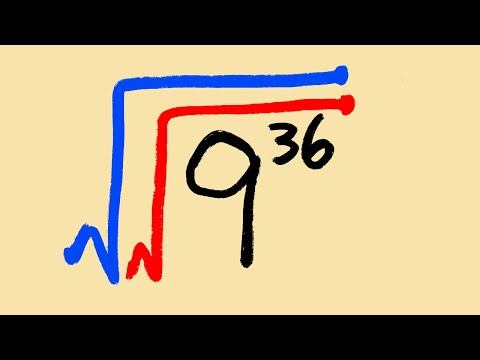
We will talk about why the square root (when you use the square root symbol) of 36 is just 6. We will also talk about the common mistake that people get mixed up between evaluating sqrt(36) and solving x^2=36. Subscribe to @bprpmathbasics for more math basics that you need for your algebra class!
-----------------------------
-----------------------------
#math #algebra #mathbasics
-----------------------------
-----------------------------
#math #algebra #mathbasics
No more confusion on square root
Can we really just cancel the square root & the square? BE CAREFUL!
No more confusion! Is it +5 or - 5? #maths #mathematics #square #squares #explore #calculate
why sqrt(36) is just positive 6
No more confusion on square root
Addressing The Square-Root Confusion
No More Confusion on Square Root🤓 #algebra #maths #squareroot #shorts #trending #youtube #shortsfeed...
Don't get confused with square roots on test day! #shorts
NO more confusion to take LCM and GCF
fun square root problem (nested square root tho!)
Most math students don’t understand this about square roots!
No More Extraneous Roots CONFUSION – Radical Equations
Mathematics Operations simplified | No more confusion with mathematics signs
this edit though 🔥|| the incredibles 2 #shorts
A Better Version of the Square Root Symbol... #shorts
99% of Math Students Get This STEP CONFUSED!
Simplifying Square Roots: Correcting a Common Confusion
No more confusion on square root and principal square root ( In Bengali) @Susovanmath
Square Root | Confusion Explained | Radicals and Exponents
16 Golden Rules for Crafting Powerful Research Hypotheses - No More Confusion - Complete Guide
my brain is square and im often confused
Confused with Radical Expressions? Let’s solve this problem…
Math League Problem | No More Confusion On fraction problem | A-Maths
No more confusion with US and UK terms!
Комментарии
 0:01:02
0:01:02
 0:02:54
0:02:54
 0:01:29
0:01:29
 0:01:42
0:01:42
 0:00:36
0:00:36
 0:14:32
0:14:32
 0:01:44
0:01:44
 0:00:33
0:00:33
 0:15:39
0:15:39
 0:03:27
0:03:27
 0:09:57
0:09:57
 0:18:33
0:18:33
 0:00:45
0:00:45
 0:00:08
0:00:08
 0:00:59
0:00:59
 0:18:32
0:18:32
 0:06:09
0:06:09
 0:13:21
0:13:21
 0:18:09
0:18:09
 0:22:23
0:22:23
 0:05:35
0:05:35
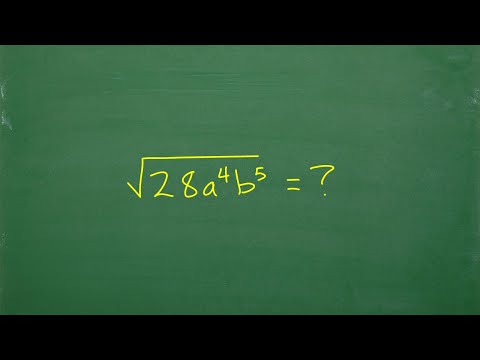 0:15:53
0:15:53
 0:04:01
0:04:01
 0:00:10
0:00:10