filmov
tv
The Algebra Word Problem That Stumps Everyone – Can You Solve It?

Показать описание
Help with Middle and High School Math
Test Prep for High School Math, College Math, Teacher Certification Math and More!
Popular Math Courses:
Math Foundations
Math Skills Rebuilder Course:
Pre-Algebra
Algebra
Geometry
Algebra 2
Pre-Calculus
• MIDDLE & HIGH SCHOOL MATH
• HOMESCHOOL MATH
• COLLEGE MATH
• TEST PREP MATH
• TEACHER CERTIFICATION TEST MATH
The Algebra Word Problem That Stumps Everyone – Can You Solve It?
😊Solving word problems in Algebra (math test)😊
Algebraic Word Problems
Translating Words To Algebraic Expressions Explained!
Solving Word Problems Using Algebra
Solve Algebra Word Problems
Let’s Solve This Algebra Word Problem…Step-By-Step…
[March SAT Math] Weird Trick To Solve All Word Problems - Raise Your SAT SCORE!
Mathematics: Word Problem ( Part3)(SS1)
How To Solve Math Percentage Word Problems | Algebra
How to write algebraic expressions from word problems | 6th grade | Khan Academy
Solving Word Problems in Algebra : - 4 Videos-in-1 Lesson dealing with Translation of Expressions
Algebra Word Problems Into Equations - Basic Introduction
Algebra SYSTEM WORD PROBLEM – Let’s solve it step-by-step...
Algebra 1 Level WORD Problem – Need to Know to PASS…
Father and Son Age Word Problem with two variables Linear System
Time and Work Problems - Shortcuts and Tricks
Using Algebra To Solve Word Problems
🧮 Jessie Solving Word Problems | Math Video Lesson | ABCmouse 🎲🤓
Systems of equations word problems example 1 | Algebra I | Khan Academy
Linear equation word problem | Linear equations | Algebra I | Khan Academy
Word Problems—Add or Subtract? | MightyOwl Math | Kindergarten
How to Solve Distance Rate Time Problems
Algebra AGE WORD PROBLEM – Let’s solve it step-by-step...
Комментарии
 0:11:01
0:11:01
 0:15:55
0:15:55
 0:05:38
0:05:38
 0:10:43
0:10:43
 0:11:14
0:11:14
 0:25:55
0:25:55
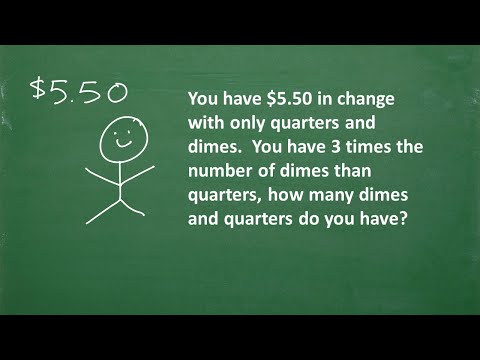 0:11:28
0:11:28
![[March SAT Math]](https://i.ytimg.com/vi/vhMRoD7uA4w/hqdefault.jpg) 0:15:23
0:15:23
 0:07:50
0:07:50
 0:05:42
0:05:42
 0:04:41
0:04:41
 0:13:31
0:13:31
 0:22:35
0:22:35
 0:23:43
0:23:43
 0:15:40
0:15:40
 0:05:44
0:05:44
 0:33:55
0:33:55
 0:14:15
0:14:15
 0:04:26
0:04:26
 0:05:51
0:05:51
 0:05:59
0:05:59
 0:06:54
0:06:54
 0:10:31
0:10:31
 0:21:35
0:21:35