Dimension and basis for solution space of a homogeneous system of equations Example 1

VS11
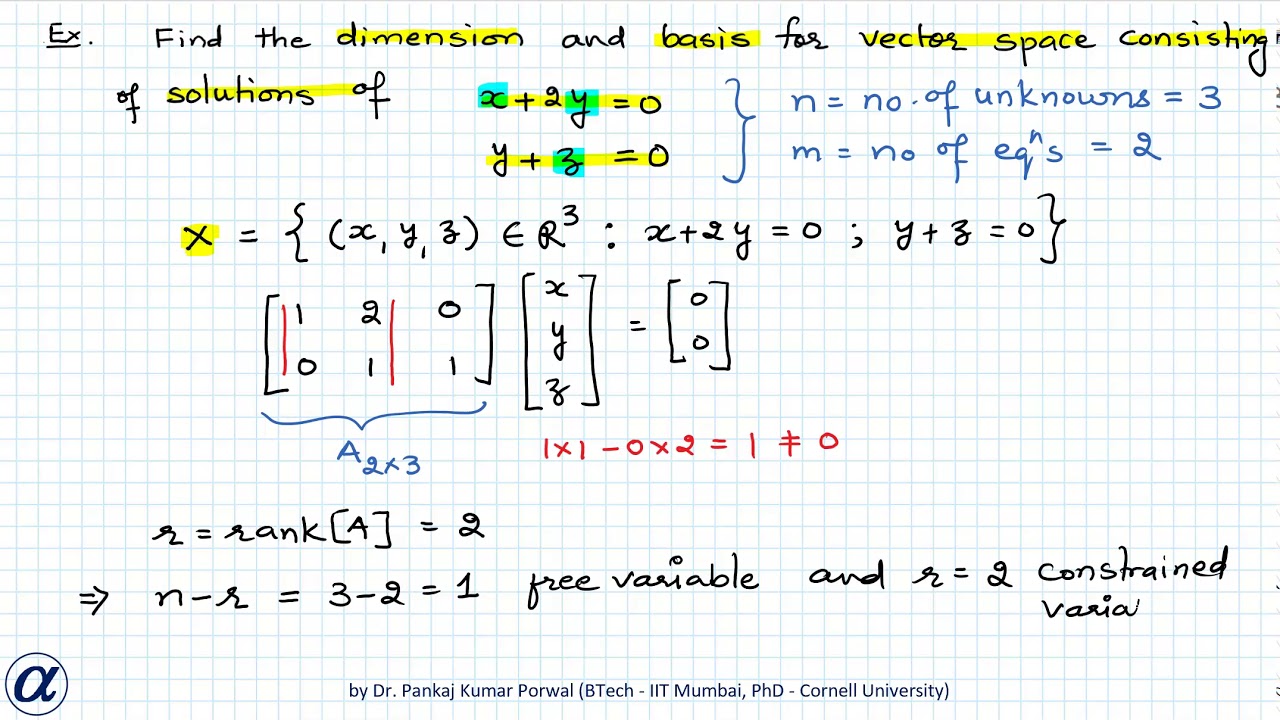
Find the dimension and basis of vectors space spanned by solutions of homogeneous system of equations
x+2y = 0
y+z = 0

in other words
Find the dimension and bais for the vector space V={(x, y, z) in R3 : x+2y = 0 , y+z = 0 }

Vector Space V – Span , Basis and Orthonormal basis
A collection of vectors which is closed under addition and scaler multiplication is called a vector space.

Closed under addition and scaler multiplication implies that linear combination of any number of vectors in the vector space also lies in the vector space.

Span – A collection of vectors v1, v2, …vk from vector space V is said to span V if every vector in V can be written as linear combination of v1, v2, …vk. (Note that v1, v2, …vk do not have to be linearly independent).

Basis – A collection of linearly independent vectors v1, v2, …vn that spans vector space V is called basis of vector space V. Basis for a vector space is not unique but number of vectors in the basis is fixed. This fixed number of vectors in basis is called dimension of vector space dim V or dim(V).

Every vector in vector space V can be written as linear combination of basis in a unique way. The scalers in the linear combination are called coordinates of the vector with respect to the basis.

Orthonormal basis – If the vectors in the basis are orthogonal to each other and have unit magnitude then the basis is called orthonormal basis. Any basis can be converted into orthonormal basis.

This video is uploaded by
Alpha Academy, Udaipur
Minakshi Porwal (9460189461)

[BushraImran-zn]

The value you assign like alpha minus alpha is random??

[eunicedeepthivanka]

Sir what do you mean by x, y, z belonging to R^3