Finding Basis for Column Space, Row Space, and Null Space - Linear Algebra

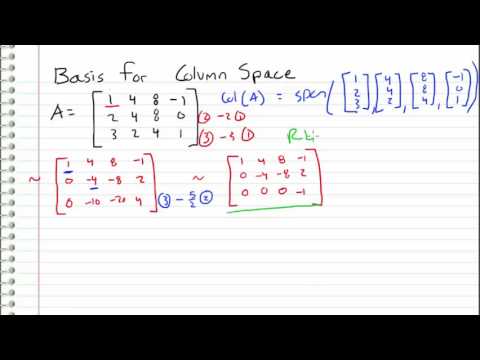
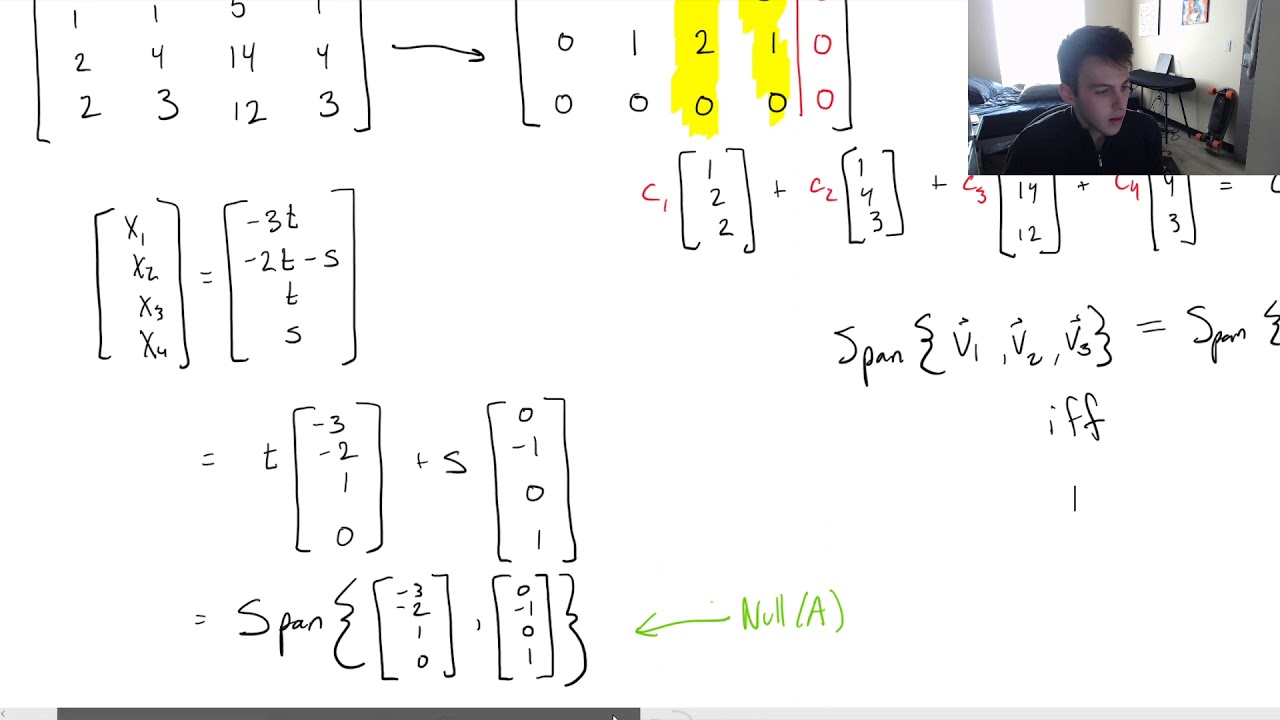
What exactly is the column space, row space, and null space of a system? Let's explore these ideas and how do we compute them?

[tony-hzgg]

Bro you're a goat I never comment but u made everything so much easier to understand than the other tutors who just yap about definitions, but you explain the intuition. Love it def gonna start watching u more for linear.

[hagopderghazarian]

I never comment on videos but you my friend just aced this chapter. Khan academy complicates it for no reasons. Great job

[rustomcadet]

Thank you for this; you makes things much easier to understand.

[pharaohscurse]

Thank you so much. Finally understood the concept perfectly

[volken]

Great! Thanks for this simple and intelligent explanation!

[semkiz]

omg i literally have my final tmrw and u just explained the concepts i've been dreading the most in the most understandable way ever omfg ur the goat

[AdrenalStorm]

OMG THANK YOU SO MUCH. You are a life saver. I was having so much trouble with a question on MyOpenMath and now I understand 😭

[TumuhairwePeace-wezd]

Thanks for good explanation, may God bless you abandantly

[FarheenQureshi-eijv]

best explanation of topic .... finally i understood the topic ... it is simple but our teacher make it very hard.

[moshiurrahman]

Excellent presentation. Thanks.
You presented it in consideration of a homogenous system. Could you please add some explanation of this topics in a non-homogenous system? You are a great teacher!

[maxpercer]

interesting you say that applying a linear transformation is 'shifting space'. So that is one way to think about it, as a mapping between two spaces, the departure space and the arrival space, or as transformation of the departure space.
A linear transformation is equivalent to matrix multiplication, and for the null space we are looking for solutions to A*x = 0, where x is an n x 1 matrix of "solutions" and A is a given m x n matrix. When x varies you have a map from R^n -> R^m, defined by x -> A * x .

[nattavich]

Thank you for teaching. It helps me to solve my homework. And if you don’t mind, please you will suggest the book of Linear Algebra.

[cerberuss]

thank you! my endterm is tomorrow, u helped a lot!

[ColeWagner-lj]

Hey thought the video was great but I think your definition on independence may be off. A matrix is independent if the subsets don’t contain other subset variables. Your first problem you said was independent was actually dependent even though it spanned

[bumguqs]

thank you so much! btw your voice is super cool

[cornmasterliao]

so for column space I should use the corresponding column vectors in the original matrix. for row space I should use the row vectors in the RREF matrix?

[titaniumx]

explained it better than my prof and my textbook combined. appreciate it man thank you

[mirmubasher]

will the dimensions of basis of col(A) and row(A) always be the same?
Does dimensions of basis of null(A) hold any significance with col(A) and row(A)?

Thank you!
you're blessed.

[abdelazizamr]

great video you deserve more likes and subscribes

[maxpercer]

11:22 I think there is a mistake, it should be the span of {v1, v2, v3, v3} = span {v1, v2}, not
span {v1, v2, v3 } = span v1, v2, since there are four vectors we started with in Col(A).