filmov
tv
Independence, Basis, and Dimension
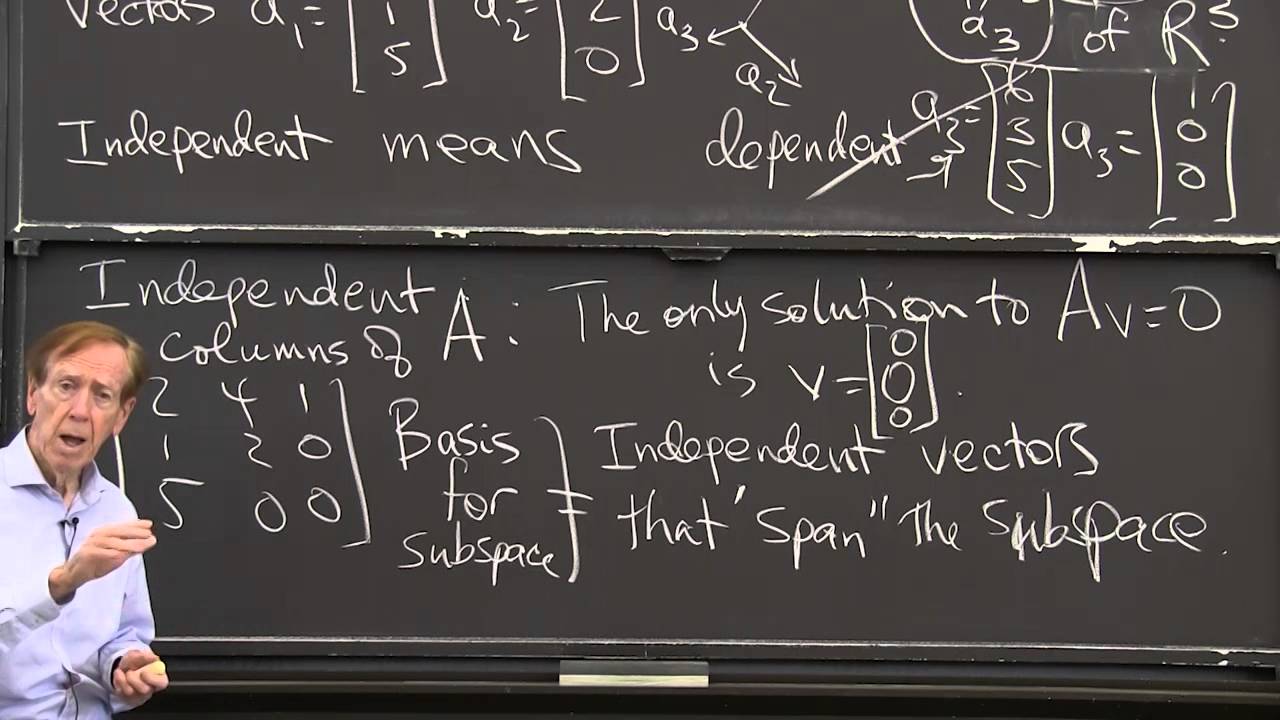
Показать описание
MIT RES.18-009 Learn Differential Equations: Up Close with Gilbert Strang and Cleve Moler, Fall 2015
Instructor: Gilbert Strang
Vectors are a basis for a subspace if their combinations span the whole subspace and are independent: no basis vector is a combination of the others. Dimension = number of basis vectors.
License: Creative Commons BY-NC-SA
Instructor: Gilbert Strang
Vectors are a basis for a subspace if their combinations span the whole subspace and are independent: no basis vector is a combination of the others. Dimension = number of basis vectors.
License: Creative Commons BY-NC-SA
Independence, Basis, and Dimension
Basis and Dimension
Linear combinations, span, and basis vectors | Chapter 2, Essence of linear algebra
Linear Independence
The Vector Space of Polynomials: Span, Linear Independence, and Basis
Basis and Dimension
Linear Algebra 4.3.1 Linearly Independent Sets and Bases
Introduction to linear independence | Vectors and spaces | Linear Algebra | Khan Academy
[Linear Algebra] Linear Independence and Bases
Span and linear independence example | Vectors and spaces | Linear Algebra | Khan Academy
Independence, Basis, Dimension - Examples
Basis of a subspace | Vectors and spaces | Linear Algebra | Khan Academy
Basis and dimension
Abstract Algebra, Lec 33A: Review Linear Algebra: Linear Independence, Basis, Dimension, Examples
Span, basis and dimension | Lecture 18 | Matrix Algebra for Engineers
Linear Algebra Example: Span Questions
EECE212 Note Set 17a Independence, Basis, Dimension
❖ Linear Independence and Linear Dependence, Ex 1 ❖
Linear Independence, Dimension & Basis
Subspaces and Span
How to find out if a set of vectors are linearly independent? An example.
Lecture 9 - Independence, Basis and Dimension
Basis and dimension of a vector space
Null space and column space basis | Vectors and spaces | Linear Algebra | Khan Academy
Комментарии
 0:13:20
0:13:20
 0:10:06
0:10:06
 0:09:59
0:09:59
 0:12:56
0:12:56
 0:12:50
0:12:50
 0:08:10
0:08:10
 0:15:53
0:15:53
 0:15:46
0:15:46
![[Linear Algebra] Linear](https://i.ytimg.com/vi/OLqc_rt7abI/hqdefault.jpg) 0:08:40
0:08:40
 0:16:53
0:16:53
 0:57:30
0:57:30
 0:19:00
0:19:00
 0:06:20
0:06:20
 0:28:43
0:28:43
 0:10:34
0:10:34
 0:12:47
0:12:47
 0:27:55
0:27:55
 0:08:05
0:08:05
 0:23:30
0:23:30
 0:05:50
0:05:50
 0:04:41
0:04:41
 1:00:01
1:00:01
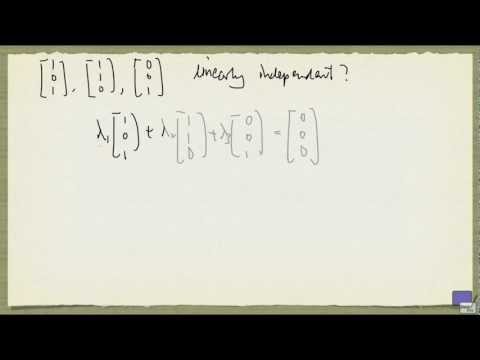 0:12:46
0:12:46
 0:25:13
0:25:13