Basis & dimension of Solution set for SOLE | Linear Algebra

In this video, we will find the particular solution for a system of linear equations, and we'll also try and understand the meaning of some standard terms used.
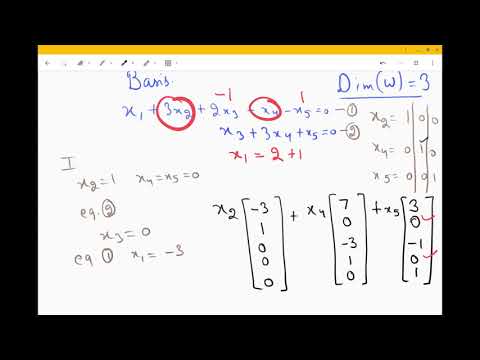
To evaluate the general solution, in this case we have discussed, we can just put the free variables as a, b & c , seperately, i.e if one free variable is a, then other two are 0.
Then if second is b, the remaining two are zero.
Also, note that since this is a homogenous system of equations, the three base vectos we get at the end are the basis for the null space also, since they send the solution to 0.
If you still have doubts, ask me in the comments.