filmov
tv
Limit of x-sinx/x^3 as x approaches 0
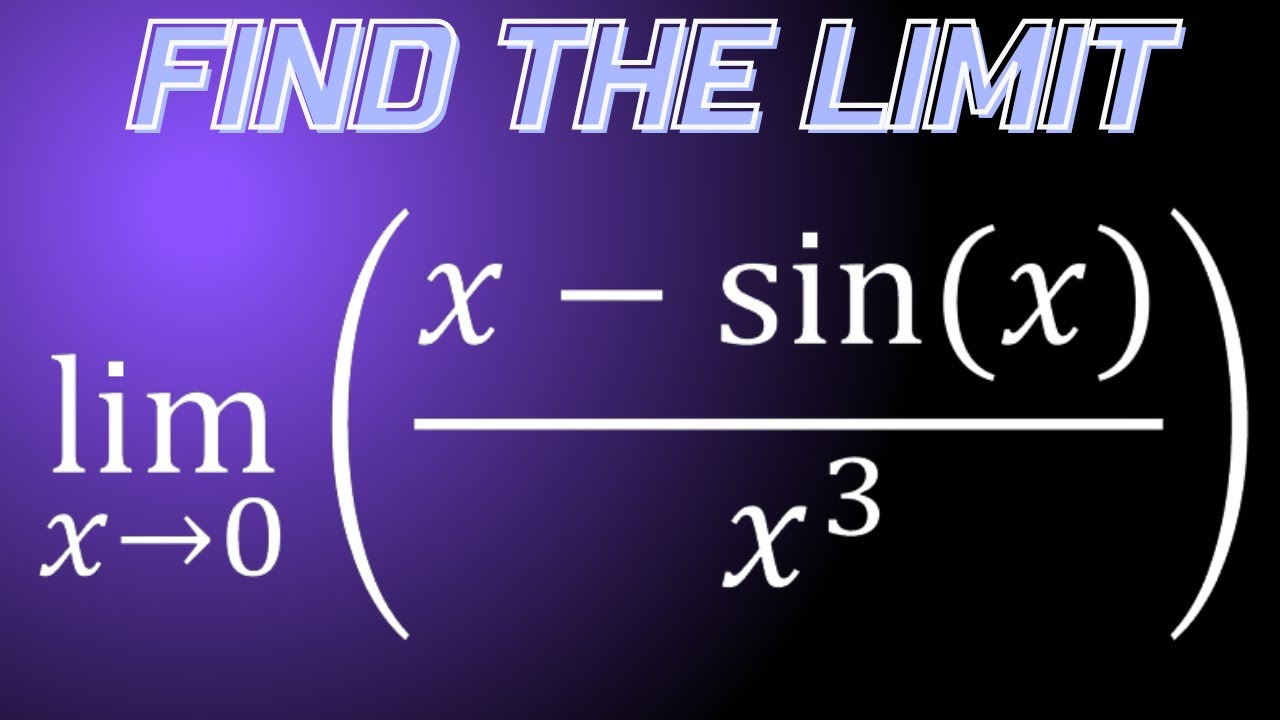
Показать описание
In this video we find the limit of x-sinx/x^3 as x approaches 0.
Each step is explained carefully. We use L'Hopital's rule when required. Limit Laws are taken for granted in this problem.
If you found this content useful, make sure to SUBSCRIBE and check out some of my other videos! Thanks for watching I hope you have a great day. All questions are welcome.
I have linked some videos below which I think you may find useful! If you are struggling on this topic make sure to check them out!
Limit of x^x^x as x goes to 0+ :
Limit of (1+1/x)^x as x approaches infinity :
Limit of (x/x+a)^x as x approaches infinity :
Limit of x^x as x goes to 0+ :
I hope you have a great day!
Each step is explained carefully. We use L'Hopital's rule when required. Limit Laws are taken for granted in this problem.
If you found this content useful, make sure to SUBSCRIBE and check out some of my other videos! Thanks for watching I hope you have a great day. All questions are welcome.
I have linked some videos below which I think you may find useful! If you are struggling on this topic make sure to check them out!
Limit of x^x^x as x goes to 0+ :
Limit of (1+1/x)^x as x approaches infinity :
Limit of (x/x+a)^x as x approaches infinity :
Limit of x^x as x goes to 0+ :
I hope you have a great day!
Limit of x-sinx/x^3 as x approaches 0
limit x to 0 of (x-sinx)/x^3
lim x tends to x-sinx / x^3 = 1/6
Limits of Trigonometric Functions
Limit of sin(x)/x as x goes to Infinity (Squeeze Theorem) | Calculus 1 Exercises
Finding a Limit Involving sinx/x as x approaches zero Example 3
The most important limit in Calculus // Geometric Proof & Applications
Limit of sinx/x^(1/3) as x approaches 0 | Calculus 1 Exercises
GATE 2025: Data Science & AI - Calculus & Optimization Practice + PYQs (Part 3) | GfG GATE
limit x tends to 0 (x - Sinx)/(x^3)
lim x/ Sinx as x tends to 0 , # trick # Easy # Short
Limit tanx-sinx/x^3 as x approaches 0 (without L'Hopital's rule)
How to Solve the Limit of (x - sinx)/x^3 as x Approaches 0: Step-by-Step Guide with 2 Methods
limx→0(tanx-sinx)/x^3=?
limit tanx-sinx/x^3 as x approaches 0
lim sinx/x | limit x tends to infinity sinx/x | sinx/x | lim x - 0 sinx/x
Evaluate the limit of sinx over x
Lim (x-tanx)/(x-sinx) as x goes to 0
Limit of sinx-x/x^3 as x approaches 0
lim sinx/x as x tends to 0 Intuition #math #calculus #limit #sinx/x #sinx/x as x tends to 0
Limit (x-sinx)/x^3 where x goes to 0
lim x→0( tanx-sinx/x^3) || L'Hospital Rule
Proof: Limit of sinx/x as x approaches 0 with Squeeze Theorem | Calculus 1
Limit of sin(x)/x as x approaches 0 | Derivative rules | AP Calculus AB | Khan Academy
Комментарии
 0:01:35
0:01:35
 0:01:51
0:01:51
 0:04:15
0:04:15
 0:15:23
0:15:23
 0:03:59
0:03:59
 0:02:32
0:02:32
 0:11:54
0:11:54
 0:01:51
0:01:51
 0:43:06
0:43:06
 0:02:36
0:02:36
 0:00:17
0:00:17
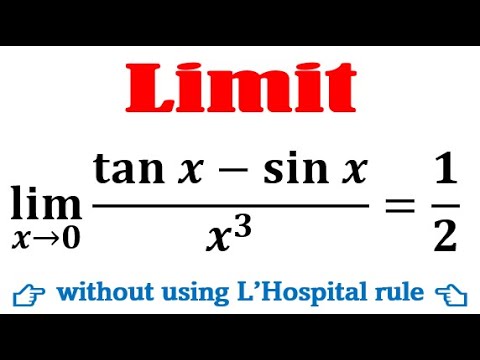 0:05:47
0:05:47
 0:14:32
0:14:32
 0:02:51
0:02:51
 0:04:14
0:04:14
 0:03:25
0:03:25
 0:03:28
0:03:28
 0:07:43
0:07:43
 0:02:12
0:02:12
 0:00:59
0:00:59
 0:00:39
0:00:39
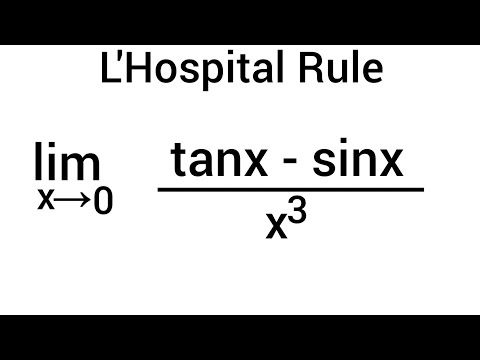 0:03:04
0:03:04
 0:10:21
0:10:21
 0:09:16
0:09:16