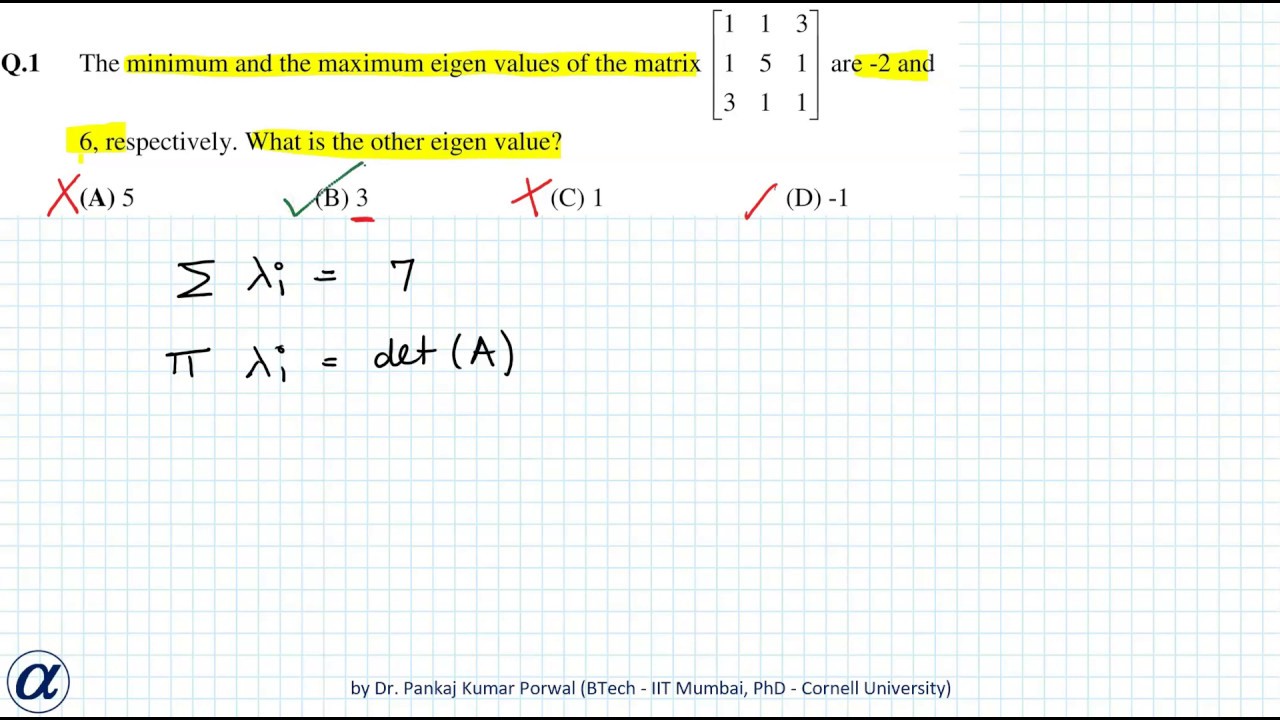
Ex 10 - The minimum and maximum eigenvalues of matrix are –2 and 6 respectively. The other two

GATE Eigenvalue Eigenvector Problems Short Cut Method Example 10

Q. The minimum and maximum eigen values of matrix are –2 and 6 respectively. The other two eigen values are (GATE CE 2007)

Theory

Eigenvalue problems often show up in physics and many branches of engineering. Such problems are in the form

A v = lambda * v

Here A is a matrix, v is eigenvector, lambda is eigenvalue. Eigenvector and eigenvalue are often incorrectly written as eigen vector and eigen value.

The matrix A corresponds to some linear transformation on vector v. The vector v satisfying above equation is called eigenvector.

In general when we multiply a matrix A ( n x n ) with a vector v (n x 1) we get a vector Av, which is not parallel to v. However, when v is an eigenvector of A then A v = lambda v is parallel to v. lambda is the factor by which v is scaled, i.e., magnitude of v is multiplied by lambda. lambda is called the eigenvalue. v is eigenvector corresponding to eigenvalue lambda .

In GATE most of eigenvalue / eigenvector problems are solved using properties of eigenvalues and eigenvectors.

Property 1. When a matrix is multiplied by its eigenvector then the eigenvector gets scaled by eigenvalue corresponding to the vector without change in the direction ( rotation ) of the eigenvector.

Property 2. Sum of eigenvalues of a matrix is equal to trace of the matrix, i.e., sum of diagonal elements of the matrix.

Property 3. Product of eigenvalues of a matrix is equal to determinant of the matrix.

This part of the lecture covers short cut method to solve eigenvalue and eigenvector problems commonly asked in GATE exam.

This video is uploaded by
Alpha Academy, Udaipur
Minakshi Porwal (9460189461)