Find Standard Form of Equation of Circle Given Endpoints of a Diameter

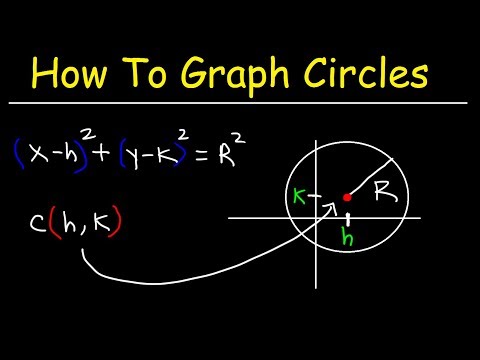
A circle has a diameter with endpoints (-5,2) and (7,-8). Find the equation of the circle in standard form. The standard form of the equation of a circle is (x-h)^2+(y-k)^2=r^2 with the center of (h, k) and radius=r. The center is at the half way point of the diameter, so it is found using the midpoint formula. The radius is half the length of the diameter, so it is found using the distance formula. For two points (x1, y1) and (x2, y2), the distance formula is d=sqrt((x2-x1)^2+(y2-y1)^2) and the midpoint formula is (x,y)=((x1+x2)/2, (y1+y2)/2)). The center and radius are then used to write the equation of the circle in standard form.

Timestamps
0:00 Introduction
0:18 Standard Form
1:56 Find Center (Midpoint Formula)
3:26 Find Radius (Distance Formula)
5:55 Equation of Circle