filmov
tv
Solving A Differential Equation | Two Methods

Показать описание
🤩 Hello everyone, I'm very excited to bring you a new channel (SyberMath Shorts)
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#CalculusProblems #DifferentialEquations #Integrals
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#CalculusProblems #DifferentialEquations #Integrals
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Separable First Order Differential Equations - Basic Introduction
How to solve differential equations
Differential equation introduction | First order differential equations | Khan Academy
How to determine the general solution to a differential equation
How to solve ANY differential equation
Differential equations, a tourist's guide | DE1
First order, Ordinary Differential Equations.
First Order Linear Differential Equations
Differential Equations: systems of linear differential equations introduction, 9-6-24
First Order Linear Differential Equation & Integrating Factor (introduction & example)
4 Types of ODE's: How to Identify and Solve Them
Edexcel A level Maths: 11.10 Solving Differential Equations (Part 1)
How to Solve First Order Linear Differential Equations
Solving an Exact Differential Equation
What are Differential Equations and how do they work?
Finding particular linear solution to differential equation | Khan Academy
How To Solve First Order Homogeneous Differential Equation
Differential Equations - Solution of a Differential Equation
General Solution of a Differential Equation
Solving A Differential Equation
A-Level Maths: H7-01 Differential Equations: Introducing Differential Equations
Physics Students Need to Know These 5 Methods for Differential Equations
How To Solve Differential Equations | By direct Integration.
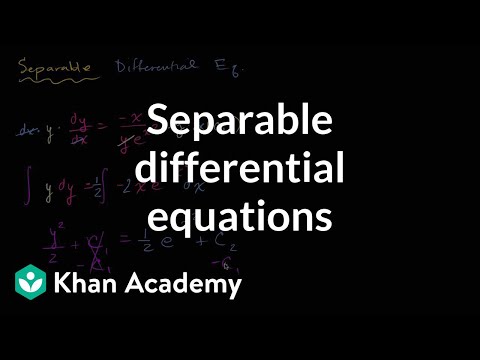
Separable differential equations introduction | First order differential equations | Khan Academy
Комментарии
 0:10:42
0:10:42
 0:00:46
0:00:46
 0:07:49
0:07:49
 0:02:03
0:02:03
 0:05:05
0:05:05
 0:27:16
0:27:16
 0:48:35
0:48:35
 0:22:28
0:22:28
 0:51:19
0:51:19
 0:20:34
0:20:34
 0:06:57
0:06:57
 0:14:07
0:14:07
 0:10:53
0:10:53
 0:02:46
0:02:46
 0:09:21
0:09:21
 0:06:30
0:06:30
 0:08:33
0:08:33
 0:08:01
0:08:01
 0:02:31
0:02:31
 0:12:22
0:12:22
 0:04:32
0:04:32
 0:30:36
0:30:36
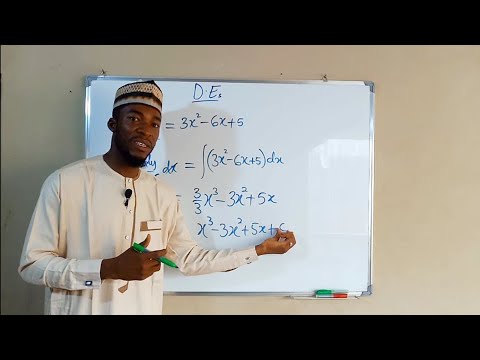 0:07:33
0:07:33
 0:09:05
0:09:05