filmov
tv
What are Differential Equations and how do they work?

Показать описание
In this video I explain what differential equations are, go through two simple examples, explain the relevance of initial conditions and how differential equations generally work, and then discuss what this means to the question whether the future is determined already. This video will be continued next week by a video about what this means for free will.
#physics #science #mathematics
0:00 Motivation and Content Summary
0:55 Example Disease Spread
3:25 Example Newton's Law
5:18 Initial Values
6:15 What are Differential Equations used for?
7:08 How Differential Equations determine the Future
#physics #science #mathematics
0:00 Motivation and Content Summary
0:55 Example Disease Spread
3:25 Example Newton's Law
5:18 Initial Values
6:15 What are Differential Equations used for?
7:08 How Differential Equations determine the Future
What are Differential Equations and how do they work?
Differential equations, a tourist's guide | DE1
Differential equation introduction | First order differential equations | Khan Academy
This is why you're learning differential equations
What are differential equations?
What is a DIFFERENTIAL EQUATION?? **Intro to my full ODE course**
What are differential equations?
Overview of Differential Equations
What is a differential equation? Applications and examples.
Separable First Order Differential Equations - Basic Introduction
Differential Equations and Dynamical Systems: Overview
Differential Equations: The Language of Change
Physics Students Need to Know These 5 Methods for Differential Equations
But what is a partial differential equation? | DE2
Differential Equations. All Basics for Physicists.
Differential Equations Introduction | Differential Calculus Basics #differentialequation
4 Types of ODE's: How to Identify and Solve Them
First Order Linear Differential Equation & Integrating Factor (introduction & example)
Introduction to Differential Equations (Differential Equations 2)
Calculus - Order and Degree of a Differential Equation | Don't Memorise
🔵01 - Differential Equations, Order, Degree, Ordinary and Partial Differential Equation
First Order Linear Differential Equations
Differential Equations: Lecture 1.1-1.2 Definitions and Terminology and Initial Value Problems
Differential Equations - Introduction, Order and Degree, Solutions to DE
Комментарии
 0:09:21
0:09:21
 0:27:16
0:27:16
 0:07:49
0:07:49
 0:18:36
0:18:36
 0:03:41
0:03:41
 0:11:26
0:11:26
 0:05:35
0:05:35
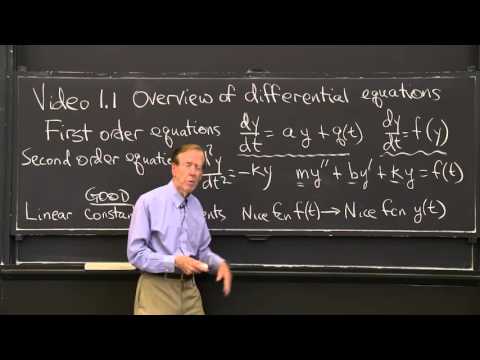 0:14:04
0:14:04
 0:02:11
0:02:11
 0:10:42
0:10:42
 0:29:31
0:29:31
 0:23:24
0:23:24
 0:30:36
0:30:36
 0:17:39
0:17:39
 0:47:38
0:47:38
 0:18:50
0:18:50
 0:06:57
0:06:57
 0:20:34
0:20:34
 0:09:56
0:09:56
 0:05:31
0:05:31
 0:21:50
0:21:50
 0:22:28
0:22:28
 1:06:42
1:06:42
 0:34:59
0:34:59