Tangent spaces and Riemannian manifolds

Показать описание

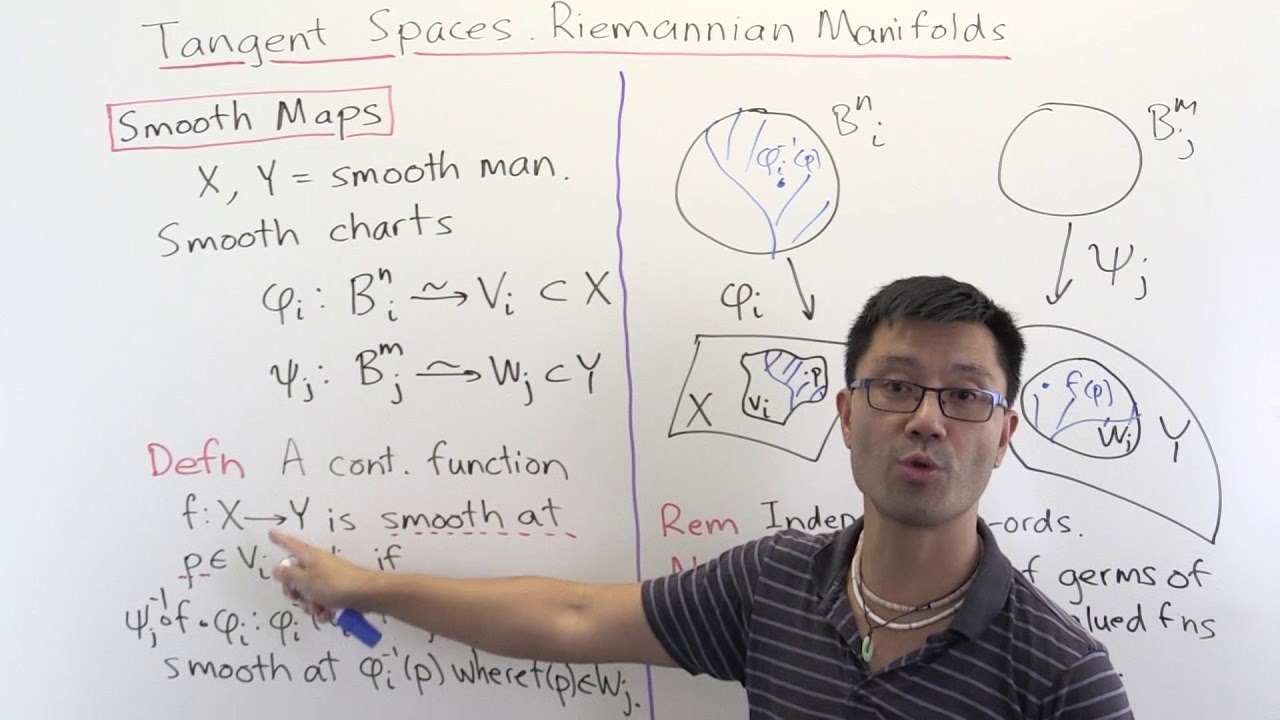
In this video, we give three alternative ways to view tangent vectors on manifolds. The first is dynamic, viewing tangent vectors as velocities of trajectories, the second is via identifying tangent vectors with their directional derivatives. Lastly, we consider the dual approach, defining first the cotangent space via differentials. We then give the definition of Riemannian manifolds and show how this concept allows one to define lengths of curves on a manifold.

Рекомендации по теме

Комментарии

As someone who was trained in an applied mathematics background, I had to look up quite a few technical vocabularies you used in your videos. Thank you for your eye-opening expositions!

MathwithMing

I love this channel. The videos are conceptual and worth coming back to later, while also including some interesting proofs. I watched some of the videos in this playlist before, but I found that I lacked some of the background in topology and analysis. I know a bit more now, so I am binge watching the whole list and learning a lot. Please keep on making videos!

fbkintanar

This was *the* perfect lecture, thank you!

ramanasubramanyam

to define directions in terms of directional derivatives ... it kind of makes sense if directions will only be used in conjunction with directional derivatives at a distinguished point. and it smells like a universal property :)

JoelSjogren

@11:10, if we are taking a germ f at p, then for any other germ at p, say g, they agree for some small enough neighborhood of p, so the derivative D_gamma f = D_gamma g, is that correct? If that is true, then for the definition of derivation at a point p, doesn't that mean f(p)=g(p), and δ(f)=δ(g)?

AzizBouland

I suppose it doesn't matter because they are homeomorphisms, but I've always seen chart maps defined from an open neighborhood in the manifold to a subset of R^n and not the other way around as you have. Is there any advantage to defining them this way?

billf

Great video! By the way, at around 18:00 you mention that every derivation on C^{\infty}(M)_{p} is in fact a directional derivative. How do we know this? Tried proving it myself but no luck...

joshuagraham

Please bring the camera lower. Your hand disappears

zaheddastan

Tangent spaces and Riemannian manifolds

Tangent spaces and Riemannian manifolds

Manifolds #5: Tangent Space (part 1)

Manifolds #5 - Tangent Space (Introduction)

Manopt.jl, Optimization on Riemannian Manifolds | Ronny Bergmann

Differential Geometry in Under 15 Minutes

JuliaCon 2020 | Manifolds in Julia – Manifolds.jl & ManifoldsBase.jl | Ronny Bergmann

Tangent space, basis vectors, and Riemannian metric

Riemannian manifold

Advanced Calculus: gradient on Riemannian manifold, metric construction, 11-6-17, part 1

Manifolds 4.1 : Introduction to the Tangent Space

Differential Forms: PART 1A: TANGENT SPACES (INTUITIVELY)

Manifolds and Tangent Spaces

Manifold | Riemannian Manifold | Differential geometry lecture video | Differential geometry lecture

Relativity 107b: General Relativity Basics - Manifolds, Covariant Derivative, Geodesics

Geodesic flow on Riemannian manifolds 1

What is a sub-Riemannian manifold?

Analysis II Lecture 12 Part 2 tangent space using curves

Neshan Wickramasekera: Variational theory of minimal hypersurfaces in Riemannian manifolds

Riemannian Geometry || EP.2 (Tangent Spaces || Part 1)

Riemannian Geometry - Definition: Oxford Mathematics 4th Year Student Lecture

21.2 The tangent space to a manifold

Stephan Mescher (3/10/22): Geodesic complexity of Riemannian manifolds

Differential Geometry - Student Talks Ep 1. (Pseudo - ) Riemannian Manifolds and Relativity

The Christoffel Symbols In Riemannian Geometry