filmov
tv
Manifolds #5: Tangent Space (part 1)

Показать описание
Today, we introduce the notion of tangent vectors and the tangent vector space at a point on a manifold.
Manifolds #5: Tangent Space (part 1)
Manifolds #5 - Tangent Space (Introduction)
Differential Forms: PART 1A: TANGENT SPACES (INTUITIVELY)
Intro to Manifolds Part 5: Mappings and Tangents
Tutorial 5: Tangent Spaces (International Winter School on Gravity and Light 2015)
Analysis II Lecture 12 Part 1 the tangent space
TSBP: Tangent Space Belief Propagation for Manifold Learning
Lecture 5: Tangent Spaces (International Winter School on Gravity and Light 2015)
Daniel Beltita - Leaf spaces in Lie theory
Manifolds 4.1 : Introduction to the Tangent Space
Lecture 5 Definition of the tangent manifold
Analysis II Lecture 12 Part 2 tangent space using curves
Finding Tangent Space to Cylinder
Tangent spaces and Riemannian manifolds
Lecture 4 Trying to define the tangent space
21.2 The tangent space to a manifold
Differentials-Part 5 Tangent Space for a parametric surface
Tangent Space to Submanifolds (part 1)- in terms of curves
Manifold 4.2 : Differentials of Smooth Maps and Dimension of the Tangent Space
Tangent Space
Aspects of the Tangent Space derivation
Tangent space
12.1 The tangent space - concretely (Commutative Algebra and Algebraic Geometry)
Tangent space to a Submanifold (part 3)- As derivations
Комментарии
 0:17:27
0:17:27
 0:09:42
0:09:42
 0:05:43
0:05:43
 0:27:28
0:27:28
 1:32:26
1:32:26
 0:12:28
0:12:28
 0:09:57
0:09:57
 1:36:41
1:36:41
 0:57:36
0:57:36
 0:27:11
0:27:11
 0:17:09
0:17:09
 0:11:34
0:11:34
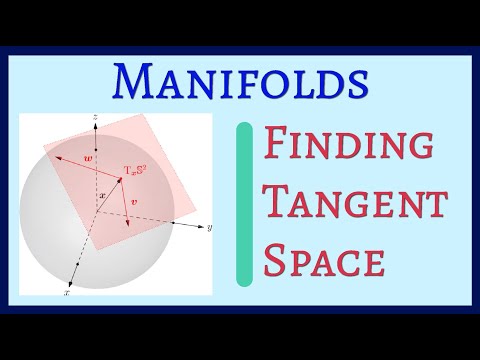 0:13:16
0:13:16
 0:31:07
0:31:07
 0:32:58
0:32:58
 0:34:01
0:34:01
 0:08:32
0:08:32
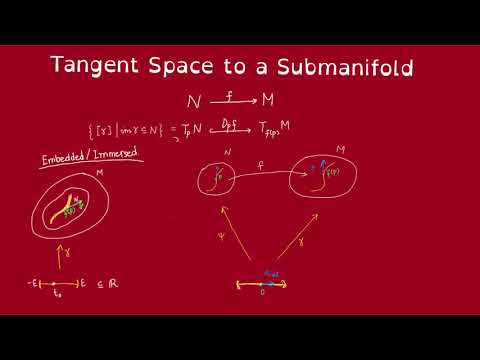 0:06:41
0:06:41
 0:23:09
0:23:09
 0:10:01
0:10:01
 0:06:50
0:06:50
 0:10:44
0:10:44
 0:14:59
0:14:59
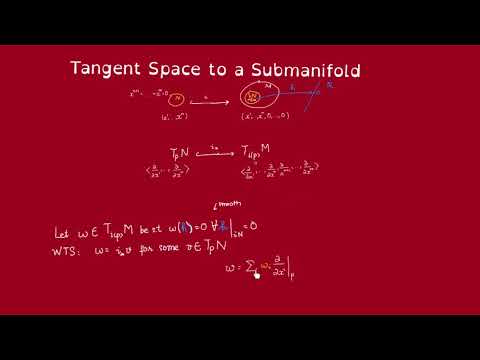 0:07:06
0:07:06