filmov
tv
completing the square with two variables, and application to ellipse equation
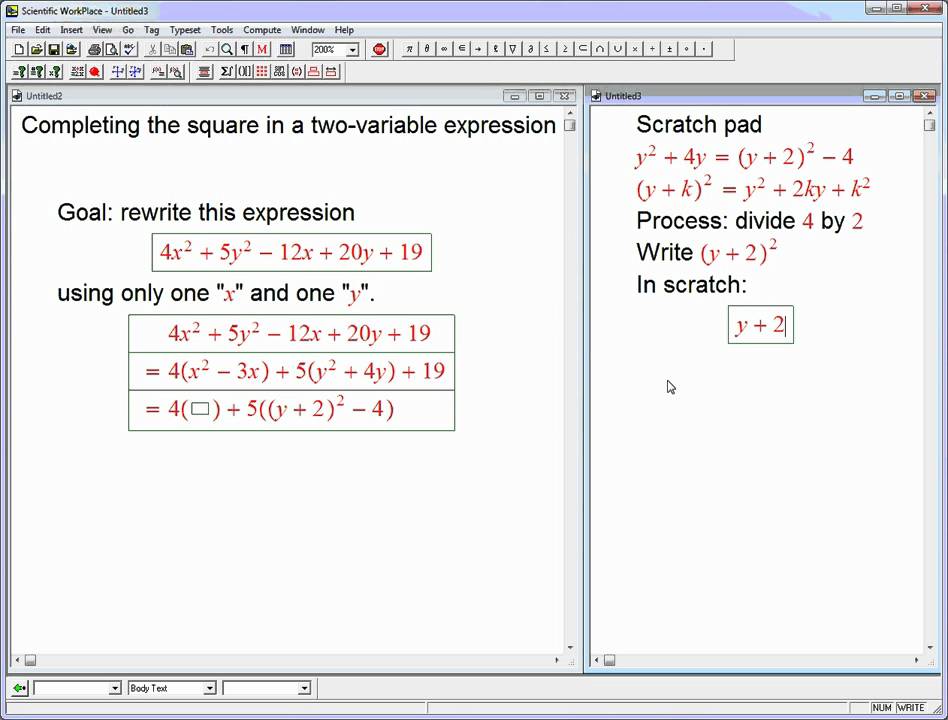
Показать описание
I show an example of completing the square in an expression with two variables, and go on to an application to putting an ellipse equation in standard form.
Completing the Square 2 - Corbettmaths
Solve by completing the square | Step by Step Technique
Completing the Square Visually
Solving Quadratic Equations By Completing The Square
GCSE Maths - How to Factorise Quadratics by Completing the Square (Part 1 - Factorise When a=1) #52
Solving a quadratic by completing the square
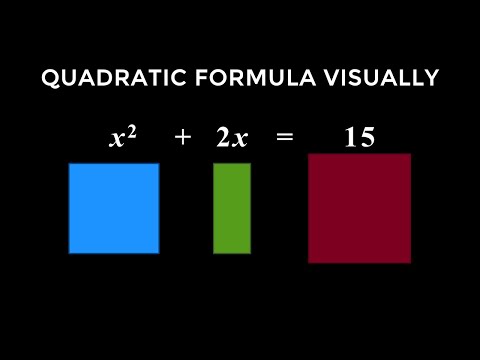
Completing the Square (visual proof)
Solving a quadratic by completing the square
Learn Algebra: Quadratic Equations - 3x/(x+2)+1/(x-1)=(4-7x)/(x^2+x-2)
Completing the square of two functions
Solve by Completing the Square: Step-by-Step Technique
How Solve to by || Completing the Square || Quadratic Equation || x-2/x-5=0
Solving quadratic equations by completing the square | Algebra II | Khan Academy
❖ Completing the Square Example 2 Solve Quad. Equations ❖
Identifying a Conic from an Equation by Completing the Square, Ex 2
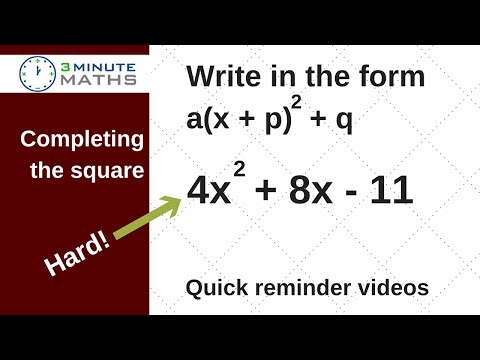
Higher level completing the square, write in the form a(x + p)squared + q
04 - Completing the Square to Solve Quadratic Equations - Part 1
Completing the Square (1 of 2: Explanation)
Completing the square to transform from standard to vertex form
GCSE Maths - How to Solve a Quadratic by Completing the Square (Part 2 - Solve When a=1) #53
Solving Quadratics by Completing the Square
Completing the Square (2 of 2: Example)
How to Solve By Completing the Square (NancyPi)
Why do we 'complete the square'?
Комментарии
 0:09:17
0:09:17
 0:05:20
0:05:20
 0:00:54
0:00:54
 0:07:57
0:07:57
 0:05:14
0:05:14
 0:10:43
0:10:43
 0:01:26
0:01:26
 0:04:10
0:04:10
 0:15:15
0:15:15
 0:05:19
0:05:19
 0:07:40
0:07:40
 0:07:28
0:07:28
 0:14:06
0:14:06
 0:05:18
0:05:18
 0:02:22
0:02:22
 0:03:24
0:03:24
 0:22:34
0:22:34
 0:10:19
0:10:19
 0:04:14
0:04:14
 0:03:37
0:03:37
 0:07:32
0:07:32
 0:08:20
0:08:20
 0:17:33
0:17:33
 0:09:50
0:09:50