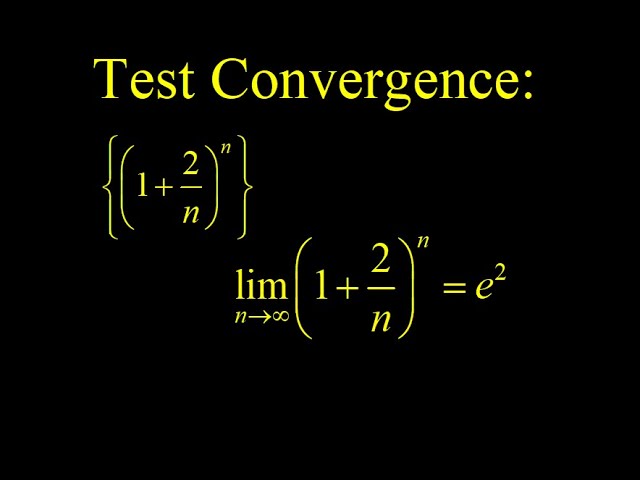
Convergence of a sequence (1+2/n)^n leading to a limit of e^2.

Questions or requests? Post your comments below, and I will respond within 24 hours.

We test for convergence in the sequence (1+2/n)^n in two different ways. First, we compare to the definition of e as the large n limit of (1+1/n)^n by making a substitution. Second, we compute the limit by using a function on the real numbers, taking the log of both sides, and using L'Hopital's rule to deal with an indeterminate form. Either way, the sequence converges to e^2.