filmov
tv
Convergence of an infinite sequence with factorials trick: test the convergence of 2^n/n!

Показать описание
Infinite sequence with factorials trick! We test convergence of 2^n/n!: an infinite sequence with factorials and exponentials in the sequence. The trick for this problem is not obvious, but sequences with factorials often require this sort of trick to test the convergence of an infinite sequence.
We begin by writing the first several terms of the sequence to look for a pattern, and we show each term is less than or equal to 4/n. This puts an upper bound on the terms of the sequence, and we also know that each term is positive, so the lower bound on the terms is zero.
Taking the limit of the upper bound for the sequence, we get that the limit of 4/n as n goes to infinity is zero. Finally, we apply the squeeze theorem for sequences to conclude that the limit of 2^n/n! is zero as well, so the sequence is convergent.
We begin by writing the first several terms of the sequence to look for a pattern, and we show each term is less than or equal to 4/n. This puts an upper bound on the terms of the sequence, and we also know that each term is positive, so the lower bound on the terms is zero.
Taking the limit of the upper bound for the sequence, we get that the limit of 4/n as n goes to infinity is zero. Finally, we apply the squeeze theorem for sequences to conclude that the limit of 2^n/n! is zero as well, so the sequence is convergent.
Convergence and Divergence - Introduction to Series
Converging and Diverging Sequences Using Limits - Practice Problems
Convergence and Divergence: The Return of Sequences and Series
Infinite Sequences - Convergent or Divergent? | Calculus 2 | Math with Professor V
Determining whether a sequence converges or diverges
Calculus 2 Lecture 9.1: Convergence and Divergence of Sequences
Choosing Which Convergence Test to Apply to 8 Series
Convergent and divergent sequences | Series | AP Calculus BC | Khan Academy
Convergence of an infinite sequence with factorials trick: test the convergence of 2^n/n!
Simple Guide to Series Convergence Tests
Infinite Series - Numberphile
Sequences | Convergence and Divergence
Geometric Series | Convergence, Derivation, and Example
Calculus BC – 10.1 Defining Convergent and Divergent Infinite Series
Calculus 2 - Geometric Series, P-Series, Ratio Test, Root Test, Alternating Series, Integral Test
Infinite series as limit of partial sums | Series | AP Calculus BC | Khan Academy
Geometric series convergence and divergence examples | Precalculus | Khan Academy
Calculus 2 - Integral Test For Convergence and Divergence of Series
100 series convergence tests (no food, no water, no stop)
Finding The Sum of an Infinite Geometric Series
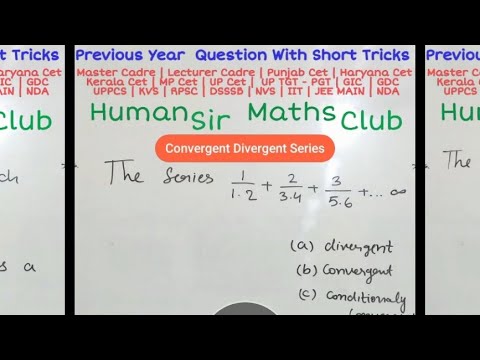
Infinite Series & Sequence : Convergence Super Tricks | KVS | DSSSB | NVS | IIT | JEE | NDA
Introduction to Infinite Series
Sequence & Series | Convergence & Divergence | Introduction | Maths
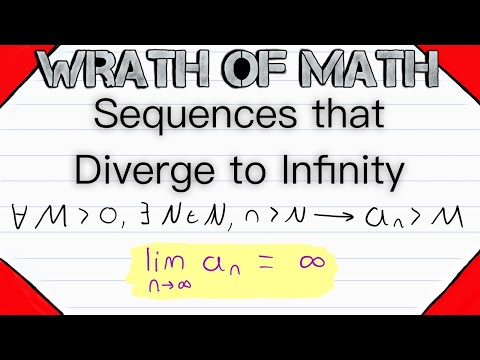
Sequences that Diverge to Infinity (Definition) | Calculus, Real Analysis
Комментарии
 0:16:18
0:16:18
 0:30:13
0:30:13
 0:09:40
0:09:40
 0:28:59
0:28:59
 0:05:39
0:05:39
 2:27:29
2:27:29
 0:12:13
0:12:13
 0:04:59
0:04:59
 0:05:00
0:05:00
 0:15:36
0:15:36
 0:09:31
0:09:31
 0:27:54
0:27:54
 0:06:28
0:06:28
 0:16:31
0:16:31
 0:43:52
0:43:52
 0:04:47
0:04:47
 0:07:08
0:07:08
 0:28:17
0:28:17
 6:06:54
6:06:54
 0:19:50
0:19:50
 0:01:00
0:01:00
 0:08:16
0:08:16
 0:21:22
0:21:22
 0:09:59
0:09:59