filmov
tv
Complex Analysis: Integral of (x^n)/(x+1) using Contour Integration
Показать описание
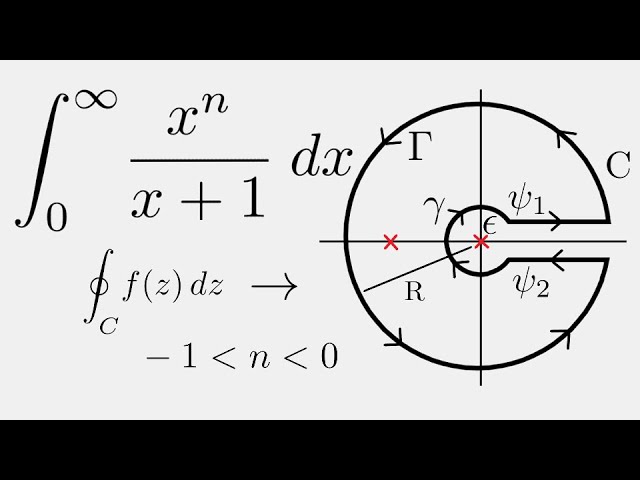
Today, we evaluate the improper integral from 0 to infinity of (x^n)/(x+1) where n is a negative real number between -1 and 0. We will be using this result in a future video!
Complex Analysis: Integral of (x^n)/(x+1) using Contour Integration
Complex Analysis: Integral of ln(x)/(x^n+1) using Contour Integration
Complex Analysis: Integral of 1/(x^n+1) feat. pizza contour
Complex Analysis: Integral of 1/(x^4+1) using Contour Integration
Integral of 1/(x^2+1) from -inf to inf, Contour Integral
A beautiful result in calculus: Solution using complex analysis ( Integral cos(x)/(x^2+1) )
Complex Analysis: Integral of x^k/(x^n+1) using Contour Integration
Complex Analysis: Integral of 1/(x^4-x^2+1) using Contour Integration
Approximate independence of permutation mixtures
Complex Analysis: Integral of x^(n-2)/(x^n+1) using Contour Integration
Complex Analysis: Integral of xsin(x)/(x^2+1) using Contour Integration
Integral of 1/(x^n+1) from 0 to infinity
Outtakes - Integral of ln(x)/(x^n+1) with Complex Analysis
IIT Bombay CSE 😍 #shorts #iit #iitbombay
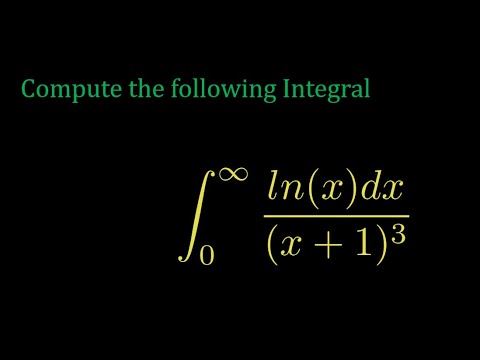
Complex analysis, contour integral: integral of ln(x)/(1+x)^3 from 0 to infinity
Integral of x^(n - 1)/(x^n + a)^n
2 ridiculously awesome log integrals solved using contour integration
1st yr. Vs Final yr. MBBS student 🔥🤯#shorts #neet
Integral xsinx/1+x^2 from 0 to infinity
Most💯 Important Step Before any Procedure 🔥
Complex Analysis | Unit 2 | Lecture 13 | Example of Cauchy's Integral Formula
Memorization Trick for Graphing Functions Part 1 | Algebra Math Hack #shorts #math #school
Complex Integration ( Part 2 ) Explanation & Examples
Salsa Night in IIT Bombay #shorts #salsa #dance #iit #iitbombay #motivation #trending #viral #jee
Комментарии
 0:50:28
0:50:28
 0:29:50
0:29:50
 0:36:33
0:36:33
 0:16:15
0:16:15
 0:19:50
0:19:50
 0:16:40
0:16:40
 0:26:52
0:26:52
 0:18:31
0:18:31
 0:57:43
0:57:43
 0:38:32
0:38:32
 0:17:37
0:17:37
 0:07:29
0:07:29
 0:23:52
0:23:52
 0:00:11
0:00:11
 0:06:29
0:06:29
 0:02:50
0:02:50
 0:26:14
0:26:14
 0:00:20
0:00:20
 0:08:45
0:08:45
 0:00:16
0:00:16
 0:09:05
0:09:05
 0:00:15
0:00:15
 0:11:49
0:11:49
 0:00:14
0:00:14