filmov
tv
Complex Analysis: Integral of ln(x)/(x^n+1) using Contour Integration

Показать описание
Today, we use complex analysis to evaluate the improper integral from 0 to infinity of ln(x)/(x^n+1) where our n is a real number greater than 2. We also come across some other familiar results which you can check out in more detail through these links to other videos I have created:
Solutions to z^n+1=0:
Integral of 1/(x^n+1):
Logarithm of complex numbers:
Complex exponential definition of sine:
Outtakes for integral over Gamma and gamma:
Solutions to z^n+1=0:
Integral of 1/(x^n+1):
Logarithm of complex numbers:
Complex exponential definition of sine:
Outtakes for integral over Gamma and gamma:
Complex Analysis: Integral of ln(x)/(x^n+1) using Contour Integration
Outtakes - Integral of ln(x)/(x^n+1) with Complex Analysis
integral lnx/1+x^n from 0 to infinity
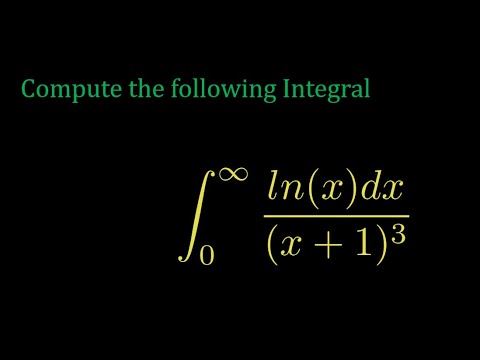
Complex analysis, contour integral: integral of ln(x)/(1+x)^3 from 0 to infinity
Integral of lnx/x
Complex Analysis: Integral of 1/(x^n+1) feat. pizza contour
Complex Analysis: Integral of log(x)/(x+1)^2
Complex contour Integral of ln(x)/(x^2+1)^2 from 0 to infinity
Complex Analysis: Integral of (x^n)/(x+1) using Contour Integration
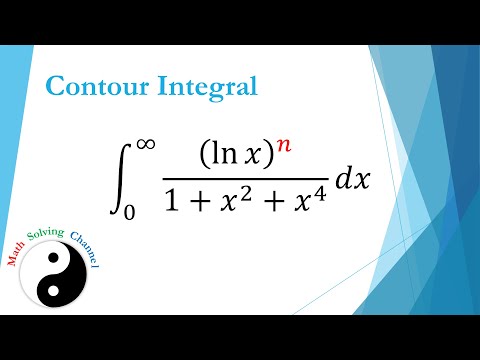
Contour Integral, Generating Function Method, ln(x)^n/(1+x^2+x^4)
Insane Contour Integral from @maths_505 !
Complex contour: Integral of ln(x)/(x^2+1) and ln(x)^2/(x^2+1)
Improvised Integrals #1: Integral of ln(x)/(x^2+1)^2 using Complex Analysis
Integral of ln(x!) from 0 to 1| Integral of ln(x factorial)
Contour Integral (Part 1) Alternative Method. Originally posted by Michael Penn, ln(x)/(1+x^2+x^4)
NEWYES Calculator VS Casio calculator
integral of x^n lnx from 0 to 1
How REAL Men Integrate Functions
Complex Analysis | Unit 2 | Lecture 13 | Example of Cauchy's Integral Formula
Cheating in exams😏!?
Complex Analysis: Nasty Integral with Elegant Solution
Human Calculator Solves World’s Longest Math Problem #shorts
Integral of lnx/x with Substitution
Reduction Formula for Integral of ∫ln^n(x)dx
Комментарии
 0:29:50
0:29:50
 0:23:52
0:23:52
 0:06:02
0:06:02
 0:06:29
0:06:29
 0:00:16
0:00:16
 0:36:33
0:36:33
 0:24:46
0:24:46
 0:12:25
0:12:25
 0:50:28
0:50:28
 0:09:02
0:09:02
 0:17:48
0:17:48
 0:10:13
0:10:13
 0:26:30
0:26:30
 0:03:02
0:03:02
 0:07:00
0:07:00
 0:00:14
0:00:14
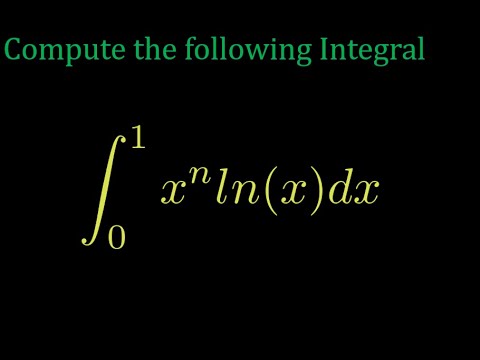 0:03:19
0:03:19
 0:00:35
0:00:35
 0:09:05
0:09:05
 0:00:32
0:00:32
 0:24:48
0:24:48
 0:00:34
0:00:34
 0:02:47
0:02:47
 0:06:36
0:06:36